Muskan Shafi Education Content Expert
Education Content Expert
Percentage Formula is a mathematical formula used to find the amount or share of a quantity in terms of one hundred. The term “Percentage” was coined from the Latin word “Per Centum” meaning “by the hundred”.
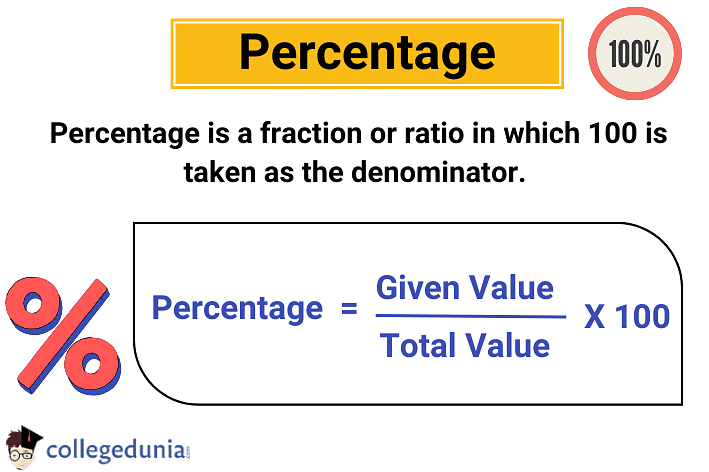
- Percentage is a fraction or ratio in which 100 is taken as the denominator.
- It is denoted by the symbol “%” or percent.
- It is expressed as the relation between part and whole where the value of the whole is always 100.
Percentage of a number is calculated by dividing the given value by the total value and then multiplying the resultant by 100. Percentage Formula is given as
| Percentage = (Given Value/Total Value) × 100 |
Read More: NCERT Solutions for Class 8 Maths Comparing Quantities
Key Terms: Percentage Formula, Percentage, Hundred, Fraction, Ratio, Denominator, Percentage Increase, Percentage Decrease
What is Percentage?
[Click Here for Sample Questions]
Percentage is a ratio that is expressed as a fraction of 100 in Mathematics. The term ‘Per Cent’ refers to ‘Per 100’.
- Percentage is a fraction with the denominator as 100.
- It is denoted by the sign “%” or simply percent or pct.
- Percentage of a number is calculated by dividing the number by the whole and multiplying it by 100.
- It has no dimension and is thus referred to as a dimensionless number.
- Percantage can also be expressed in terms of decimals like 0.8%, 0.55%, etc.
Percentage
Percentage Examples
Examples of Percentages include:
- 10% = 10/100 = 1/10 or 0.1
- 25% = 25/100 = 1/4 or 0.25
- 50% = 50/100 = 1/2 or 0.5
- 40% = 40/100 = 2/5 or 0.4
The video below explains this:
Percentage Error Formula Detailed Video Explanation:
Read More:
Percentage Formula
[Click Here for Sample Questions]
Percentage Formula is used in order to find the amount or share of a whole in terms of 100. It helps to represent a number as a fraction of 100.
Percentage Formula is given as:
| Percentage = (Given Value/Total Value) x 100 |
Percentage Formula is used in a wide variety of applications such as discounts, bank interest rates, rates of inflation, and in statistics.
Solved ExampleExample: There are 40 students in a class out of which 10 are boys. Find the percentage of boys in the class. Solution: Given that,
Using the Percentage Formula, Percentage = (Given Value ⁄ Total Value) × 100 Percentage of Boys = 10/40 × 100 = 25%. Thus, the percentage of boys in the class is 25%. |
How to Calculate Percentage?
[Click Here for Sample Questions]
Percentage refers to the calculation of the share out of the whole, in terms of 100. Percentage can be calculated in two ways as follows:
- By Changing Denominator of Fraction to 100
- By Using Unitary Method
By Changing Denominator of Fraction to 100
In this method, the equivalent fraction of the given fraction is calculated such that the resultant denominator is 100. Then, the numerator obtained is itself the percentage.
Example: What will be the percentage of 4/25?
4/25 = 4/25 × 4/4 = 16/100 = 16%
By Using Unitary Method
In the unitary method, the fraction is multiplied by 100 to get the percentage.
Example: The percentage for the fraction 4/25 will be as follows:
= 4/25 × 100 = 400/25 = 16%
The first method for calculating the percentage is not used when the denominator is not a factor of 100 and thus the unitary method is applicable in that case.
Read More: Comparing Quantities MCQs
Percentage Difference Formula
[Click Here for Sample Questions]
Percentage Difference Formula is used to calculate the difference between the percentages of two values. It is calculated when the difference between two values is divided by the average of the same values. It is used to calculate the change in the value over a given period.
Percentage Difference Formula is given as:
| \( Percentage \, Difference = \frac{\left|N_{1}-N_{2}\right|}{\left[\frac{\left(N_{1}+N_{2}\right)}{2}\right]} \times 100\) |
Percentage Difference is expressed as a ratio and is a unitless number.
Solved ExampleExample: Find the percentage difference between 20 and 30. Solution: The two given values are 20 and 30. Using the Percentage Difference Formula, \( Percentage \, Difference = \frac{\left|N_{1}-N_{2}\right|}{\left[\frac{\left(N_{1}+N_{2}\right)}{2}\right]} \times 100\) Substituting the values, we get \(\begin{array}{l}Percentage~Difference = \frac{\left|20-30\right|}{\left[\frac{\left(20+30\right)}{2}\right]} \times 100\end{array}\) = |-10|/(50/2) x 100 = |-10|/25 x 100 = 40 Thus, the percentage difference between 20 and 30 is 40%. |
Percentage Change
[Click Here for Sample Questions]
Percentage Change is defined as the change in the value of a quantity in terms of percentage over a period of time. There are two cases of Percentage change:
- Percentage Increase
- Percentage Decrease
Percentage Increase
Percentage Increase is the change in the percentage when it is increased over a period of time. Percentage increase is calculated using the given formula:
| Percentage Increase = (Increased Value – Original Value)/Original Value x 100 |
Solved ExampleExample: The cost of a makeup item increased from Rs.100 to Rs. 150. Find the percentage increase in the price. Solution: Given that,
Using the Percentage Increase Formula, Percentage Increase = (Increased Value – Original Value)/Original Value x 100 Percentage Increase = (150 - 100) / 100 × 100 = 50% |
Percentage Decrease
Percentage Decrease is defined as the change in percentage when it is decreased over a period of time. Percentage decrease is calculated using the given formula:
| Percentage Decrease = (Original Value - Decreased Value)/Original Value x 100 |
Solved ExampleExample: The price of chocolate decreased from Rs.127 to Rs. 103. What will be the percentage decrease? Solution: Given that,
Using the Percentage Decrease Formula, Percentage Decrease = (Original Value – Decreased Value)/Original Value x 100 Percentage Decrease = (127 - 103) / 127 × 100 = 18.9% |
Percentage Chart
[Click Here for Sample Questions]
Percentage Chart is a tabular presentation of various fractions values in form of percentages for easier and faster calculations. The percentage chart is given as follows:
| Fractions | Percentage |
|---|---|
| 1/2 | 50% |
| 1/3 | 33.33% |
| 1/4 | 25% |
| 1/5 | 20% |
| 1/6 | 16.66% |
| 1/7 | 14.28% |
| 1/8 | 12.5% |
| 1/9 | 11.11% |
| 1/10 | 10% |
| 1/11 | 9.09% |
| 1/12 | 8.33% |
| 1/13 | 7.69% |
| 1/14 | 7.14% |
| 1/15 | 6.66% |
Check More:
Things to Remember
- Percentage is a number or ratio that is expressed as a fraction with a denominator of 100.
- Percentage Formula = (Given Value/Total value) × 100.
- It can be calculated using two ways mainly, either by Changing the Denominator to 100 or by Unitary Method.
- Percentage Difference Formula helps to find the difference between the percentages of two values.
- Percentage Difference Formula is \( Percentage \, Difference = \frac{\left|N_{1}-N_{2}\right|}{\left[\frac{\left(N_{1}+N_{2}\right)}{2}\right]} \times 100\).
- Percentage Increase is calculated using the formula: (Increased Value – Original Value)/Original Value x 100.
- Percentage Decrease is calculated using the formula: (Original Value – Decreased Value)/Original Value x 100.
For Latest Updates on Upcoming Board Exams, Click Here: https://t.me/class_10_12_board_updates
Sample Questions
Ques. What is 10% of 45? (2 Marks)
Ans. The given number is 45. The 10% of 45 needs to be calculated.
Thus,
10% of 45 = (10/100) x 45
= 450/100
= 4.5
Therefore, 10% of 45 is 4.5.
Ques. There are 150 students in a class. Out of them, 75 are girls. Find the percentage of girls in the class. (3 Marks)
Ans. Given that,
- Total Number of Students = 150
- Number of Boys = 75
Using the Percentage Formula,
Percentage = (Given Value ⁄ Total Value) × 100
Percentage of Girls = (75/150) × 100 = (7500/150) = 50%
Thus, the percentage of girls in the class is 50%.
Ques. Find out the percentage difference between two values of 20 and 4. (3 Marks)
Ans. The values are given as 20 and 30.
Using the Percentage Difference Formula,
\( Percentage \, Difference = \frac{\left|N_{1}-N_{2}\right|}{\left[\frac{\left(N_{1}+N_{2}\right)}{2}\right]} \times 100\)
Now, substitute the values,
\(\begin{array}{l}Percentage~Difference = \frac{\left|20-4\right|}{\left[\frac{\left(20+4\right)}{2}\right]} \times 100\end{array}\)
= |16|/(12) x 100 = 4/3 × 100
= 1.33 × 100 = 133.33%
Thus, the percentage difference between 20 and 4 is 133.33%.
Ques. What is the percentage change in the rent of the house if in the month of January, it was Rs. 20,000 and in the month of March, it is Rs. 15,000? (3 Marks)
Ans. It can be clearly seen that there is a percentage decrease in the rent.
Decreased Value = 20,000 – 15, 000 = 5, 000
Using the Percentage Decrease Formula,
Percentage Decrease = (Original Value – Decreased Value)/Original Value x 100
= (5000/20,000) x 100
= (1/4) x 100 = 25%
Hence, there is a 25% decrease in the rent.
Ques. What is 50% of 30? (1 Mark)
Ans. The given number is 30. The 50% of 30 needs to be calculated.
Thus,
50% of 30 = (50/100) x 30
= 1500/100
= 15
Therefore, 50% of 30 is 15.
Ques. 50 % of some numbers is 360. What will be 99 % of the same number? (3 Marks)
Ans. Let the number be y. It is given that the 50% of the number is 360.
Thus,
50/100 × y = 360
y = 720
Now, 99% of 720 =?
99% of 720 = 99/100 × 720 = 712.80
Thus, 99% of 720 is 712.80.
Ques. Find 20% of 40? (2 Marks)
Ans. The given number is 40. The 20% of 40 needs to be calculated.
Thus,
20% of 40 = (20/100) x 40
= 800/100 = 8
Therefore, 20% of 40 is 8.
Ques. What is 15% of 60 equal to? (2 Marks)
Ans. The given number is 60. The 15% of 60 needs to be calculated.
Thus,
15% of 60 = (15/100) x 60
= 900/100 = 9
Therefore, 15% of 60 is 9.
Ques. There are 120 people present in an examination hall. The number of men is 50 and the number of women is 70 in the examination hall. Calculate the percentage of women present in the examination hall. (3 Marks)
Ans. Given that,
- Total Number of People in Hall = 120
- Number of Women = 70
Using the Percentage Formula,
Percentage = (Given Value ⁄ Total Value) × 100
Percentage of Women = (70/120) x 100
= 8400/100 = 84%
Thus, the percentage of women in the examination hall is 84%.
Ques. What is meant by Percentage? (2 Marks)
Ans. Percentage is a number or a ratio, which is expressed as a fraction over 100. The symbol of percentage is denoted by this sign %. Percentage formula is given as:
Percentage = (Given Value ⁄ Total Value) × 100
Ques. How to convert a Percentage to a Decimal? (1 Mark)
Ans. To convert a percentage to a decimal, remove the symbol of percentage and divide it by 100
Example: 25.70% = 25.7/100 = 0.257.
Ques. How to convert a Decimal to a Percentage? (1 Mark)
Ans. To convert a decimal to a percentage, multiply it by 100, and add the sign of percentage.
Example: 0.257 = 0.257 × 100 = 25.7%
Check-Out:





Comments