Interest Formula: Simple and Compound Interest, Differences

Interest formulas constitute simple and compound interests formulas. The profit obtained on lending a quantity of money is known as interest. It is always calculated using a specific rate of interest for a specific time period. A person, for example, borrows Rs 1000 from a money lender and promises to repay it in two years. Moneylender demands a 10 percent annual profit, which must be added to the total at the conclusion of two years. We all know that 10% of 1000 is 100. This means that a moneylender requires an extra Rs 100 in profit each year to lend money. Interest is the term for the moneylender's additional profit.

Also Read: Arithmetic Progression

Key Terms: Interest, Simple Interest, Compound Interest, SI, CI, Principal, amount, profit, Formula

---

Simple Interest

[Click Here for Sample Questions]

Simple Interest, abbreviated as S.I., is a method for calculating the amount of interest on a given principal amount of money. Simple interest (SI) is a type of interest calculated on the amount borrowed or invested for the entire term of the loan, without taking into account any other elements such as previous interest (paid or charged) or other financial considerations. 

Simple interest is commonly applied to financial company-administered short-term loans with terms of one year or less. The same can be said for money that has been invested for a relatively short length of time. The simple interest rate is a percentage that is usually stated as a ratio.

Before delving more into the concept of simple interest, it's important to grasp what a loan is. A loan is a sum of money borrowed from a bank or other financial institution to meet one's requirements. Home loans, vehicle loans, student loans, and personal loans are all instances of loans. A loan amount must be returned to the authorities on time, along with an additional amount, which is usually the interest paid on the loan.

---

Simple Interest formula

[Click Here for Sample Questions]

If we know the principal amount, the rate of interest, and the time period, we can use the simple interest formula to calculate the interest amount.

The interest rate is calculated using a basic interest formula, 

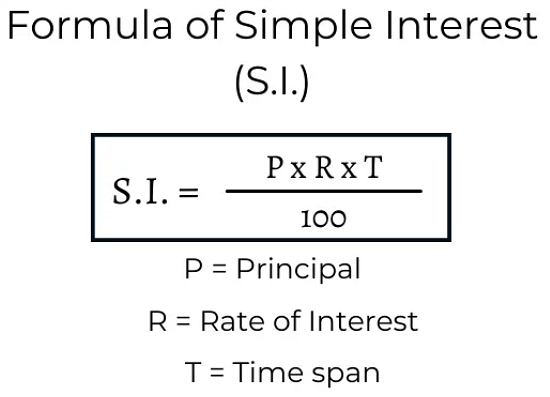
SI = (P × R ×T) / 100

In which,

SI = simple interest

P = principal amount or the original amount being borrowed

R = rate of interest (in percentage)

T = time period (in years)

For the purpose of computing the total amount, apply the following formula:

Amount (A) = Principal (P) + Interest (I)

In which,

Amount (A) = total amount of money repaid at the end of the time period for which it was borrowed

Simple Interest formula

---

S.I. Formula for Months

[Click Here for Sample Questions]

The formula for calculating annual interest rates is well-known. Let's look at the formula for calculating the monthly interest rate. If P is the principal amount, R is the yearly interest rate, and n is the time period (in months), the simple interest formula is as follows:

Simple Interest for ‘n’ months = (P × n × R)/(12 ×100)

---

Example of Simple Interest

[Click Here for Sample Questions]

Anurag's father took out a Rs1,000 loan from the bank, at a 5% interest rate. What would the simple interest rate be if you borrowed the money for a year? Calculate the simple interest if the money is borrowed for 2 years, 3 years, and 10 years, respectively.

Solution: Principal Amount = Rs1,000

Rate of Interest = 5%

Time for which the money is borrowed = T = 1 year

SI = (P × R ×T) / 100

For 1 Year = S.I = (1000 ×5 × 1)/100 = 50

For 2 Years = S.I = (1000 × 5 × 2)/100 = 100

For 3 Years = S.I = (1000 ×5 × 3)/100 = 150

For 10 Years = S.I = (1000 × 5 × 10)/100 = 500

We can now data table for the above question by telling the amount that will be repaid when the time period has passed.

After 1 Year = A= 1000 + 50 = 1050

After 2 Years = A= 1000 + 100 = 1100

After 3 Years = A = 1000 + 150 = 1150

After 10 Years = A = 1000 + 500 = 1500

---

Compound Interest

[Click Here for Sample Questions]

Compound interest is calculated on the principal as well as the interest that has accumulated during the preceding tenure. As a result, compound interest (CI) is frequently referred to as "interest on interest". It is crucial in establishing the amount of interest charged on a loan or investment. Compound interest works by multiplying the amount of money in your bank account. In fact, compound interest is a fantastic thing to have because it isn't just basic interest on a specific quantity of money. 

Compound interest is interest earned on both the principal (original amount) and previously earned interest. It also continues to grow year after year. Compound interest is a new method of calculating interest that is used in all financial and business transactions worldwide. When we look at the compound interest values accumulated over successive time periods, we can see how powerful compounding is. It's like reinvesting an investment's interest income to make the money grow quicker over time! Compound interest is exactly what it does to money. Banks and other financial institutions determine the amount solely on the basis of compound interest.

Also read: Difference Between Relation and Function

Compound Interest Formula

After determining the entire amount over a period of time, based on the rate of interest and the beginning principal, compound interest is determined. The following formula is used to calculate the amount for an initial principal of P, an annual rate of interest of r, a time period of t in years, and the frequency of the number of times the interest is compounded annually, n.

Compound Interest Formula

The total amount at the conclusion of the time period is calculated using the above method, which includes compounded interest and principal. By subtracting the principal from this amount, we can calculate compound interest. The following is the formula for computing compound interest.

In the above formula,

• P is the principal amount
• r is the rate of interest(decimal)
• n is frequency or no. of times the interest is compounded annually
• t is the overall tenure.

It should be noted that the above-mentioned formula is the general formula for compounding the principal n times each year. If the given principal is compounded annually, the amount at a percent rate of interest, r, after the time period is given as:

A = P(1 + r/100)t , and C.I. would be: P(1 + r/100)t – P

---

C.I. Formula for Various Time Periods

[Click Here for Sample Questions]

Compound interest for a given principal can be calculated using several formulas for various time periods.

Compound Interest Formulated for Half Yearly

In the case of compound interest, the rate of interest fluctuates depending on the computation time. When the principal is compounded semi-annually or half-yearly, the compound interest formula is as follows:

Compound Interest Formulated for Half Yearly

In this case, compound interest is calculated over a half-yearly period, thus the rate of interest, r, is divided by 2 and the time period is doubled. When the principal is compounded semi-annually or half-yearly.

---

Compound Interest Formulated Quarterly

[Click Here for Sample Questions]

If the interest is calculated quarterly, the interest is calculated every three months, and the amount is compounded four times every year. When the principal is compounded quarterly, the following formula is used to determine compound interest:

Compound Interest Formulated Quarterly

In this case, compound interest is calculated for a quarterly time period, thus the rate of interest, r, is divided by four and the time period is quadrupled. When the principal is compounded quarterly.

---

Example of Compound Interest

[Click Here for Sample Questions]

Calculate the compound interest on Rs 15,000 over 2 years at a rate of 10% per annum compounded annually.

Solution: Principal amount for first year = Rs 15,000

Interest for first year = 10% of P = Rs 1,500

Amount at the end of first year = Rs 16,500

Principal amount for the second year = Rs 16,500

Interest for the second year = 10% of 16500 = Rs 1,650

The amount at the end of the second year = Rs 18,150

---

Differences Between Simple Interest and Compound interest

[Click Here for Sample Questions]

Compound interest is more difficult to compute than simple interest due to some fundamental distinctions between the two. Here are the key differences: 

Simple Interest

1. Every time, simple interest is calculated on the original principal amount.
2. S.I. = P R T is the formula that is used to compute it.
3. It is the same for each year based on a fixed principal.

Compound Interest

1. The accumulated amount of principal and interest is used to compute compound interest.
2. The following formula is used to compute it: C.I.= P × (1+r)t – P
3. It varies depending on the time period because it is based on the amount rather than the principal.

Also Read:

---

Things to Remember

• S.I. (Simple Interest) is a way of determining the amount of interest on a given principal amount of money. Simple interest (SI) is a sort of interest computed on the amount borrowed or invested for the entire length of the loan, with no other factors such as past interest (paid or charged) or other financial considerations taken into account.
• A loan is a sum of money borrowed from a bank or other financial institution to meet one's requirements. Home loans, vehicle loans, student loans, and personal loans are all instances of loans.
• The interest rate is calculated using a basic interest formula,

SI = (P × R ×T) / 100

• Compound interest is calculated on the principal as well as the interest that has accumulated during the preceding tenure. As a result, compound interest (CI) is frequently referred to as "interest on interest". Compound interest is interest earned on both the principal (original amount) and previously earned interest.
• The following formula is used to calculate the amount for an initial principal of P, an annual rate of interest of r, a time period of t in years, and the frequency of the number of times the interest is compounded annually, n.