
Content Writer
A quadrilateral is a closed-shaped polygon that has four straight sides. The sum of angles of all the sides is always equal to 360°. It is a two-dimensional shape. If a shape does not have four sides, then it’s not a quadrilateral.
- Quadrilaterals are shapes that are often divided into many different types.
- It is also popular with the name tetragon.
- Quadrilaterals are formed by connecting non-collinear points.
- It is derived from a Latin word named Quadra, equivalent to four, and latus, equivalent to sides.
- These shapes are different from the others by their properties and their names.
- In our daily lives, we see a lot of quadrilaterals around us.
- These shapes are squares, rectangles, parallelograms, rhombus, etc.
Read More: Isosceles Triangle Theorems
Key Terms: Quadrilaterals, Types of Quadrilaterals, Square, Rectangle, Rhombus, Trapezoid, Parallelogram, Non-Collinear Points, Sides, Angles
What are Quadrilaterals?
[Click Here for Sample Questions]
Quadrilaterals are two-dimensional shapes with four sides, four angles & four vertices. All the sides of a quadrilaterals can be equal or unequal depending on their shape and properties.
- All the sides of a quadrilateral have the sum of all sides equivalent to 360°.
- Square, rectangle, rhombus, trapezoid (trapezium) & parallelograms are the most popular types of quadrilaterals.
- Joining the vertices of the quadrilateral will determine the diagonals of the figure.
- Cards, chess boards and traffic signals are some examples of the figure in real life.
Read More: Cyclic Quadrilateral
The summary about what are quadrilaterals are provided in the table below:
| Category | Data |
|---|---|
| Quadrilaterals | Two-dimensional plane figure with four edges and four vertices |
| Number of Sides of | 4 |
| Number of Vertices of Quadrilaterals | 4 |
| Number of Diagonals of Quadrilaterals | 2 |
| Sum of all Interior Angles of Quadrilaterals | 360 degrees |
| Sum of all Exterior Angles of Quadrilaterals | 360 degrees |
Solved Examples of QuadrilateralsGiven below are some of the examples of Quadrilaterals Example 1: The sides of a square are 7 m. Find out the Area of the square. Ans: Given, sides=7 m Sides of the square = 7 m Area of a square = side2 = 72 = 7×7 = 49 m2 Thus, the area of the square is 49 m2. Read More: Area of Parallelogram Example 2: The length of a rectangle farm is 50 yards & the width is 30 yards. Find out the area of the rectangle. Ans: Given, Length= 50 yd, width= 30 yd . Area of the rectangular farm = length × width = 50 ×30 = 1500 Square yards So the area of the trapezoid farm is 1500 Square yards. |
Read More: Quadrilateral formulas
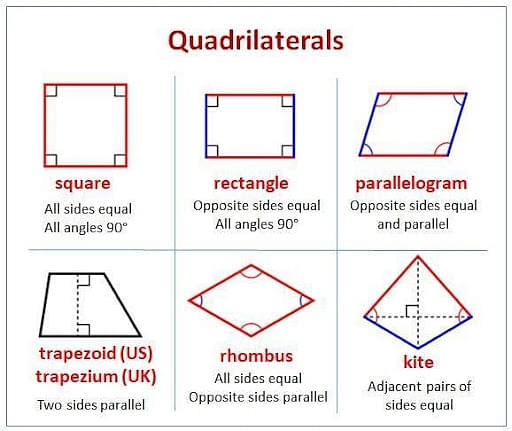
Types of Quadrilaterals
[Click Here for Sample Questions]
There are different types of quadrilaterals that can be divided into the following types: Square, Rectangle, Trapezoid, parallelogram, rhombus & kite. The detail discuss about each of the category is provided below:
Square
A square has four equal sides i.e., all the sides are of the same length & width. A square is also called a right angle due to its accurate measurement. All the sides of a square are 90°. Some examples of squares are chessboard, stamps, bread, clock & cheese slices, etc.

Read More: Difference between Square and Rectangle
Solved Examples of SquareGiven below are some of the examples of square Example 1: The sides of a square are 10 m. Find out the Area of the square. Ans: Given, sides = 10 m Sides of the square = 10 m Area of a square = side2 = 102 = 10 x 10 = 100 m2 Thus, the area of the square is 100 m2 |
Read More: Square Formula
Rectangle
A rectangle is different from a square. The opposite sides of a rectangle are equal in length. Some examples of rectangles are laptops, books, cellphones, beds, etc.

Read More: Mensuration
Solved Examples of RectangleGiven below are some of the examples of Rectangle Example 1: The length of a rectangle farm is 70 yards & the width is 30 yards. Find out the area of the rectangle. Ans: Given, Length= 70 yd, width= 30 yd . Area of the rectangular farm = length × width = 70 ×30 = 2100 Square yards So the area of the trapezoid farm is 2100 Square yards. |
Read More: Area Formula
Rhombus
A rhombus is also quadrilateral. All the opposite sides are parallel to each other whereas opposite angles are the same in measurement. The sum of two adjacent angles of a rhombus is always 180°. Some examples of a rhombus are Diamond, buildings, gardening tools, spare blades, mats, etc.

Read More: Area of Rhombus
Solved Examples of RhombusGiven below are some of the examples of Rhombus Example 1: David has drawn a rhombus where the lengths of the two diagonals d1 and d2 are 15 units and 16 units, respectively. He asks his sister Linda to help him find the area. Can you help Linda find the answer? Ans: Given: Diagonal, d1 = 15 units, and d2 = 16 units A = (d1 × d2)/2 A = (15×16)/2 A = 120 sq. units The area of the rhombus = 120 sq. units. |
Read More: Difference between Square and Rhombus
Trapezoids
In a trapezoid the opposite sides are usually parallel. The base of a trapezoid parallel to the opposite side. A trapezoid is also called a trapezium. Some examples of a trapezoid are a bucket, glass, lamp, guitar, ring, etc.

Read More: Area of a Trapezoid Formula
Solved Examples of TrapezoidGiven below are some of the examples of Trapezoid Example 1: A trapezoid has four sides measuring 10 units, 17 units, 15 units, and 19 units. What is the perimeter of the trapezoid? Ans: The perimeter of a trapezoid is given by the sum of all the sides. Perimeter = 10 + 17 + 15 + 19 = 61 units Therefore, the perimeter of the given trapezoid is 61 units. |
Read More: Perimeter of a Trapezoid
Properties of Quadrilaterals
[Click Here for Sample Questions]
Properties for each of the types of quadrilaterals are as follows:
Square
Some of the properties about square are as follows:
- It has four sides of equal length.
- The measure of each angle is 90°.
- Diagonals of a square perpendicularly bisect each other.
Rectangle
Some of the properties about rectangle are as follows:
- The opposite sides in a rectangle are equal in length.
- All the angles measure 90°.
- The diagonals perpendicularly bisect each other.
Read More:
Rhombus
Some of the properties about rhombus are as follows:
- The four sides of a rhombus are equal.
- The opposite sides are parallel to each other.
- The opposite angles in a rhombus measure the same.
- The sum of two adjacent angles of a rhombus is 180°.
Read More: Angle Between a Line and a Plane
Trapezoid
Some of the properties about trapezoid are as follows:
- It is made up of two parallel & two non-parallel sides.
- A trapezoid is of two types: scalene trapezium & isosceles trapezium.
- In scalene trapezium, non-parallel sides are of different lengths, whereas in isosceles non-parallel sides are of the same length.

Read More: Intersecting & Non-Intersecting Lines
Important Formulas for Quadrilaterals
[Click Here for Sample Questions]
The formulas relating to quadrilaterals can be divided into two parts:
- Formula of Area
- Formula of perimeter
It can be clearly understood with the help of the following table:
| Type of Quadrilateral | Area | Perimeter |
|---|---|---|
| Square | side2 | 4×side |
| Rectangle | length× width | 2(Length+Breadth |
| Rhombus | (1/2) × Diagonal 1 × Diagonal 2 | P=4×side |
| Trapezoid | 12×(sum of the lengths of parallel side)×height | P=a+b+c+d |
Read More: Perimeter of a Triangle
Things to Remember
- Quadrilaterals are four sides figures where sum of all interior angles is 360°.
- Diagonals are formed by joining two non-collinear points
- The sum of adjacent angles of a quadrilateral is 180°.
- Square, Rhombus, Rectangle and Trapezoid are some types of quadrilaterals.
- A quadrilateral can form a regular or irregular shape.
- A square and a rhombus have four equal sides.
- The opposite sides in a rectangle are equal.
- The opposite sides of a trapezium are parallel to each other.
Read More:
Sample Questions
Ques: Find out the area of a trapezoidal park. If it has one base measuring 150 m & the other base is 75 m in length & the height of the park 55 m. (4 marks)
Ans: One base of the park= 150 m
The second base of the park= 75 m
Height of the park= 55 m
The Quadrilateral area formula of a trapezoid is ½ ×(sum of the length of parallel sides)×height
Area of a trapezoid= ½ × (sum of the lengths)×heights
= ½ × 225×50
=112.5×50
= 6187.5 m2
Therefore the area of the trapezoidal park is 6187.5 m2.
Ques: Find out the area of these squares. If the sides of the square are given: (5 marks)
(A) Sides= 5 m
(B) Sides= 6 m
(C) Sides= 8 m
(D) Sides= 10 m
Ans: (A)- Given= 5 m
Area of the square= (side)2
= (5)2
parallel = 5×5
= 25 m
The area of the square is 25 m.
(B)- Given= 6 m
Area of the square = (side)2
= (6)2
= 6×6
= 36 m
The area of the square is 36 m.
(C)- Given= 8 m
Area of the square= (side)2
= (8)2
= 8×8
= 64 m
The area of the square is 64 m.
(D)- Given= 10 m
Area of the square= (side)2
= (10)2
= 10×10
= 100 m
The area of the square is 100 m.
Ques: Find out the area of a rectangular-shaped room of length 150 yards & the width of the rectangle is 250 yards. (2 marks)
Ans: Given, length= of 150 yards
Width= 250 yards
Area of the rectangle= length×width
=150×250
= 37500 yards
Therefore the area of the rectangular-shaped room is 37500 yards.
Ques: What is a quadrilateral? What are its properties. (2 marks)
Ans: A quadrilateral is a polygon that has four sides. All the sides of a quadrilateral are equal and the sum of their angles is 360°. There are so many types of quadrilaterals like rectangle, square, rhombus, parallelogram & trapezoid.
Properties of a quadrilateral:
- A quadrilateral has 4 sides.
- It has 4 vertices.
- The sum total of all the angles in a quadrilateral is 360°.
Ques: If the side of a square is 12 m. Find out the perimeter of the square. (2 marks)
Ans: Given, the side of the square =12 m
The quadrilateral formula of perimeter = 4× side
=4×12
= 48 m.
Therefore the area of the square is 48 m.
Ques: Find out the area of a rhombus. If its diagonal lengths are 8 & 6 respectively. (2 marks)
Ans: Given, Diagonal length = 8
Diagonal length = 6
Area of the rhombus = ½ × Diagonal 1 × Diagonal 2
. = ½×8×6
. = ½× 48
Rhombus = 24 Square units
Therefore the area of the rhombus is 24 Square units.
Ques: A trapezoid has four sides measuring 11 units, 27 units, 35 units, and 29 units. What is the perimeter of the trapezoid. (2 marks)
Ans: The perimeter of a trapezoid is given by the sum of all the sides.
Perimeter = 11 + 27 + 35 + 29
= 102 units
Therefore, the perimeter of the given trapezoid is 102 units.
Ques: Find out the area of a rhombus. If its diagonal lengths are 10 & 6 respectively. (2 marks)
Ans: Given, Diagonal length = 8
Diagonal length = 6
Area of the rhombus = ½ × Diagonal 1 × Diagonal 2
. = ½× 10 × 6
. = ½× 60
Rhombus = 30 Square units
Ques: Alisha has a rectangular photo frame that is 17 inches long and 19 inches wide. Can you help Alisha find its area?
Ans: We know the formula to calculate the area of a rectangle.
Area of a Rectangle = (Length × Width).
Thus, the area of the rectangular frame = 17 × 19 = 323 square inches
Therefore, the area of the photo frame = 323 square inches
Ques: If the side of a square is 22 m. Find out the perimeter of the square. (2 marks)
Ans: Given, the side of the square =22 m
The quadrilateral formula of perimeter = 4× side
=4 ×22
= 88 m.
Therefore the area of the square is 88 m.
Ques: Find out the area of a trapezoidal park. If it has one base measuring 150 m & the other base is 65 m in length & the height of the park 50 m. (4 marks)
Ans: one base of the park= 150 m
The second base of the park= 65 m
Height of the park= 50 m
The Quadrilateral area formula of a trapezoid is ½ ×(sum of the length of parallel sides)×height
Area of a trapezoid= ½ × (sum of the lengths)×heights
= ½ × 215×50
=215 x 25
= 4300 m2
Therefore the area of the trapezoidal park is 4300 m2.
Ques: The length of a rectangle farm is 50 yards & the width is 30 yards. Find out the area of the rectangle. (2 marks)
Ans: Given, Length= 150 yd, width= 20 yd
. Area of the rectangular farm = length × width
= 150 ×20
= 3000 Square yards
So the area of the trapezoid farm is 3000 Square yards.
Ques: Find out the area of these squares. If the sides of the square are given: (5 marks)
(A) Sides= 15 m
(B) Sides= 16 m
(C) Sides= 18 m
(D) Sides= 21 m
Ans: (A)- Given= 15 m
Area of the square= (side)2
= (15)2
parallel = 15× 5
= 225 m
The area of the square is 225 m.
(B)- Given= 16 m
Area of the square = (side)2
= (16)2
= 16×16
= 256 m
The area of the square is 256 m.
(C)- Given= 18 m
Area of the square= (side)2
= (18)2
= 18×18
= 324 m
The area of the square is 324 m.
(D)- Given= 21 m
Area of the square= (side)2
= (21)2
= 21×21
= 441 m
The area of the square is 441 m.
Ques: Amit has drawn a rhombus where the lengths of the two diagonals d1 and d2 are 40 units and 60 units, respectively. He asks his sister Linda to help him find the area. Can you help Linda find the answer. (3 marks)
Ans: Given:
Diagonal, d1 = 40 units, and d2 = 60 units
A = (d1 × d2)/2
A = (40×60)/2
A = 1200 sq. units
The area of the rhombus = 1200 sq. units.
Ques: Find out the area of a rectangular-shaped room of length 120 yards & the width of the rectangle is 300 yards. (2 marks)
Ans: Given, length= of 120 yards
Width= 300 yards
Area of the rectangle= length×width
=120×300
= 36000 yards
Therefore the area of the rectangular-shaped room is 36000 yards.
Check-Out:





Comments