
Content Curator
Sphere is a circular shaped object which has an absolute three dimensional symmetry. In a three dimensional space, all the points on the surface of the sphere are at equal distance from a fixed point that is known as the centre of the sphere. The line that joins the centre to the boundary is called radius of the sphere. It is usually represented by the letter ‘r’. Diameter of the sphere is the longest straight line that passes through the centre of the sphere, It is twice the length of the radius. It touches the surface at two different points. A sphere is devoid of any vertices or edges. As the sphere is a three-dimensional shape it also has a volume and surface area.
Read More: Experimental Probability
Formulas of a Sphere
There are 4 main formulas for a sphere.
1. Diameter of the Sphere
The diameter of a sphere is defined as the straight line that passes through the center of the sphere and touches two points on either side of its surface. The diameter of a sphere is always twice its radius.
The diameter of the sphere when r is the radius is given by the formula:
D = 2 x r

2. Circumference of a Sphere
The circumference of a sphere can be calculated as 2π times its radius. The Circumference of a sphere and that of a circle is given by the same formula:
C = 2 π r
Here, π is a constant and its value is 3.14 or 22/7.

Also Read: Surface Areas and Volumes
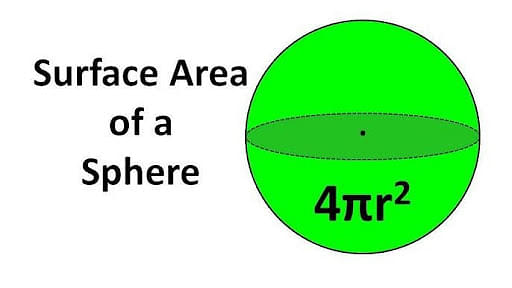
3. Total Surface Area of a Sphere
The total surface area of a sphere is the same as its curved surface area as it is evident that the sphere does not have any lateral surfaces.
The formula obtained for deriving the surface area of a sphere is written mathematically as:
TSA = 4 π r2
In the above equation,
- TSA is the total surface area of a sphere. It can also be simply stated as surface area.
- π is a constant and its value is equal to 3.14 or 22/7
- The value of the radius of the given sphere is represented by ‘r’
4. Volume of the Sphere
The derivation of volume of a sphere is the same as finding the total space available within the surface of the sphere. The unit of volume of a sphere is represented as the (unit)3. Cubic meters or cubic centimeters are known as the metric units of volume. The volume of the sphere is proportional to the radius of the sphere. There are mainly two types of spheres, solid sphere and hollow sphere. Both the types of spheres have different volumes.
The volume of the sphere is derived from the formula given below:
V = 4/3 π r3
In the above equation,
- ‘V’ is the volume of the sphere
- π is a constant and its value is equal to 3.14 or 22/7
- ‘r’ represents the value of the radius of the given sphere

In the case of a hollow sphere, R is represented as the radius of the outer sphere, r is represented as the radius of the inner sphere and V is represented as the volume of the sphere. Now, the volume of the sphere is given by:
Volume of Sphere, V = Volume of Outer Sphere - Volume of Inner Sphere = (4/3)πR3 - (4/3)πr3 = (4/3)π(R3 - r3)

Also Read: Surface Areas and Volumes Revision Notes
Difference between Sphere and Circle
| Sphere | Circle |
|---|---|
| Sphere is a three dimensional shape. | Circle is a two dimensional shape. |
| A sphere extends in three directions i.e. X-axis, Y-axis and Z-axis. | A circle extends in two direction i.e. X-axis and Y-axis. |
| Sphere occupies space and it has volume. | Circle does not have any vlume. |
| Sphere does not have any faces, it has curved surface. | Circle has one flat face. |
| The area of sphere is represented at 4πr2 | The area of circle is represented as πr2 |
Things to Remember
- Sphere is an absolute symmetrical circular shaped object in a three dimensional space.The sphere and circle are not the same. All the points on the surface of the sphere are at equidistant from a fixed point which is called as the center of the sphere. The circle is a two-dimensional geometric shape whereas a sphere is a three-dimensional shape.
- The straight line that connects the center of the sphere to any point on its surface is the radius of the sphere which is generally represented by the letter ‘r’ while the diameter of a sphere is that longest line which passes through the center of the sphere and touches the surface at two different points on the surface.
- By deriving the surface area of a sphere formula, it was found by Archimedes that it was the same as the lateral surface area of a cylinder with the base radius equal to that of the sphere and the height equal to the diameter of the sphere.
- A solid sphere has one radius but a hollow sphere has two radii, and both the radii have two different values, one for the outer sphere and other one for the inner sphere. Thus, the volume of both spheres are different.
Read More:
Solved Examples
Ques 1. Calculate the diameter and the circumference of a sphere whose radius is 7 cm. (3 marks)
Solution: Given,
Radius of the sphere = 7cm
Diameter of the sphere is calculated as:
D = 2 x r
D = 2 x 7
D = 14 cm
Circumference of the sphere is calculated as:
C = 2 x π x r
C = 2 x (22/7) x 7
C = 2 x 22
C = 44 cm
Hence, the diameter of the sphere is 14 cm and circumference of the sphere is 44 cm.
Ques 2. Calculate the diameter, surface area and volume of a sphere of radius 8 cm? (3 marks)
Solution: Given,
r = 8 cm
Diameter of a sphere =2r= 2 × 8=16 cm
Surface area of a sphere
4πr2 = 4 × π × 82 = 4 × π × 64 = 804.24 cm2
Volume of a sphere = 4/3 πr3= 4/3 π. 83= 2144.66 cm3
Thus, the Diameter 16cm, , Surface area is 804.24 cm2 and volume of the given sphere 2144.66 cm3
Read More:
Ques 3. Find the total surface area and the volume of a sphere whose radius is 14 cm. (4 marks)
Solution: Given,
Radius of the sphere = 14 cm
The formula for the deriving surface area of a sphere is given as:
SA = 4 π r2
SA = 4 x (22/7) x (14)2
SA = 4 x (22/7) x 14 x 14
SA = 4 x 22 x 28
SA = 2464 cm2
The volume of a sphere is calculated as:
V = (4/3) π r3
V = (4/3) x (22/7) x (14)3
V = 11494.04 cc
Therefore, the volume and total surface area of a sphere of radius 14 cm are 11494.04 cc and 2464 cm2 respectively.
Ques 4. The volume of a sphere is found to be 729 cc. Calculate its radius. (2 marks)
Solution: Given,
Volume of the sphere = 729 cc
The formula for deriving volume of a sphere is
V = 4/3 π r3
729 = (4/3) (22/7) r3
729 = (88/21) r3
r3 = (729 x 21) / 88
r3 = 173.97
r = 3√173.97
r = 5.58 cm
Hence, 5.58 cm is the radius of the sphere.
Ques 5. Find the volume of the sphere having a radius of 4 inches. (2 marks)
Solution: Given,
r = 4 inches
As we know, the volume of sphere, V = (4/3)πr3
Thus, volume of sphere, V = (4/3)πr3 = ((4/3) × π × 43) in3
V = 268.08 in3
Therefore, 268.08 in3 is the volume of the sphere.
Read More:
Ques 6. The surface area of a spherical object is 379.94 m2. What is the radius of the object? (2 marks)
Solution: Given,
Surface area = 379.94 m2
But, surface area of a sphere = 4πr2
379.94 = 4 x 3.14 x r2
379.94 =12.56r2
r2 = 379.94/12.56
r2 = 30.25
r = √30.25
r = 5.5
Therefore, the radius of the spherical solid is 5.5 m.
Ques 7. The cost of leather is Rs10 per square meter. Find the cost of manufacturing 1000 footballs of radius 0.12 m. (2 marks)
Solutions: Surface area = 4πr2
SA = 4 x 3.14 x 0.12 x 0.12
SA = 0.181 m2
The cost of manufacturing a ball = 0.181 m2 x Rs10 per square meter = Rs1.81
Therefore, the total cost of manufacturing 1000 balls = Rs 1.81 x 1000 = Rs1810
Ques 8. The radius of the Earth is said to be 6,371 km. What is the surface area of the Earth? (2 marks)
Solutions: Since, Earth is a sphere.
Radius : 6371km
SA = 4πr2
SA = 4 x 3.14 x 6,371 x 6,371
Surface area = 5.098 x 108 km2
Also Read:






Comments