
Namrata Das Exams Prep Master
Exams Prep Master
Did you know that the term integer comes from the Latin word integer, which means "whole"? A number that may be expressed as a non-fraction is referred to as an integer. That is, integers are numbers that may be written without a fractional component.
Read Also: Rationalize the Denominator
Integers include numbers such as 1, 4, 9, -7, -66, and so on. Exponents that should be integers, whether positive or negative, are referred to as integer exponents in mathematics. The number of times a number should be multiplied by itself is denoted by the positive integer exponents. Negative exponents must usually be inverted before being multiplied. Let’s learn about the integers exponent rule in detail and discuss some important questions.
| Table of Content |
What are Integers?
The integer is a whole-valued positive or negative number or 0. The integers are generated from the set of counting numbers, 1, 2, 3,... or the operation of subtraction. For instance, when a counting number is subtracted from itself (4 - 4 = 0). The result will be a negative whole number when a larger number is subtracted from a smaller number (2 - 3 = -1). This is the way how every integer can be derived from the counting numbers.

Integers
Also Read: Meaning of Consecutive Numbers
What are Exponents?
Exponents are used to depict how a number is multiplied over and over again. It can be tiresome to write enormous numbers. They take up more space and time in huge mathematical statements. Exponents are used to solve this problem. For example, the number 7*7*7 can be written as 7^3. The exponent, in this case, is '3,' which represents the number of times the value has been multiplied by itself. The number 7 is referred to as the base, and it is the number that is being multiplied. For example, the speed of light is 300000000 m/s. 3*10^8 m/s is a simple way to express this (approximate value). Raising to a power, where the exponent is the power, is a method of utilising exponents.
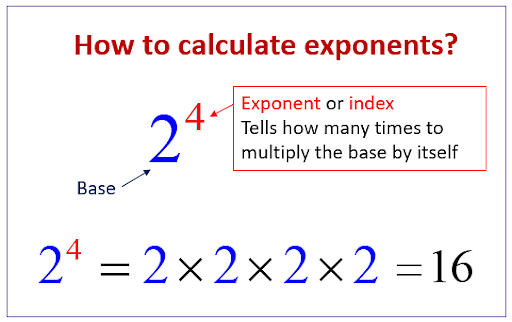
Exponents
Exponents that should be integers are known as integer exponents in mathematics. It can either be a positive or a negative number. The positive integer exponents indicate how many times the base number should be multiplied by itself in this case. On the other hand, negative integer exponents explain flipping the numerator and denominator values before multiplying the number by itself for the number of times specified. Simply put, any integer, positive or negative, can be an exponent. Exponents indicate how many times a base number should be multiplied by itself or by another integer.
The phrase 5*5 can be represented as 5^2, for example. The exponent is 2, yet the integer is both 2 and 5. The exponent 2 indicates that 5 must be multiplied twice with itself in this case. Similarly, 43 stands for 4 × 4 × 4 shows that 4 should be multiplied by itself three times.
Also check:
Integer Exponents Rule
The answer to the question "how to solve integer exponents" is seven major exponents rules. In terms of mathematical operations like addition, multiplication, and division, these rules are all-encompassing.

Integer Exponents Rule
Let's take a closer look at these Integer Exponents rules:
- Product of Powers Rule
When multiplying two bases of the same number, add the exponents while keeping the base number constant.
For example- 32 × 34 = 32+4
= 36
= 3 × 3 × 3 × 3 × 3 × 3
= 729
Read Further: Distance Formula and Derivation of Coordinate Geometry
- Quotient of Powers Rule
When dividing two bases with the same number, subtract the exponents while keeping the base constant.
For example- 46 divided by 42 = 46-2
= 44
= 4 × 4 × 4 × 4
= 256
- Power of a Power Rule
When raising an exponent to a higher exponent, multiply the two exponents while keeping the base constant.
For example- (52)3 = 56
= 5 × 5 × 5 × 5 × 5 × 5
= 15,625
- Power of a Product Rule
Distribute the exponent to each of the bases when two bases are multiplied by the same exponent.
For example- (41 × 51)2 = 42 × 52
= 16 × 25
= 400
Check Important Notes for Empirical Probability
- Power of a Quotient Rule
When you increase power to a quotient, divide it evenly between the denominator and the numerator.
For example- (4/5)2 = 42/52
= 16/25
- Zero Power Rule
Any base that has been raised to the power of zero equals one.
For example- 30 = 1
- Negativity Content Rule
Turn any number into a fraction and then make it reciprocal when it is increased to a negative exponent.
For example- 3-2 = 1/32
The purpose of this rule is to transform negative exponents into positive exponents.
Also check:
How to Solve Integer Exponents?
Solving integer exponents can be a simple assignment if the student understands the fundamentals of the idea. As previously stated, integer exponents are exponents that specify the number of times the base number is to be multiplied with itself or with another number.
The solving procedure is governed by seven rules, all of which have been discussed above. Integer exponent questions can be answered with the help of these rules.
Let us look at the general steps that are involved in solving such questions-
- Step 1. Carefully observe the given question.
- Step 2. Determine which of the seven formulas is appropriate.
- Step 3. Use the chosen formula in the question.
- Step 4. Write the answer clearly.
- Step 5. Recheck the process and the final answer at the end.
The complete process of using one of the formulae, getting the solution, and then re-checking ensures that there are no errors and that they can be fixed if they are made. A method like this would help you get good grades and avoid making dumb blunders. You will also have a better understanding of the question and the steps involved.
Also Check:
Things to Remember
- The term "power" refers to a mathematical phrase that represents repeated multiplications of the same integer. On the other hand, the exponent is the amount that represents the power to which we raise the number.
- According to the zero exponent rule, every base with a zero exponent equals one.
- Multiplication of similar bases and addition of exponents are the first two exponent laws. Second, divide the bases that are the same and subtract the exponent. Finally, when there are two or more exponents and only one base, exponent multiplication is used.
- When multiplying two bases of the same number, add the exponents while keeping the base number constant. For example- 32 × 34 = 32+4
- When dividing two bases with the same number, subtract the exponents while keeping the base constant. For example- 46 divided by 42 = 46-2
- Distribute the exponent to each of the bases when two bases are multiplied by the same exponent. For example- (41 × 51)2 = 42 × 52
Also Read:
Sample Questions
Ques: Find the multiplicative inverse of 9^-4 =1/9^4. (1 mark)
Ans: The multiplicative inverse of 1/9^4 is 9^4.
Ques: Expand the number 12345 in the exponent form. (2 marks)
Ans: The number 12345 can be expressed as:
12345 = 1 × 10000 + 2 × 1000 + 3 × 100 + 4 × 10 + 5 × 1
⇒ 12345=1*10^4 +2*10^3+3*10^2+4*10^1+5*10^0 (Any number raised to the power of zero equals one.)
This approach can also be used to convert decimal numbers.
Ques: Simplify: (3 marks)
3(a4b3)10 × 5(a2b2)3
Ans:
3(a4b3)10 × 5(a2b2)3
= 3 x a4x10 x b3x10 x 5 x a2x3 x b2x3
= 3 x a40 x b30 x 5 x a6 x b6
= 15 x a40+6 x b30+6
= 15 a46 b36
Read Also: Class 10 Polynomials
Ques: Simplify: (2 marks)
(2x-2y3)3
Ans: \((2x^{-2}y)^3\)
\(=2^{3}\times x^{-2\times3}\times y^{3\times3}\)
\(=8\times x^{-6}\times y^{9}\)
\(=8 x^{-6}y^{9}\)
Ques: Simplify: (3 marks)
\(\frac{(4\times 10^{7})(6\times 10^{-5})}{8\times 10^{4}}\)
Ans: \(\frac{(4\times 10^{7})(6\times 10^{-5})}{8\times 10^{4}}\)
\(=\frac{4\times 10^{7}\times 6\times 10^{-5}}{8\times 10^{4}}\)
\(=\frac{4\times 6\times 10^{7-5}}{8\times 10^{4}}\)
\(=\frac{24\times 10^{2}}{8\times 10^{4-2}}\)
\(=\frac{3}{10^{2}}\)
\(=\frac{3}{100}\)
Ques: Simplify: (3 marks)
\(\frac {4ab^{2}(-5ab^{3})}{10a^{2}b^{2}}\)
Ans: \(\frac {4ab^{2}(-5ab^{3})}{10a^{2}b^{2}}\)
\(=\frac {4\times (-5)\times a \times a \times b^{2} \times b^{3}}{10 \times a^{2}\times b^{2}}\)
\(=\frac {-20\times a^{1+1} \times b^{2} \times b^{3}}{10 \times a^{2}\times b^{2}}\)
\(=\frac {-20\times a^{2} \times b^{2} \times b^{3}}{10 \times a^{2}\times b^{2}}\)
\(=-2\times b^{3}\)
\(=-2b^{3}\)
Read Also: Section Formula in Coordinate Geometry
Ques: Simplify: (2 marks)
\((\frac {x^{2}y^{2}}{a^{2}b^{3}})^n\)
Ans: \((\frac {x^{2}y^{2}}{a^{2}b^{3}})^n\)
\(=\frac {x^{2n}\times y^{2n}}{a^{2n}\times b^{3n}}\)
\(=\frac {x^{2n}y^{2n}}{a^{2n}b^{3n}}\)
Ques: If a = 3 and b = -2, find the value of: (2 marks)
ab + ba
Ans: Given, a=3 and b=-2
\(\therefore a^{b} + b^{a} = 3^{-2} + (-2)^{3}\)
\(= \frac{1}{3^{2}} + (-8)\)
\(= \frac{1}{9} - 8\)
\(= \frac{1 - 72}{9}\)
\(= \frac{-71}{9}\)
Mathematics Related Links:






Comments