Real Numbers Formula: Some Basic Properties of Real Numbers

The infinite list of mathematical objects assisting humans to count, or measure inculcates in itself, another fascinating set of numbers called real numbers. Real numbers are numbers that are both rational and irrational numbers. They are represented by the symbol ‘R’. 

These numbers can also be plotted on a number line similar to the other types of numbers that we have studied. Also, real numbers can be used to perform various mathematical operations.

Examples of real numbers include -12, 5.2, 1/34, 0, 1, 3, etc.

Read Also:- Real Numbers

Keyterms: Rational numbers, irrational numbers, mathematical operations, subsets, Natural number, Whole number, Integers

---

Sets of Real Numbers

[Click Here for Sample Questions]

The real numbers contain five subsets, namely-

1. Natural numbers

They contain all the positive counting numbers starting from one and are donated by the symbol ‘N’.

N= {1,2,3, 4…}

Examples- 1,1000, 6745, 99, etc.

2. Whole numbers

They contain all the positive counting numbers starting from zero and are denoted by the symbol ‘W’.

W= {0,1,2,3,4…}

Examples-0, 1, 344, 500, etc.

3. Integers

They contain all the positive and negative numbers along with zero. They are denoted by the symbol ‘Z’.

Z= {-4, -3, -2, -1,0,1,2,3,4…}

Examples- 0, 1, 2, -100, 100, etc.

Integers are further classified as positive and negative integers. Positive integers are represented by Z+ while negative integers are denoted by the symbol Z-

.  

Examples for Z+ =11, 27.6, 99, etc.

Examples for Z-= -11, -27.6, -99, etc.

4. Rational numbers

They can be expressed in the form a/b, where a and b are integers and b is non-zero. They are denoted by the symbol ‘Q’.

Examples -2/5, 1/80, 1, 7/8, etc.

5. Irrational numbers

They cannot be expressed in the form a/b, where a and b are integers and b is non-zero. They are denoted by the symbol ‘P’.

Examples- 3, 5,11, etc.

Real numbers are a subset of another type of number called complex numbers which are a combination of real and imaginary numbers.
The inter-relationship of natural numbers, whole numbers, integers, rational numbers, irrational numbers, and real numbers can be depicted by a Venn diagram as shown below:

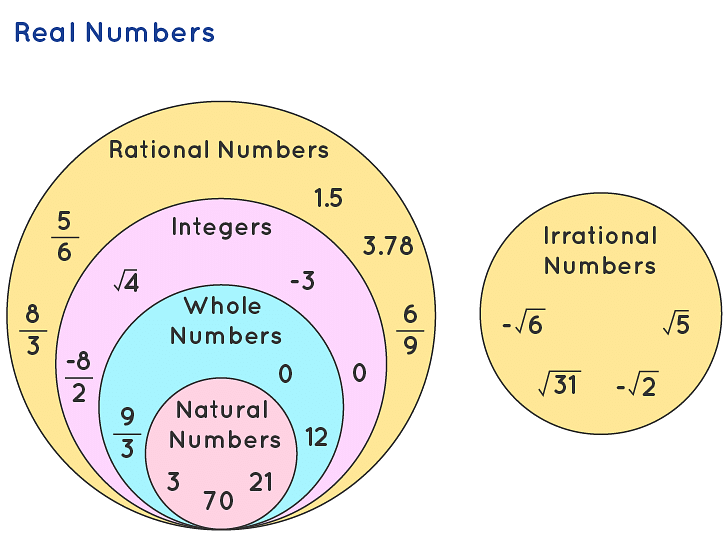
Sets of Real Numbers

---

Basic properties of real numbers

[Click Here for Sample Questions]

Some of the basic properties of real numbers include commutative, distributive, identity, and associative properties. These properties help to ease the calculations to a greater extent and avoid basic human error.

The list of properties is given below:

1. Commutative properties

Let x and y are two real numbers. The commutative properties in addition and multiplication for x and y are:

x+y=y+x=real number

x.y=y.x=real number

Examples are 7+3=3+7=10, which is a real number.

7.3=3.7=21, which is a real number.

2. Associative properties

Let x, y, and z are three real numbers. The associative properties in addition and multiplication for x, y, and z are:

(x+y) +z=x+(y+z) =real number

(xy)z=x(yz) =real number

Examples are (2+3) +4=2+(3+4) =9, which is a real number

(2.3)4=2(3.4) =24, which is a real number

 3. Distributive properties

Let x, y, and z be three real numbers. The distributive properties in addition and multiplication for x, y, and z are:

x(y+z) =xy+xz

An example is 2(3+4) =2.3+2.4=14, which is a real number.

4. Identity properties

Let x is any real number. Identity properties in addition and multiplication for x are:

0 is the additive identity so x+0=0+x=x

1 is the multiplicative identity so x.1=1.x=x

Also Read:

---

Real Numbers Formulas

[Click Here for Sample Questions]

• Theorem 1: Euclid’s Division Lemma

It states that for given positive integers p and r, there exist unique integers x and y such that p=ry+x and 0≤x

Euclid’s division algorithm is based on Euclid’s division lemma.

• Theorem 2: Euclid’s Division Algorithm

It states that to obtain the highest common factor (HCF) of two positive integers, say a and b, such that a>b, the following method has to be adopted:

Step 1: Use Euclid’s division lemma on the two positive integers, a and b. Next, we find the whole numbers x and y such that a=by+x, 0≤x

Step 2: b is the HCF if x is zero. Apply Euclid’s division lemma to b and x if x is non-zero.

Step 3: The process continues till zero is obtained as remainder. The HCF will be the divisor once the process terminates.

Here, one can see that the HCF of a and b is equal to the HCF of b and x.

Example: Show that every positive even integer is of the form 2q and that every positive odd integer is of the form 2q+1, where q is some integer.

Solution: let, the positive integer be a and b=2.

According to Euclid’s division lemma, we can say; a=bq+r

So, a=2q+r such that 0≤r<2.

So, case 1

When r=0

a=2q+0

a=2q

since, 2x integer= even integer

therefore, a=2q represents an even positive integer.

Case 2

When r=1

a=2q+1

so, positive integers= even positive integers + odd positive integers

Therefore, 2q+1 represents an odd positive integer.

Example: Use Euclid’s division algorithm to find HCF of 441, 567, and 693.

Solution: HCF (441,567,693)

The HCF of 441 and 567 is 63 by Euclid’s division algorithm and by step division method.

Now HCF of 63 and 693 is 11.

Since, the remainder is 0, the combined HCF of 441, 567, and 693 is 63.

• Theorem 3: Fundamental Theorem of Arithmetic

It states that every composite number can be given as a product of prime numbers and this factorization is unique other than the order of the prime factors occurrence.

The prime factorization of a natural number is unique, except for the order of the factors.

Theorem 4: for a prime number, say, a. If a divides square of p, where p is a positive integer, then a divides p.

Theorem 5: Let a is a rational number with terminating decimal expansion. So, a is expressed in the fractional form x/y, where x and y are coprime numbers, and the prime factorization of y is in the form 2m5n such that m and n are positive integers.

Theorem 6: let a=x/y is a rational number, and the prime factorization of y is in the form 2m5n such that m and n are positive integers, then a has a terminating decimal expansion.

Theorem 7: Let a=x/y where x and y are co-prime numbers and a is a rational number, such that the prime factorization of y is not in the form 2m5n such that m and n are positive integers, then a has a non-terminating, repeating decimal expansion.

Example: Find the simple form of 368/496

Solution: we find the HCF of 368 and 496 by step division method.

HCF=16

Now, dividing numerator and denominator with the HCF, we get 23/31.

The simplified form is 23/31.

Thus, for every rational number, the decimal expansion can be terminating or non-terminating repeating.

HCF is the highest common factor. It is the greatest number that can divide any two numbers exactly.

LCM is the least common multiple. It is the smallest number that is divisible by any two numbers considered.

Also, for any two positive integers x and y, the product of the HCF of x and y and the LCM of x and y are equal to the product of the two positive integers x and y.

Also Read: Complex Numbers and Quadratic Equations

---