
Collegedunia Team Content Curator
Content Curator
Sin 30 degrees has a value of ½ or 0.5. In radians, Sin 30o is represented as Sin \(\frac{\pi}{6}\). Sine or Sin is one of the most important trigonometric ratios whose values range between -1 to +1. The other important trigonometric ratios are Cos, Tan, Sec, Cosec, Cot. In this article, we will learn about the value of Sin 30o and its derivation.
| Sin 30o = ½ |
Read Here: Applications of Trigonometry
| Table of Content |
Key Takeaways: Sin 30 degrees, Radians, Trigonometric Ratios, Trigonometric Table, Geometric Derivation.
Sin 30 Degrees Value
[Click Here for Sample Questions]
In a right-angled triangle, the sin of the angle is the ratio of the side opposite the angle to the hypotenuse (longest side of triangle).
\(Sin \theta = \frac{Opposite}{Hypotenuse}\)
The angle sin 30° is between 0° and 90° and lies in the First Quadrant and the value for sin 30 degree angle is 0.5.
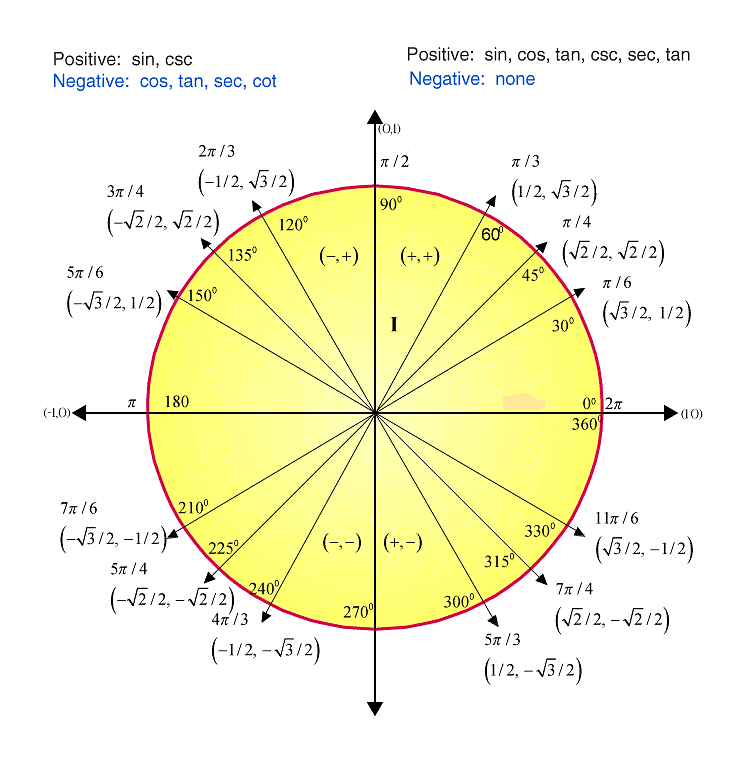
Sin 30 Degrees
Read Here: Introduction to Trigonometry
Geometric Derivation of Sin 30 Degree
[Click Here for Sample Questions]
Consider an equilateral triangle ABC
We know that, All angles in an equilateral triangle are 60 degrees.
Thus , ∠A = ∠B = ∠C = 60o
Now draw a perpendicular line AD from A to BC as shown in the figure below.

Now in Triangle ΔABD = ΔACD
Therefore, BD = DC
Also ΔBAD = ΔCAD (By Corresponding Parts of Congruent Triangles)
Lets assume the length of side of the equilateral triangle AB = BC = AC = 2a.
Then, BD = ½ BC = ½ x 2a = a
In Right Triangle ADB,
AD2 + BD2 = AB2 [By Pythagoras theorem]
AD2 = = AB2- BD2
(2a)2 – a2 = (3a)2
AD2 = 3a2
AD = a√3
Now, In right Triangle ADB,
Sin 30o = BD/AB = a/2a = ½ or 0.5.
Similarly, we can represent Sin 30o by using trigonometric identities as follows.
- Sin (180o – 30o) = Sin 50o
- -Sin (180o + 30o) = -Sin 210o
- Cos (90o - 30o) = Cos 60o
- -Cos (90o + 30o) = -Cos 120o
Check More: Trigonometry Important Formulae
Trigonometric Values Table
[Click Here for Sample Questions]
The important values of trigonometric ratios are given in the table below.
| Angles | 0o 0 | 30o \(\frac{\pi}{6}\) | 45o \(\frac{\pi}{4}\) | 60o \(\frac{\pi}{3}\) | 90o \(\frac{\pi}{2}\) | 180o \(\pi\) |
|---|---|---|---|---|---|---|
| Sin | 0 | ½ | \(\frac{1}{\sqrt{2}}\) | \(\frac{\sqrt{3}}{2}\) | 1 | 0 |
| Cos | 1 | \(\frac{\sqrt{3}}{2}\) | \(\frac{1}{\sqrt{2}}\) | ½ | 0 | -1 |
| Tan | 0 | \(\frac{1}{\sqrt{3}}\) | 1 | \(\sqrt{3}\) | Not Defined | 0 |
| Cosec | Not Defined | 2 | \(\sqrt{2}\) | \(\frac{2}{\sqrt{3}}\) | 1 | Not Defined |
| Sec | 1 | \(\frac{2}{\sqrt{3}}\) | \(\sqrt{2}\) | 2 | Not Defined | -1 |
| Cot | Not Defined | \(\sqrt{3}\) | 1 | \(\frac{1}{\sqrt{3}}\) | 0 | Not Defined |
Also Read:
| Related Articles | ||
|---|---|---|
| Sin cos Formulas | Sin 90 Degrees | Sin 0 Degrees |
| Sin 120 Degrees | Sin 180 Degrees | Sec 90 Degrees |
| Cos 60 Degrees | Cos 180 Degrees | Cos 120 Degrees |
Things to Remember
- The value of Sin 30 degrees is ½ or 0.5.
- Sin 30 degrees is also written as Sin \(\frac{\pi}{6}\) or sin (0.523598..)
- The decimal value of sin 30 degree and Cos 60 degree is same i.e. 0.50
- Sin 30° is lying in the first quadrant so its final value will be positive.
- Sinθ reciprocal is Cosecθ. We also say that Sinθ = Perpendicular / Hypotenuse, then Cosecθ = Hypotenuse / Perpendicular.
- For the conversion of degree to radian we use, θ in radians = θ in degree* (Pi/180).
Read More: Cosine Rule Important Notes
Sample Questions
Ques: In a Right angle triangle ABC, Side AB = 24cm & side BC = 7 cm, Find
1) Sin A & Cos A
2) Cos C & Sin C (2015 CBSE, 3 marks)

Ans: In triangle ABC,
Given: side AB = 24cm & BC = 7 cm
By using Pythagoras theorem,
AC2 = AB2+ BC2
AC2 = 242+ 72
AC2 = 576 + 49 = 625
AC = 25
Now for,
1). Sin A = BC/AC = 7/25
Cos A = AB/AC = 24/25.
2). Cos C =BC/AC = 7/25
Sin C = AB/AC =24/25.
Ques: In a Right angle triangle ABC, Side AB = 30cm & side BC = 10 cm, Find Sin A & Cos A (2 marks)
Ans: In triangle ABC,
Given: side AB = 30 cm & BC = 10 cm
By using Pythagoras theorem,
AC2 = AB2+ BC2
AC2 = 302+ 102
AC2 = 900 + 100 = 1000
AC = 31.62
Now for,
Sin A = BC/AC = 10/31.62 = 0.316
Cos A = AB/AC = 30/31.62 = 0.94
Ques: Find the value of Sin230 + tan245+ cot2 60 (2 marks)
Ans: From the trigonometric table,
We know that,
Sin 30 = ½
Tan 45 = 1
Cot 60 = \(\frac{1}{\sqrt{3}}\)
Therefore,
→ (1/2)2 + 12 +( \(\frac{1}{\sqrt{3}}\))2
→ ¼ + 1 + 1/3
= 1.58
Ques: Find the value of Sin230 + Sin245+ Sin2 60 (2 marks)
Ans: From the trigonometric table,
We know that,
Sin 30 = ½
Sin 45 = \(\frac{1}{\sqrt{2}}\)
Sin 60 = \(\frac{\sqrt{3}}{2}\)
Therefore,
→ (1/2)2 + (\(\frac{1}{\sqrt{2}}\))2 +(\(\frac{\sqrt{3}}{2}\))2
→ ¼ + ½ + ¾
= 1.5
Ques: Find tan A – cot C in figure? (3 marks)

Ans: In right angle triangle, ABC
By using Pythagoras theorem, we get
BC2 = AC2 – AB2
QR2 = 132 – 122
= (13-12) (13+12)
= 1* 25 = 25
BC2 = 25
BC = 5
Now,
Tan A = BC/AB = 5/12
Cot C = BC/AB = 5/12
So, tan A – cot C = 5/12 - 5/12 = 0
Ques: What is the value of sin(-30)? [CBSE, 2014, 2 marks]
Ans: We already know that the value of sin 30 = 0.5
sin (-30) = - sin (30)
therefore, sin (-30) = - 0.5
Ques: What is the value of sin 30 + cos 30? (2 marks)
Ans: We already know from the trigonometric table,
Sin 30 = ½
Cos 30 = \(\frac{\sqrt{3}}{2}\)
Therefore,
½ + \(\frac{\sqrt{3}}{2}\) = 1.36
Ques: What is the value of sin 30 + tan 30? (2 marks)
Ans: We already know from the trigonometric table,
Sin 30 = ½
Tan 30 = \(\frac{1}{\sqrt{3}}\)
Therefore,
½ + \(\frac{1}{\sqrt{3}}\) = 1.07
Ques: What is the value of sin 30 + Cos 60? (2 marks)
Ans: We already know from the trigonometric table,
Sin 30 = ½
Cos 60 = ½
Therefore,
½ + ½ = ¼
= 0.25
Ques: In right angled triangle ABC, Angle ACB = 30° and AB = 5 cm. Find the lengths of AC and BC sides? [CBSE, 2018, 3 marks]

Ans: Given: ∠ACB = 30° and side AB = 5 cm.
We know that,
Tan θ = Perpendicular/ Base
So, Tan C = AB/ BC or tan 30° = 5/ BC
1/ √3 = 5cm /BC (by using table, tan30° = 1/√3)
And also, BC = 5√3 cm.
Now, for third side by using trigonometric identities we get
Sin C = AB/ AC or Sin 30° = 5cm / AC
We know that Sin 30° = ½.
So, ½ = 5 cm /AC
AC = 10 cm.
Check-Out:






Comments