
Content Curator
Roots of Polynomials are solutions for given polynomials where the function is equal to zero. To find the root of the polynomial, you need to find the value of the unknown variable. If the root of the polynomial is found then the value can be evaluated to zero. So, the roots of the polynomials are also called its zeros.
| Table of Content |
Key Takeaways: Introduction of Polynomials, Roots of Polynomials, Monomials, Quadratic Polynomials, Polynomial Roots, Formula for Polynomial Roots, Three Degree Polynomial Roots
What are Polynomials?
Polynomial is composed of two terms: Poly (which means "many") and Nominal (which means "terms."). A polynomial is a mathematical equation made up of variables, constants, and exponents that are mixed using operations like addition, subtraction, multiplication, and division (No division operation by a variable). It is classed as monomial, binomial, or trinomial based on the number of terms in the expression. The following are some examples of constants, variables, and exponents:
- Constants. For instance, 1, 2, 3, and so on.
- Variables. For instance, g, h, x, y, and so on.
- Exponents: For instance, 5 in x5 and so on.
The video below explains this:
Polynomials Detailed Video Explanation:
Roots of Polynomials
Roots of polynomials are the solutions to any given polynomial for which the unknown variable's value must be determined. We can evaluate the value of a polynomial to zero if we know the roots. A polynomial of degree 'n' in variable x is an expression of the type anxn + an-1xn-1 +...... + a1x + a0, where each variable has a constant as its coefficient. The phrase refers to each variable in an expression that is separated by an addition or subtraction symbol. The greatest power of a polynomial variable is defined as the degree of the polynomial.

Roots of Polynomials
A polynomial of degree 1 is, for example, a linear polynomial of the form ax+ b. Quadratic polynomials have a degree of 2 while cubic polynomials have a degree of 3.

Monomials and Polynomials
A monomial is a polynomial that has only one term. A polynomial of zero degrees is a monomial containing only a constant term. Even if the constants' values are greater than zero, a polynomial can account for a null value. In such circumstances, we look for the values of variables that cause the polynomial's value to be zero. The roots of polynomials refer to the values of a variable. They are sometimes referred to as polynomial zeros.
Also Read: Nature of Roots
Roots of Polynomials: Formula
Polynomials are expressions that look like this:
anxn+an-1xn-1+......+a1x+a0
The root of a linear polynomial such as ax + b has the formula
x = -b/a
A quadratic polynomial has the general form ax2 + bx + c, and when this expression is equated to zero, we get a quadratic equation, ax2 + bx + c = 0

Roots of Polynomials
The formula is used to assess the roots of two-degree quadratic equations, such as
ax2 + bx + c = 0
\(x = {-b \pm \sqrt{b^2-4ac} \over 2a}\)
Higher degree polynomial formulas are a little more difficult.
Also Read: Polynomials Formula
Three-Degree Polynomial Roots
To discover the roots of a three-degree polynomial, we must first factor the provided polynomial equation into a linear and quadratic equation. The zeros of the three-degree polynomial can then be easily determined. Let's look at an example to help us comprehend.
2x3 − x2 − 7x + 2
Because x – 2 is one of the factors, divide the provided polynomial by it.
2x3 − x2 − 7x + 2 = (x – 2) (2x2 + 3x – 1)
Now that we have one linear equation and one quadratic equation for which we know the formula, we can find the roots of the aforementioned polynomial.
\(ax^3 + bx^2 + cx + d = 0 \)
has roots \(\alpha , \beta, \gamma\) then
\(\Sigma a = -\frac{b}{a} , \Sigma \alpha \beta = \frac{c}{a}\)
\(\alpha \beta \gamma = - \frac{d}{a}\)
Finding Polynomial Roots
Consider the following example of the polynomial p(x) of degree 1:
5x + 1 Equals p(x)
If P(a) = 0, 'a' is the root of a polynomial p(x), according to the definition of roots of polynomials.
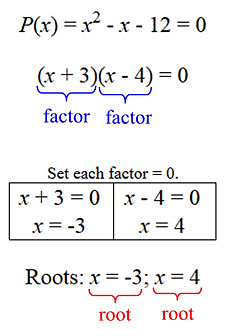
To identify the roots of the polynomial p(x), we must first establish the value of x for which p(x) = 0. Now,
0 = 5x + 1
x = -1/5
As a result, the root of the polynomial p is '-1/5'. (x).
Also Read:
| Degree of polynomial | Real Numbers | Linear Equation in Two Variable |
| Operations on Rational Numbers | Degree of a Polynomial: Zero & Constant Polynomial | Types Of Triangles |
Things To Remember
- A polynomial is a mathematical equation made up of variables, constants, and exponents that are combined using addition, subtraction, multiplication, and division operations.
- Based on the number of terms in the equation, polynomials are classified as monomial, binomial, or trinomial.
- The values of the variable that cause the polynomial to evaluate to zero are called the roots of the polynomial. Both will result in a value of 3 for the polynomial.
- In solving sets of equalities, finding roots is a means to an end (and is useful for understanding inequalities as well). If you need to determine the intersection of two lines, for example, you build up equalities and solve for the unknowns.
Sample Questions
Ques. What's the best way to find the roots of a polynomial? What is the significance of an equation's roots? (3 marks)
Ans. How many roots are there? Examine the polynomial's highest-degree term or the one with the highest exponent. The exponent represents the number of roots the polynomial will have. So if your polynomial's highest exponent is 2, it will have two roots; if it is 3, it will have three roots, and so on.
In solving sets of equalities, finding roots is a means to an end (and is useful for understanding inequalities as well). If you need to determine the intersection of two lines, for example, you build up equalities and solve for the unknowns.
Ques. What are the fundamental processes in writing a polynomial with roots? (3 marks)
Ans. The fundamental processes in writing a polynomial with roots are:
- Change the signs and place each factor in parenthesis as you write the roots as factors.
- Multiply two roots together using a box to keep track of the results.
- Check to see if each element has been multiplied by the others.
Ques. What is the definition of a polynomial formula? What is the significance of the polynomial equation? (4 marks)
Ans. A polynomial formula is a mathematical phrase that expresses a polynomial. A polynomial expression is an expression that has two or more terms (algebraic terms). A polynomial expression is created by adding or subtracting binomials or monomials repeatedly.
Polynomials are an important aspect of mathematics and algebra's "language." They are used to express numbers as a result of mathematical operations in almost every branch of mathematics. Other sorts of mathematical expressions, such as rational expressions, use polynomials as "building blocks."
Ques. What are the different sorts of polynomials? A polynomial is determined by how many points it has? (4 marks)
Ans. There are three sorts of polynomials based on the number of terms in a polynomial. Monomial, binomial, and trinomial are the three types. Polynomials are classified as zero or constant polynomials, linear polynomials, quadratic polynomials, and cubic polynomials based on their degree.
Two points decide a degree 1 polynomial (a line), three points produce a degree 2 polynomial, four points determine a degree 3 polynomial, and so on, according to Property 2.
Ques. What role do polynomials play in real-life situations? (3 marks)
Ans. Polynomials play a very important role in solving real-life problems. People utilize polynomials to graph curves in the real world because they are used to describe many types of curves. Roller coaster designers, for example, might employ polynomials to define the curves in their rides. In economics, polynomial function combinations are sometimes used to undertake cost evaluations, for example.
Ques. What are the polynomial rules? Is it true that all polynomials have roots? (3 marks)
Ans. An expression must have no square roots of variables, no fractional or negative powers on the variables, and no variables in the denominators of any fractions to be considered a polynomial term.
Any non-negative real number has a square root, as does every polynomial with an odd degree and real coefficients.
Ques. What are polynomial zeros? (3 marks)
Ans. All the x-values that make the polynomial equal to 0 are called the zeros of a polynomial p(x) or polynomial zeros. They're fascinating to us for a variety of reasons, one of which is that they reveal the graph's x-intercepts. We'll also observe that they're closely tied to the polynomial factors.
Ques. In polynomials, what is a term? (3 marks)
Ans. Polynomials are algebraic formulas that contain any number of terms that are added or subtracted together. A number, a variable, or a compound of a number and one or more variables with exponents is referred to as a term. To simplify a polynomial, like terms (the same variable or variables raised to the same power) can be combined.
Ques. Why is finding the roots of a polynomial equation useful? What is the relationship between the factors and roots of a polynomial equation? (3 marks)
Ans. Finding the roots of a polynomial equation is useful for a variety of reasons. This is because it was discovered that the equations we want to solve can be changed into comparable equations with one side set to zero. So, if we can solve that instance, we should be able to handle other cases as well!
Relationship:
The polynomial has at least one root, according to the Fundamental Theorem of Algebra. If r is a root, then (x-r) is a factor, according to the Factor Theorem. When a polynomial of degree n is divided by a factor (x-r) of degree 1, you get a polynomial of degree n-1.
Ques. A polynomial has how many real roots? (2marks)
Ans. A polynomial of even degree can have any number of unique real roots, ranging from 0 to n. A polynomial of odd degrees can have any number of unique real roots, ranging from one to n. Except for the fact that polynomials of odd degrees must have at least one real root, this is of little use.
Ques. What are the most significant factors to consider when determining whether or not a polynomial exists? (4 marks)
Ans. Points to Remember
- A polynomial has the form ???? + ???? ???? + ???? ???? + ? + ???? ???? . ...
- The value of the variable's exponent is the degree of a monomial.
- A sum of monomials is a polynomial.
- The highest degree of a polynomial's monomials is its degree.
Ques. What is a polynomial's real root? Is it true that all polynomials have roots? (3 marks)
Ans. Because the graph of a polynomial meets the x-axis, the terms solutions, zeros, and roots are interchangeable. Real roots are the roots identified when the graph intersects the x-axis; they can be seen and dealt with as real numbers in the real world.
Any non-negative real number has a square root, as does every polynomial with an odd degree and real coefficients.





Comments