Function Notation Formula & Solved Examples

Function notation formula is massively used in algebra and geometry. Primarily, the sets Relations and functions are parts of the set theory. Sets help to distinguish the groups of certain kinds of different objects. In this article, we will get to know the basics of the functions right from the basics of origin to the polynomial functions which are highly required to understand the function notation formula. The function is represented by the letter ‘f’. Sometimes,the letters 'g' or 'h' are also used to represent the function with the notation. The input variable which is generally taken as ‘x’ or ‘y’, is enclosed inside the parentheses. Here the element which is taken for the variable is generally chosen within the domain of the function. The functions have general notation as y = f(x) orf: X→Y

Keyterms: Function, Algebra, Geometry, Relation, Set, Polynomial function, Notation, Set operation, Set theory

---

Sets, Relations and Functions

[Click Here for Sample Questions]

Sets, Relations and Functions are all the sub-concepts of the set theory. The sets and relations were used to carry out the logical and some of the mathematical set operations.

• Set: A set can be defined as the representation of a collection of objects; distinct objects with one or morecommon representations.

If ‘B’ is a set and ‘a’ one of its elements then: ‘a ∈ B’ denotes that element ‘a’ belongs to ‘B’ whereas, ‘a ∉ B’ denotes that ‘a’ is not an element of the set B. The sets are represented by the Venn diagrams.

• Relations: The relation can be defined as the subset of the Cartesian product, which contains only some of the ordered pairs based on the relationship that is defined between the first and the next elements.

A relation is usually denoted by R.

Total no. of relations possible for n(A×B) are 2^{n(A) × n(B)}.

Cartesian product of two given sets A and B, A × B is given by

A × B = {(a, b): a ∈ A, b ∈ B}

In particular R × R = {(x, y): x, y ∈ R}, for a 2×2 relation

and R × R × R = (x, y, z): x, y, z ∈ R}, for a 3 × 3 relation.

• Function: If every element of any set is related to one and only one element of another set, this kind of relation qualifies as a function.

A function is a special case of relation wherein no 2 ordered pairs can have a similar first element.

A function can be described in three different ways which are Graphical form, Algebraic form and Tabular form.

---

Domain and range

[Click Here for Sample Questions]

• Domainis a group of all the possible values which are independent variables of the function it can assume. So, domain refers to the set of possible values that ‘x’ can take in the function f. A domain is generally the set of preimages.
• Codomainis the group of all the possible values which are the dependent variables of the function that it can assume.
• Rangeis a group of elements from set B that have the corresponding pre-image in set A. A range refers to the set of all the possible values of the function that we assume upon taking the different values of x inthe domain.

Cartesian product A × B of two sets A and B is given by

A × B = {(a, b): a ∈ A, b ∈ B}

In particular R × R = {(x, y): x, y ∈ R}

and R × R × R = (x, y, z): x, y, z ∈ R}, for a 3 × 3 relation.

This means that the codomain refers to the set of all the possible values that ‘y’ can take in the function given.                                                                              

Relation between domain, codomain and Range

---

Notation of function

[Click Here for Sample Questions]

The representation can be done as:

y = f(x) or f : A→B

Here,

f denotes the name of the function.

x is an element from the domain set A.

y or f(x) is the element from the range set B.

the element of the domain x, is the input variable producing an output f(x).

The arrow indicates the mapping between the input to the output.

Notation of function

The above diagram depicts the relation of y and x as y = x² , where x and y are real numbers. This equation tells us y is dependent on x because y is the square value of x. In more technical terms, y is a function of x. This specification can be seen in the above notation.

A real function will have both the domain and the codomain as the subsets of the Real numbers set R.

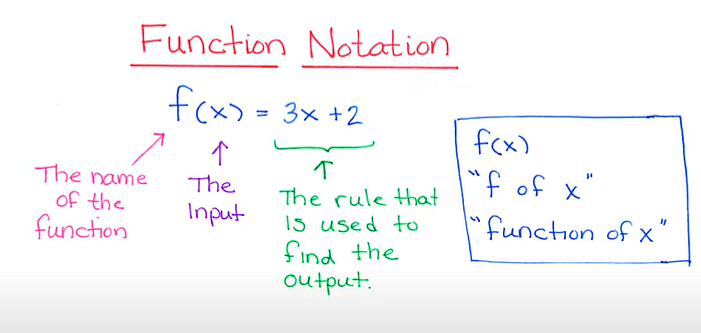
Function Notation Formula

Algebra of functions For functions f : X → R and g : X → R, we have

(f + g) (x) = f (x) + g(x), x ∈ X

(f – g) (x) = f (x) – g(x), x ∈ X

(f.g) (x) = f (x) . g (x), x ∈ X

(kf) (x) = k (f (x) ), x ∈ X, Here k is a real number.

---

Things to remember

1. The domain, codomain must belong to the real numbers set.
2. The total number of relations that can be defined from a set A to a set B is the number of possible subsets of A × B. If n (A) = p and n(B) = q, then n (A × B) = pq and the total number of relations is 2pq.
3. Range ⊆ codomain. (The range is always a subset of the codomain).
4. If A and B are non-empty sets and either A or B is an infinite set, then so is A × B.
5. Two ordered pairs are equal, only if their corresponding first elements are equal and the second elements also are equal.

---