Logarithms: Rules, Properties and Formula

 The notion of logarithms was first presented in the 17th century by John Napier. Many scientists, navigators, engineers, and others then utilized it to accomplish numerous computations, making it simple. To put it another way, logarithms are the inverse of exponentiation. Here, the concept, characteristics, and examples of logarithm will be discussed in-depth along with some important questions.  

Multiplication, division, or rational powers of large numbers are all examples of numerical expressions. Logarithms come in handy for such computations. They assist us in simplifying complex calculations.

---

bx = a ⇔ log_ba = x

Here, on the right side of the arrow represents a logarithm of a base b that is equal to x. Here,

1. a and b are considered as the two positive real numbers.
2. x is the real number.
3. a which is inside the log is known as the argument.
4. b which is at the bottom of the log is known as the base.

---

Logarithms Explanation

The power to which a number must be increased to obtain additional values is defined as a logarithm. It's the most practical technique of expressing enormous amounts of data. Multiplication and division of logarithms can alternatively be expressed as logarithms of addition and subtraction, according to a number of significant properties of logarithms.

 “The logarithm of a positive real number a with respect to base b, a positive real number not equal to 1[nb1], is the exponent by which b must be raised to yield a”.

i.e bx= a and it is read as “the logarithm of a to base b.”

In other words, the logarithm gives the answer to the question “How many times a number is multiplied to get the other number?”. 

For example, how many 3’s are multiplied to get the answer 27?

If we multiply 3 for 3 times, we get the answer 27. 

Therefore, the logarithm is 3.

The logarithm form is written as follows:

Log₃(27) = 3 

Therefore, the base 3 logarithm of 27 is 3.

The above logarithm form can also be written as:

3x3x3 = 27

3³ = 27 

Thus, the equations and both represent the same meaning.

Read more: Logarithmic Differentiation​

---

Logarithm Examples

10¹ = 10 ⇒ log₁₀10 = 1

10² =100⇒log₁₀100= 2

10³ =1000⇒log₁₀1000= 3

10⁴ = 10000 ⇒ log₁₀10000 = 4

3² = 9 ⇒ log₃9 = 2

3⁵ = 243 ⇒ log₃243 = 5 

Example 1: Solve log₂ (64) =?

Solution: As 26= 2 × 2 × 2 × 2 × 2 × 2 = 64, 

6 is the exponent value and log₂ (64)= 6.

Example 2: What is the value of log₁₀(100)?

Solution: In this case, 10 2 gives you 100. 

So, 2 is the exponent value where the value of log10(100)= 2

---

Logarithm Types

In most cases, we always deal with two different types of logarithms, namely

• Common Logarithm
• Natural Logarithm

Common Logarithm

The common logarithm, also known as the base 10 logarithms, is represented as log10 or simply log. For instance, the common logarithm of 1000 is represented as a log (1000). The common logarithm shows how many times we have to multiply the number 10 in order to get the required output.

For example, log (100) = 2

If we multiply the number 10 twice, we will get the result 100.

Natural Logarithm

The natural logarithm, also considered to be the base e logarithm, is represented as ln or loge. In the natural logarithm, “e” represents the Euler’s constant that is approximately equal to 2.71828. For instance, the natural logarithm of 78 is written as ln 78. The natural logarithm is therefore how many we have to multiply “e” so that we get the required output.

For example, ln (78) = 4.357.

Therefore, the base e logarithm of 78 is equivalent to 4.357. 

Also Check:

Logarithm Rules and Properties

Logarithmic operations can be carried out according to a set of rules. These principles are referred to as:

• Product rule
• Division rule
• Power rule/Exponential Rule
• Change of base rule
• Base switch rule
• Derivative of log
• Integral of log

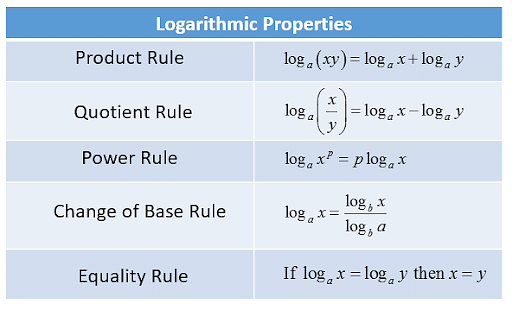
Product Rule

In the product rule, the multiplication of two logarithmic values is equivalent to the addition of their individual logarithms.

Log_b(mn)= log_bm + log_bn

For example,

For log₇(3x):

We can see that inside the bracket there are two variables, 3 and x. Now we will use the product rule to solve the logarithm

log_a(3x) = log_a(3) + log_a(x)

By using the property of the product we can also simplify the two variables of a logarithm into a single logarithm. It must be noted that the bases of the logarithm should be the same in two variables to use the property of the product while simplifying the variables into one.
For example,

We cannot use the property of the product to simplify a logarithm which is 

log₄(8) + log₆(x)

Division Rule

The division of two logarithmic values is equivalent to the difference of each logarithm.

Log_b (m/n)= log_b m – log_b n

By solving the logarithm of log₅(r/4):

We are going to write this logarithm equation into different forms by using the property of quotients.

log₅(r/4) = log₅(r) - log₅(4)

Let’s understand how to calculate the different forms of a logarithm into dividend and divisor forms. 

log₃(4) - log₃(h) = log₃(4/h)

When we simplify the different forms of a logarithm into dividend and divisor forms, the bases of the logarithm should be the same.

Exponential Rule

The logarithm of m with a rational exponent is equivalent to the exponent times its logarithm in the exponential rule.

Log_b (mn) = n log_b m

By solving log₃(x²) :  

Here, by using the property of power of logarithm we will convert the single logarithm into multiple logarithms.

log₃(x²) = 2.log₃(x) 

Now, a multiple of a logarithm will be converted into a single logarithm.

log₃(9) = log₃(95) = log₃(59049)

Change of Base Rule

Log_b m = log_a m/ log_a b    

Example: log_b 2 = log_a 2/log_a b

Base Switch Rule

log_b (a) = 1 / log_a (b)

Example: log_b 8 = 1/log8 b

Derivative of log

If f (x) = log_b (x), then the derivative of f(x) is given by;

f'(x) = 1/(x ln(b))

Example: Given, f (x) = log₁₀ (x)

Then, f'(x) = 1/(x ln(10))

Integral of Log

\(\int\)log_b(x)dx = x( log_b(x) – 1/ln(b) ) + C

Example:\(\int\)log₁₀(x) dx = x ? ( log₁₀(x) – 1 / ln(10) ) + C

Other Properties

Some other properties of logarithmic functions are:

• Log_b b = 1
• Log_b 1 = 0
• Log_b 0 = undefined

---

Applications of Logarithm

People in today's world of contemporary technology are constantly looking for methods to make things simpler and easier.  As a result, calculators and logarithms were invented to make solving mathematical problems easier. 

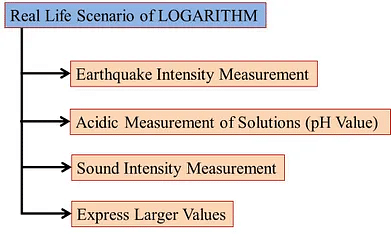
Let's look at some more benefits of knowing logarithms:

• Logarithms are utilized in a wide range of scientific disciplines as well as many other businesses.
• Because the value for pH might be small, we utilize the logarithm to have a range for utilizing it for small values, which helps us discover the pH value in chemistry.
• In finance, logarithms are widely utilized.
• The half-life of radioactive substances is calculated using logarithms.
• We can use it to determine the earthquake's intensity.
• Even in the field of medicine and engineering, logarithms and their properties are used.

---

Things to Remember

• A logarithm is referred to as the power to which a number has to be raised in order to get some other values. It is the most convenient way to express the large numbers. The logarithm can be defined by using the exponent as follows: bx = a ⇔ log_ba = x
•  It's the most practical technique of expressing enormous amounts of data. Multiplication and division of logarithms can alternatively be expressed as logarithms of addition and subtraction, according to a number of significant properties of logarithms.
• In most cases, we always deal with two different types of logarithms, namely, Common Logarithm and Natural Logarithm.
• Logarithmic operations can be carried out according to a set of rules. These principles are referred to as: Product rule, Division rule, Power rule/Exponential Rule, Change of base rule, Base switch rule, Derivative of log, Integral of log.
• Some other properties of logarithmic functions are: Log_b b = 1, Log_b 1 = 0, Log_b 0 = undefined. 
• Logarithms are utilized in a wide range of scientific disciplines as well as many other businesses.
• Because the value for pH might be small, we utilize the logarithm to have a range for utilizing it for small values, which helps us discover the pH value in chemistry.

---