Difference between Ln and Log: Definitions and Properties

Content Curator

Difference between ln and log is that ln is defined for base e while log is defined for base 10 i.e., log_e = ln. Ln is also called natural log. In mathematics, logarithm is defined as the inverse function of exponentiation. In simpler words, logarithm is the power (exponent) to which a base has to be raised to attain a given number i.e., the number of times a base should be multiplied by itself to attain a given number. Logarithms help to cover a large range of data/values in a crisp manner. Due to this reason, it has numerous applications in the field of mathematics, physics and chemistry.

Read More: Value of e

Table of Content

Definition of ln
Definition of log
Difference between ln and log
Application of Ln and Log
Things to Remember
Sample Questions

Read More: Trigonometry Table

Definition of Ln

Ln is known as the natural logarithm i.e., logarithm with the base e (an irrational and a transcendental number) which is approximately equal to 2.7183. It is referred to by ln x or log_e x. Here, e is the Euler’s constant.

Also Read:

Limit Formula	Surface Area of a Cone Formula
Midpoint Formula	Geometry Formula

log_e x = ln x = e^x = y

A table representing the ln values of 1 to 10 is:

ln (1)	0
ln (2)	0.6931
ln (3)	1.0986
ln (4)	1.3863
ln (5)	1.6094
ln (6)	1.7918
ln (7)	1.9459
ln (8)	2.0794
ln (9)	2.1972
ln (10)	2.3026

Rules of Ln

Power Rule:

Ln (xy) = y * ln (x)

Example: ln (53) = 3 * ln (5)

Quotient Rule:

Ln (x/y) = ln (x) – ln (y)

Example: ln (7/3) = ln (7) – ln (3)

Read More:

Types Of Triangles	Properties of Triangle	Formula Of Perimeter Shapes

Reciprocal Rule:

Ln (1/x) = - ln (x)

Example: ln (1/5) = - ln (5)

Also Read:

Value of log 0	Real Valued Function
Geometric Progression	Geometric Mean

Definition of Log

Log is known as the logarithm which is defined for the base 10. It is also called common logarithm.

Read More:

Quadrilateral Formula	Trapezoid Formula	Tan2x Formula

Log₁₀ y = x

10x = y

A table representing the log values of 1 to 10 is:

log (1)	0
log (2)	0.3010
log (3)	0.4771
log (4)	0.6020
log (5)	0.6989
log (6)	0.7781
log (7)	0.8450
log (8)	0.9030
log (9)	0.9542
log (10)	1

Properties of Log:

Log (mn) = log m + log n

Example: log (3*5) = log (3) + log (5)

Log (m/n) = log m – log n

Example: log (3/2) = log (3) – log (2)

Read More:

Transversal	Isosceles triangle	Scalene Triangle

Log (mn) = n log m

Example: log (52) = 2 * log (5)

Log_b m = log_a m/log_a b

Example: log₃ 8 = log₂ 8 * log_a 2

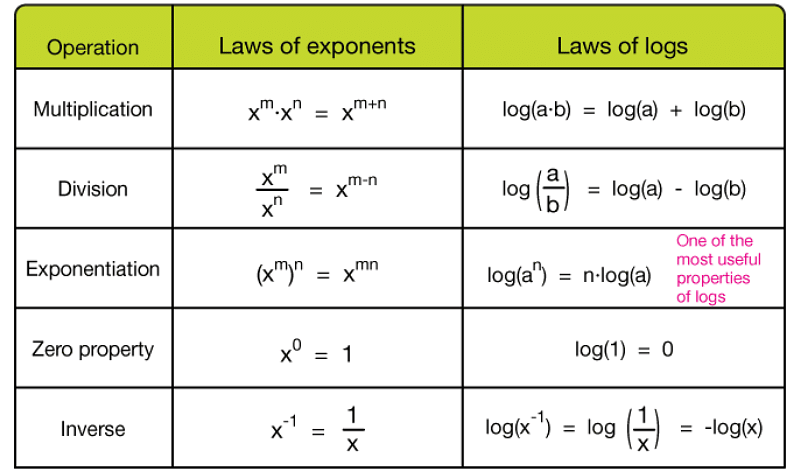
Difference between Ln and Log

S. No.	Ln	Log
1.	Ln is defined as the logarithm with base e	Log is defined as the logarithm with base 10
2.	It is also known as natural logarithm	It is also known as common logarithm
3.	It is represented as log_e x	It is represented as log₁₀ x
4.	Its exponential form is e^x= y	Its exponential form is 10^x = y
5.	General statement for exponential logarithm is “By what number must e be raised to attain y”	General statement for exponential logarithm is “By what number must 10 be raised to attain y”
6.	It is mathematically represented as log base e	It is mathematically represented as log base 10
7.	It finds less application in physics	It finds more application in physics

Application of Ln and Log

The real life applications are:

Logarithms are used in the Richter Scale for earthquakes.
It is also used in the detection of the password strength
For data scientists, logarithms help in seeing the patterns in bulk data
Log odds play a pivotal role in logistic regression

Also Read:

Trigonometric Functions	Complex numbers and Quadratic Equations
Sequence and Series	Arithmetic Progression

Points to Remember

The general form of a logarithm is:

log_a (y) = x

The above expression can also be denoted as:

a^x = y

log_e x = ln x = e^y = y
log_a 1 = 0
log_a a = 1
Ln (x) = 2.303 * log (x)
Log (x) = ln (x) / 2.303

Also Read:

Cosine Formula	Centroid Formula
Binomial Expansion Formula	Angle Formula

Sample Questions

Ques 1. Define logarithms. [1 mark]

Ans. Logarithm of a given number with base x is the power to which x should be raised to attain the given number.

a^x = y

log_a y = x

Ques 2. Define natural and common logarithms. [2 mark]

Ans. Natural logarithm is a logarithm with the base e(Euler’s constant which is approximately equal to 2.7183) i.e., log_e x = ln x = e^x

Common logarithm is a logarithm with the base 10 i.e., log₁₀ x

Read More:

Integers As Exponents?	Ordinate?	Collinear points?

Ques 3. What is the value of log 9 when log 27 is equal to 1.431? [3 mark]

Ans. Given,

Log 27 = 1.431

Log (33) = 1.431

3 * log 3 = 1.431

Log 3 = 1.431/3

Log 3 = 0.447

Log 9 = log (32)

Log 9 = 2 * log 3

Log 9 = 2 * 0.447

Log 9 = 0.954

Ques 4. Given that the value of log₁₀ 2 = 0.3010, what is the value of log₁₀ 80? [3 mark]

Ans. Log₁₀ 80 = log₁₀ (8 * 10)

Log₁₀ 8 + log₁₀ 10

Log₁₀ (23) + 1

3 * log 2 + 1

3 * 0.3010 + 1

0.9030 + 1

Hence, the value of log10 80 is 1.9030

Read More:

Operations on Rational Numbers	Addition And Subtraction Of Integers	Multiplication And Division Of Integers

Ques 5. If the value of log₁₀ 5 + log₁₀ (5x + 1) = log₁₀ (x + 5) + 1, what is the value of x? [4 mark]

Ans. Given,

log₁₀ 5 + log₁₀ (5x + 1) = log₁₀ (x + 5) + 1

log₁₀ 5 + log₁₀ (5x + 1) = log₁₀ (x + 5) + log₁₀ 10

log₁₀ [5 (5x + 1)] = log₁₀ [10(x + 5)]

5(5x + 1) = 10(x + 5)

5x + 1 = 2x + 10

3x = 9

Hence, the value of x is 3.

Ques 6. If the value of log₁₀ 7 = a, then log₁₀ (1/70) is equal to? [3 mark]

Ans. log₁₀ (1/70) = log₁₀ 1 – log₁₀ 70

= - log₁₀ (7 * 10)

= - (log₁₀ 7 + log₁₀ 10)

= - (a + 1)

Read More:

Surface Area of a Cylinder Formula	Sphere Formula	Slope Formula

Ques 7. How can you convert natural log to common log? [2 mark]

Ans. To convert a natural logarithmic value to common logarithmic value, the following formula can be used:

Ln (x) = log (x) ÷ log (2.71828)

Ques 8. Find the value of (1/log₃ 60 + 1/log₄ 60 + 1/log₅ 60) [3 mark]

Ans. Given,

(1/log₃ 60 + 1/log₄ 60 + 1/log₅ 60)

= log₆₀ (3 * 4 * 5)

= log₆₀ (60)

Hence, the answer to this question is 1.

Also Read:

Inverse Trigonometric Formulas	Area of a Kite Formula
Volume of a Pyramid Formula & Solved Example	Exponential Growth Formula with Solved Examples

CBSE CLASS XII Related Questions

1.
If $ f(x) = \begin{cases} \frac{\sin^2 ax}{x^2}, & \text{if } x \neq 0 \\ 1, & \text{if } x = 0 \end{cases} $ is continuous at $ x = 0 $, then the value of 'a' is :
- 1
- -1
- 0
- $ \pm 1 $
View Solution
2.
Find $ \int \frac{3x + 1}{(x - 2)^2 (x + 2)} \, dx $
View Solution
3.
Let \[ A = \begin{pmatrix} 1 & 4 \\ -2 & 1 \end{pmatrix} \quad \text{and} \quad C = \begin{pmatrix} 3 & 4 & 2 \\ 12 & 16 & 8 \\ -6 & -8 & -4 \end{pmatrix}. \] Then, find the matrix $B$ if $AB = C$.
View Solution
4.
Solve the differential equation $ (x - \sin y) \, dy + (\tan y) \, dx = 0 $, given $ y(0) = 0 $.
View Solution
5.
$ \int \frac{e^{10 \log x} - e^{8 \log x}}{e^{6 \log x} - e^{5 \log x}} \, dx$ is equal to :
- $x + C$
- $\frac{x^2}{2} + C$
- $\frac{x^4}{4} + C$
- $\frac{x^3}{3} + C$
View Solution
6.
Based upon the results of regular medical check-ups in a hospital, it was found that out of 1000 people, 700 were very healthy, 200 maintained average health and 100 had a poor health record.
Let $ A_1 $: People with good health,
$ A_2 $: People with average health,
and $ A_3 $: People with poor health.
During a pandemic, the data expressed that the chances of people contracting the disease from category $ A_1, A_2 $ and $ A_3 $ are 25%, 35% and 50%, respectively.
Based upon the above information, answer the following questions:
(i) A person was tested randomly. What is the probability that he/she has contracted the disease?}
(ii) Given that the person has not contracted the disease, what is the probability that the person is from category $ A_2 $?
View Solution