Latus Rectum of Parabola: Formula, Length & Derivation

Content Curator

Latus rectum of a parabola is the line passing through its foci which is parallel to the directrix of the parabola and perpendicular to the axis of the parabola. A parabola has only one latus rectum whereas an ellipse and a hyperbola have 2 latus rectums.

Length of Latus Rectum of a Parabola LL’ = 4a

The focus of the parabola lies exactly at the midpoint of the length of the latus rectum and they are all collinear in nature. For a parabola, the length of the Latus Rectum is 4 times the distance between the focus and the vertex.

Table of Content

What is a Parabola?
Latus Rectum of Parabola
Derivation of Length of Latus Rectum of Parabola
Things to Remember
Sample Questions

Key Terms : Parabola, Latus Rectum, Derivation of Length, vertex, Hyperbola

What is a Parabola?

[Click Here for Sample Questions]

Parabola is the locus of a point that moves in a plane, such that its distance from a fixed point is equal to its perpendicular distance from a fixed straight line. There are four standard equations of a parabola as follows:

y² = 4ax
y² = – 4ax
x² = 4ay
x² = – 4ay

Parabola

The important formulas relating to the Latus Rectum of a parabola are tabulated below.

Parabola	Vertex	Focus	Axis of Symmetry	Directrix	Length of Latus Rectum	Endpoints of Latus Rectum
y² = 4ax	(0,0)	(a,0)	y = 0	x = -a	4a	(a,±2a)
y² = – 4ax	(0,0)	(-a,0)	y = 0	x = a	4a	(-a,±2a)
x² = 4ay	(0,0)	(0,a)	x = 0	y = -a	4a	(±2a,a)
x² = – 4ay	(0,0)	(0,-a)	x = 0	y = a	4a	(±2a,-a)

Discover about the Chapter video:

Conic Sections Detailed Video Explanation:

Latus Rectum of a Parabola

[Click Here for Sample Questions]

Latus rectum of a conic section is a chord that is parallel to the directrix and passes through the focus. The half-length of the Latus Rectum is called Semi-Latus Rectum. The Latus Rectum of a parabola is a line segment perpendicular to the axis of the parabola, which passes through the focus and whose endpoints lie on the parabola.

The length of the latest rectum is denoted by 4a

where a is the distance between the vertex and the focus of the parabola.

The half-length of the Latus Rectum is called Semi-Latus Rectum and it is denoted by 2a.

The endpoints of the Latus Rectum lie on the parabola, which is denoted as L(a,2a) and L’(a,-2a).

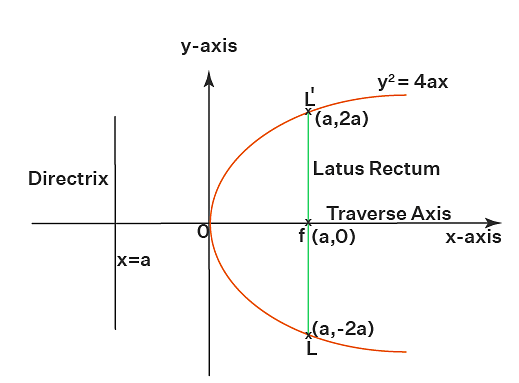
Latus Rectum of a Parabola

Latus Rectum of a Parabola

Two parabolas are said to be equal if they have the Latus Rectum of the same length.

Also Read: Latus Rectum of Ellipse

Derivation of Length of the Latus Rectum

[Click Here for Sample Questions]

We know that the endpoints on the Latus Rectum are L(a,2a) and L’(a,-2a). Hence, to find the length of the Latus Rectum, all we have to do is find the distance between the points L and L’.

Using Distance Formula, the length LL’ is

\(\to\) \(\sqrt{[(a - a)² + {2a - (-2a)}²]} \)

\(\to\)[0 + {2a + 2a}²]

\(\to\)[4a²]

\(\to ±\)4a

As the distance cannot be negative, we get the length of the Latus Rectum as 4a.

Things to Remember

[Click Here for Sample Questions]

Latus rectum of a parabola is the line passing through its foci which is parallel to the directrix of the parabola.
Length of Latus Rectum of a Parabola LL’ = 4a.
For a parabola, the length of the Latus Rectum is 4 times the distance between the focus and the vertex.
The end points of the latus rectum of a parabola with standard equation y² = 4ax is (a,±2a)

Sample Questions

Ques. Find the equation of the parabola with vertex at (0, 0) and focus at (0, 2). [2 marks]

Ans: Since the vertex is at (0,0) and the focus is at (0,2) which lies on the y-axis, the y-axis is the axis of the parabola. Therefore, the equation of the parabola is of the form x² = 4ay. Thus, we have,

x² = 4(2)y

i.e., x² = 8y

Ques. Find the length of the latus rectum of the parabola y² - 2y = -2x - 3. [2 marks]

Ans: y² - 2y = -2x - 3

(y - 1)² - 1 = -2x - 3

(y - 1)² = -2x - 3 + 1

(y - 1)² = -2x - 2

(y - 1)² = -2(x + 1)

Therefore, the length of the Latus Rectum is 2.

Ques. For parabola y²=84x, what are the endpoints and length of the Latus Rectum? [2 marks]

Ans: The standard equation of the parabola is

y²=4ax

Comparing it with y²=84x, we get

4ax = 84x

4a = 84

a = 21

We know that the endpoints on the Latus Rectum are L(a,2a) and L’(a,-2a).

Therefore, the endpoints are L(21,42) and L’(21,-42).

Also, the length of the Latus Rectum is 84.

Ques. For parabola y²=96x, what are the endpoints and length of the Latus Rectum? [2 marks]

Ans: The standard equation of the parabola is

y²=4ax

Comparing it with y²=96x, we get

4ax = 96x

4a = 96

a = 24

We know that the endpoints on the Latus Rectum are L(a,2a) and L’(a,-2a).

Therefore, the endpoints are L(24,48) and L’(24,-48).

Also, the length of the Latus Rectum is 96.

Ques. For parabola y²=-24x, what are the endpoints and length of the Latus Rectum? [2 marks]

Ans: The standard equation of the parabola is

y²=-4ax

Comparing it with y²=-24x, we get

4ax = 24x

4a = 24

a = 6

We know that the endpoints on the Latus Rectum are L(-a,2a) and L’(-a,-2a).

Therefore, the endpoints are L(-6,12) and L’(-6,-12).

Also, the length of the Latus Rectum is 24.

Ques. For parabola x²= – 36y, what are the endpoints and length of the Latus Rectum? [2 marks]

Ans: The standard equation of the parabola is

x²=-4ay

Comparing it with x²=-36y, we get

4ay = 36y

4a = 36

a = 9

We know that the endpoints on the Latus Rectum are L(2a, -a) and L’(-2a, -a).

Therefore, the endpoints are L(18,-9) and L’(-18,-9).

Also, the length of the Latus Rectum is 36.

Ques. For parabola x²=-72y, what are the endpoints and length of the Latus Rectum? [2 marks]

Ans: The standard equation of the parabola is

x²=-4ay

Comparing it with x²=-72y, we get

4ay = 72y

4a = 72

a = 18

We know that the endpoints on the Latus Rectum are L(2a, -a) and L’(-2a, -a).

Therefore, the endpoints are L(36,-18) and L’(-36,-18).

Also, the length of the Latus Rectum is 72.

Ques. For parabola x²=64y, what are the endpoints and length of the Latus Rectum? [2 marks]

Ans: The standard equation of the parabola is

x²=4ay

Comparing it with x²=64y, we get

4ay = 64y

4a = 64

a = 16

We know that the endpoints on the Latus Rectum are L(2a, a) and L’(-2a, a).

Therefore, the endpoints are L(32,16) and L’(-32,16).

Also, the length of the Latus Rectum is 64.

Also Read:

Circle	Standard Equation of Circle	Ellipse
Eccentricity of Ellipse	Hyperbola	Latus Rectum of Hyperbola
Distance between Two Points	Horizontal and Vertical Lines	Angle Between Two Lines

Related Links:

NCERT Solutions for Class 11 Maths	Important Formulas in Maths	Maths Study Materials

Latus Rectum of Parabola: Formula, Length & Derivation

What is a Parabola?

Conic Sections Detailed Video Explanation:

Latus Rectum of a Parabola

Derivation of Length of the Latus Rectum

Things to Remember

Sample Questions

CBSE CLASS XII Related Questions

1.
Find the vector and the cartesian equations of the lines that pass through the origin and(5,-2,3).

2.
By using the properties of definite integrals, evaluate the integral: \(∫_0^π log(1+cosx)dx\)

3.
If A'= \(\begin{bmatrix} 3 & 4 \\ -1 & 2 \\ 0 &1 \end{bmatrix}\)\(\begin{bmatrix} -1 & 2 & 1 \\ 1 &2 & 3\end{bmatrix}\) , then verify that
(i) \((A+B)'=A'+B' \)
(ii) \((A-B)'=A'-B'\)

4.
If \(\frac{d}{dx}f(x) = 4x^3-\frac{3}{x^4}\) such that \(f(2)=0\), then \(f(x)\) is

5.
If (i) A=\(\begin{bmatrix} \cos\alpha & \sin\alpha\\ -\sin\alpha & \cos\alpha \end{bmatrix}\),then verify that A'A=I
(ii) A= \(\begin{bmatrix} \sin\alpha & \cos\alpha\\ -\cos \alpha & \sin\alpha \end{bmatrix}\),then verify that A'A=I

6.
For what values of x,\(\begin{bmatrix} 1 & 2 & 1 \end{bmatrix}\)\(\begin{bmatrix} 1 & 2 & 0\\ 2 & 0 & 1 \\1&0&2 \end{bmatrix}\)\(\begin{bmatrix} 0 \\2\\x\end{bmatrix}\)=O?

Similar Mathematics Concepts

Comments

SUBSCRIBE TO OUR NEWS LETTER

Latus Rectum of Parabola: Formula, Length & Derivation

What is a Parabola?

Conic Sections Detailed Video Explanation:

Latus Rectum of a Parabola

Derivation of Length of the Latus Rectum

Things to Remember

Sample Questions

CBSE CLASS XII Related Questions

1. Find the vector and the cartesian equations of the lines that pass through the origin and(5,-2,3).

2. By using the properties of definite integrals, evaluate the integral: \(∫_0^π log(1+cosx)dx\)

3. If A'= \(\begin{bmatrix} 3 & 4 \\ -1 & 2 \\ 0 &1 \end{bmatrix}\)\(\begin{bmatrix} -1 & 2 & 1 \\ 1 &2 & 3\end{bmatrix}\) , then verify that (i) \((A+B)'=A'+B' \)(ii) \((A-B)'=A'-B'\)

4. If \(\frac{d}{dx}f(x) = 4x^3-\frac{3}{x^4}\) such that \(f(2)=0\), then \(f(x)\) is

5. If (i) A=\(\begin{bmatrix} \cos\alpha & \sin\alpha\\ -\sin\alpha & \cos\alpha \end{bmatrix}\),then verify that A'A=I(ii) A= \(\begin{bmatrix} \sin\alpha & \cos\alpha\\ -\cos \alpha & \sin\alpha \end{bmatrix}\),then verify that A'A=I

6. For what values of x,\(\begin{bmatrix} 1 & 2 & 1 \end{bmatrix}\)\(\begin{bmatrix} 1 & 2 & 0\\ 2 & 0 & 1 \\1&0&2 \end{bmatrix}\)\(\begin{bmatrix} 0 \\2\\x\end{bmatrix}\)=O?

Similar Mathematics Concepts

Comments

SUBSCRIBE TO OUR NEWS LETTER

1.
Find the vector and the cartesian equations of the lines that pass through the origin and(5,-2,3).

2.
By using the properties of definite integrals, evaluate the integral: \(∫_0^π log(1+cosx)dx\)

3.
If A'= \(\begin{bmatrix} 3 & 4 \\ -1 & 2 \\ 0 &1 \end{bmatrix}\)\(\begin{bmatrix} -1 & 2 & 1 \\ 1 &2 & 3\end{bmatrix}\) , then verify that
(i) \((A+B)'=A'+B' \)
(ii) \((A-B)'=A'-B'\)

4.
If \(\frac{d}{dx}f(x) = 4x^3-\frac{3}{x^4}\) such that \(f(2)=0\), then \(f(x)\) is

5.
If (i) A=\(\begin{bmatrix} \cos\alpha & \sin\alpha\\ -\sin\alpha & \cos\alpha \end{bmatrix}\),then verify that A'A=I
(ii) A= \(\begin{bmatrix} \sin\alpha & \cos\alpha\\ -\cos \alpha & \sin\alpha \end{bmatrix}\),then verify that A'A=I

6.
For what values of x,\(\begin{bmatrix} 1 & 2 & 1 \end{bmatrix}\)\(\begin{bmatrix} 1 & 2 & 0\\ 2 & 0 & 1 \\1&0&2 \end{bmatrix}\)\(\begin{bmatrix} 0 \\2\\x\end{bmatrix}\)=O?