Latus Rectum of Hyperbola: Definition, Equations, Formula and Sample Questions

Content Curator

Latus rectum of the hyperbola is a line segment perpendicular to the transverse axis and passes through any of the foci with end points lying on the hyperbola.

The topic ‘Latus Rectum of Hyperbola’ falls under Chapter 11 Conic Sections of CBSE Class 11 Mathematics Syllabus. Conic Sections with other chapters of Unit-3 i.e Coordinate Geometry carries a combined weightage of 10 Marks in the CBSE Class 11 Mathematics Examination.

Table of Content

Hyperbola
Latus Rectum
Latus Rectum of Conic Sections
Latus Rectum of Hyperbola
Sample Questions

Also read: Calculus Formula

Hyperbola

[Click Here for Sample Questions]

A hyperbola is the locus of all the points in a plane in such a way that the difference in their distances from the fixed points in the plane is a constant.

The figure shows the basic shape of the hyperbola with its parts. We have four-point P₁, P₂, P₃, and P₄ at certain distances from the focus F₁ and F₂.

Discover about the Chapter video:

Conic Sections Detailed Video Explanation:

Latus Rectum

[Click Here for Sample Questions]

In a Conic Section, the latus rectum is the chord that passes through the focus, and is parallel to the directrix. The word latus rectum is derived from the Latin word- latus which means side and the rectum mean straight.

Also read: Definite Integral Formula

Latus Rectum of Conic Sections

[Click Here for Sample Questions]

The latus rectum of the conic sections is the chord that passes through the focus and is perpendicular to the main axis. Both the starting point and the ending points of the latus rectum are on the curve.

The length of the latus rectum different for all the conic sections:

The Latus rectum is forever equivalent to the length of the diameter in a circle.
The length of the latus rectum in the hyperbola is square of the length of the transverse axis separated by the length of the conjugate axis.
The length of the latus rectum in a parabola is four-times to its focal length.
The length of the latus rectum in an ellipse is square to the length of the conjugate axis divided by the length of the transverse axis.

Conic Section	Ends of the Latus Rectum	Length of the Latus Rectum
y² = 4ax	L = (a, 2a), L’ = (a, -2a)	4a
(x²/a²) + (y²/b²) =1	L = (ae, b²/a), L = (ae, – b²/a)	If a>b; 2b²/a
(x²/a²) + (y²/b²) =1	L = (ae, b²/a), L = (ae, – b²/a)	If b>a; 2a²/b
(x²/a²) – (y²/b²) =1	L = (ae, b²/a), L = (ae, – b²/a)	2b²/a

Latus Rectum of Hyperbola

[Click Here for Sample Questions]

The line segments perpendicular to the transverse axis through any of the foci such that their endpoints lie on the hyperbola are defined as the latus rectum of a hyperbola.

The length of the latus rectum of Hyperbola is 2b²/a.

Also read: Determinant Formula

Length of Latus Rectum of Hyperbola

In the above figure,

LSL' and TS'T' are the latus rectum and LS is the semi latus rectum.

The coordinates of L are (ae, SL)

Since, L lies on the hyperbola.

(x²/a²) - (y²/b²) = 1

The coordinate will satisfy the equation of the hyperbola

((ae)²/a²) - ((SL)²/b²) = 1

(a²e²/a²) - ((SL)²/b²) = 1

e² - 1 = ((SL)²/b²)

(SL)² = b²(e² - 1) ….(1)

b² = a² (e² - 1)

(e² - 1) = b²/a²

On putting the values of (e² - 1) in (1), we get

(SL)² = b²(b²/a²)

(SL)² = b⁴/a²

SL = b²/a (length of semi latus rectum)

SL + SL' = 2b²/a

LSL' = 2b²/a

Hence it proves that the length of Latus Rectum of Hyperbola is 2b²/a

Also read: Trapezoid Formula

Sample Questions Based on Latus rectum of Hyperbola

Ques.1: Find the length of the latus rectum of the hyperbola x²− 4y²= 4. (3 Marks)

Answer: Given Equation of Hyperbola:

x²− 4y²= 4

⇒x²/22 − y²/1 = 1

⇒a = 2, b = 1

Thus length of the latus rectum is

2b²/a = 1

Ques.2: Find the equation of the hyperbola whose foci are (0,+-12,) and Latus Rectum is 36. (4 Marks)

Answer: Let the eccentricity of Hyperbola be e.

Let the eccentricity of Hyperbola

Ques.3: Determine the equation of the hyperbola which satisfies the given conditions: Foci (0, ±13), the conjugate axis is of length 24. (5 Marks)

Answer:

Ques.4: Find the coordinates of the foci and the vertices, the eccentricity and the length of the latus rectum of the hyperbolas. \(\frac{y^2}{9} - \frac{x^2}{27} = 1\) (5 Marks)

Answer:

Find the coordinates of the foci and the vertices, the eccentricity and the length of the latus rectum of the hyperbola

Ques.5: Find the coordinates of the foci and the vertices, the eccentricity and the length of the latus rectum of the hyperbolas. 9y² – 4x² = 36 (5 Marks)

Answer: Given a Hyperbola equation,

9y² – 4x² = 36

Find the coordinates of the foci and the vertices, the eccentricity and the length of the latus rectum of the hyperbolas. 9y2 – 4x2 = 36

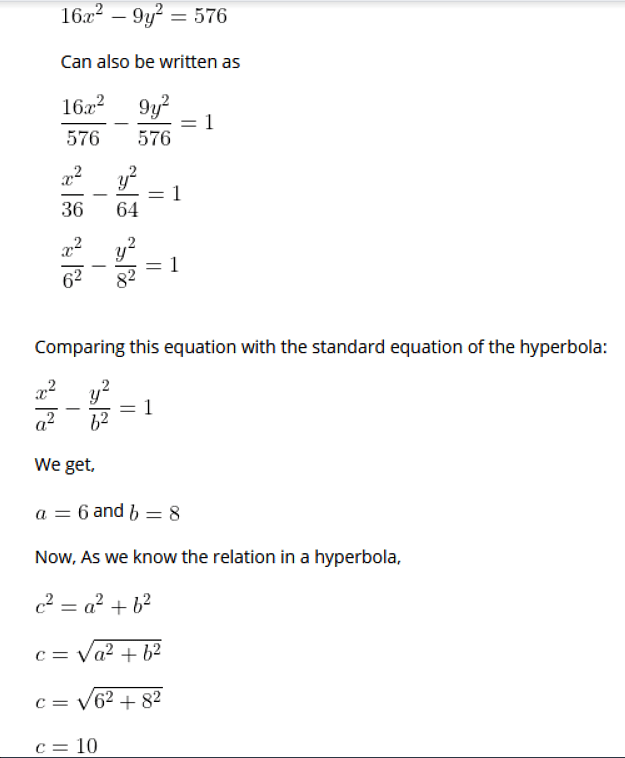
Ques.6: Find the coordinates of the foci and the vertices, the eccentricity and the length of the latus rectum of the hyperbolas. 16x² – 9y² = 576 (5 Marks)

Answer:Given a Hyperbola equation,

Also Read:

Isosceles triangle	Scalene Triangle
Types Of Triangles	Properties of Triangle
Differential Equation	Difference between Sequence and Series
First Order Differential Equation	Differentiation and Integration Formula
Eccentricity	Eccentric Formula
Events in Probability	Probability Distribution

CBSE CLASS XII Related Questions

1.
Find the following integral: \(\int (ax^2+bx+c)dx\)

View Solution

2.
Find the inverse of each of the matrices,if it exists \(\begin{bmatrix} 2 & 1 \\ 7 & 4 \end{bmatrix}\)

View Solution

3.
Find the vector and the cartesian equations of the lines that pass through the origin and(5,-2,3).

View Solution

4.
If (i) A=\(\begin{bmatrix} \cos\alpha & \sin\alpha\\ -\sin\alpha & \cos\alpha \end{bmatrix}\),then verify that A'A=I
(ii) A= \(\begin{bmatrix} \sin\alpha & \cos\alpha\\ -\cos \alpha & \sin\alpha \end{bmatrix}\),then verify that A'A=I

View Solution

5.
Let A=\(\begin{bmatrix} 0 & 1 \\ 0 & 0 \end{bmatrix}\),show that(aI+bA)ⁿ=aⁿI+na^n-1bA,where I is the identity matrix of order 2 and n∈N

View Solution

6.
If A'= \(\begin{bmatrix} 3 & 4 \\ -1 & 2 \\ 0 &1 \end{bmatrix}\)\(\begin{bmatrix} -1 & 2 & 1 \\ 1 &2 & 3\end{bmatrix}\) , then verify that
(i) \((A+B)'=A'+B' \)
(ii) \((A-B)'=A'-B'\)

View Solution

View All

CBSE CLASS XII Previous Year Papers

Mathematics

Mathematics Preparation Guides

Sequence and Series: Types, Formulas & Examples

Measures of Dispersion: Types, Absolute & Relative measure

Events and their Types in Probability

Angle between two lines: Derivation, Types, Formulae

Mathematics: Latus rectum of Ellipse- Definition, Equation, Examples and Sample Questions

Pascal’s Triangle: Construction, Notation, Pattern, Properties

Ellipse: Standard Equation, Derivation & Formula

Circle: Definition, Equation and Segments

Geometric Progression: Definition, Sum of N Terms, Formula

Conic Sections: Formula, Equations & Examples

Parabola: Graph, General Equation, Formulae, Latus Rectum, Focal, Normal , Tangent, Sample Questions

Horizontal and Vertical Lines: Formula, Examples & Equations

Conic Sections: Parabola, Hyperbola, Ellipse, Circle

Eccentricity of Ellipse Formula, Definition, Derivation & Examples

Complex Numbers and Quadratic Equations

Real Valued Functions: Types, Operations & Examples

Latus Rectum of Parabola: Formula, Length & Derivation

Venn Diagrams: Symbols, Formula, Set Operations & Examples

Types of Events in Probability

Types of Sets: What is Set, Examples & Symbols

Mathematics NCERT Solutions

Factorial Formula: Calculation, Values, Application

Multiplication Rule of Probability: Proof and Solved Examples

Logarithms: Rules, Properties and Formula

Mathematical Logic: Conjunction, Disjunction and Negation

Value of Log 1: Logarithmic Functions, Value of In 1, Logarithmic Values

Solving Inequalities

Tautology: Operators, Logic Symbols, and Truth Table

Quotient Rule: Definition, Formula, Applications and Sample Questions

Conjugate of a Complex Number: Definition, Properties and Equations

Difference between Ln and Log: Definitions and Properties

Latus Rectum of Hyperbola: Definition, Equations, Formula and Sample Questions

Hyperbola

Conic Sections Detailed Video Explanation:

Latus Rectum

Latus Rectum of Conic Sections

Latus Rectum of Hyperbola

Length of Latus Rectum of Hyperbola

Sample Questions Based on Latus rectum of Hyperbola

CBSE CLASS XII Related Questions

1.
Find the following integral: \(\int (ax^2+bx+c)dx\)

2.
Find the inverse of each of the matrices,if it exists \(\begin{bmatrix} 2 & 1 \\ 7 & 4 \end{bmatrix}\)

3.
Find the vector and the cartesian equations of the lines that pass through the origin and(5,-2,3).

4.
If (i) A=\(\begin{bmatrix} \cos\alpha & \sin\alpha\\ -\sin\alpha & \cos\alpha \end{bmatrix}\),then verify that A'A=I
(ii) A= \(\begin{bmatrix} \sin\alpha & \cos\alpha\\ -\cos \alpha & \sin\alpha \end{bmatrix}\),then verify that A'A=I

5.
Let A=\(\begin{bmatrix} 0 & 1 \\ 0 & 0 \end{bmatrix}\),show that(aI+bA)ⁿ=aⁿI+na^n-1bA,where I is the identity matrix of order 2 and n∈N

6.
If A'= \(\begin{bmatrix} 3 & 4 \\ -1 & 2 \\ 0 &1 \end{bmatrix}\)\(\begin{bmatrix} -1 & 2 & 1 \\ 1 &2 & 3\end{bmatrix}\) , then verify that
(i) \((A+B)'=A'+B' \)
(ii) \((A-B)'=A'-B'\)

Comments

SUBSCRIBE TO OUR NEWS LETTER

Latus Rectum of Hyperbola: Definition, Equations, Formula and Sample Questions

Hyperbola

Conic Sections Detailed Video Explanation:

Latus Rectum

Latus Rectum of Conic Sections

Latus Rectum of Hyperbola

Length of Latus Rectum of Hyperbola

Sample Questions Based on Latus rectum of Hyperbola

CBSE CLASS XII Related Questions

1. Find the following integral: \(\int (ax^2+bx+c)dx\)

2. Find the inverse of each of the matrices,if it exists \(\begin{bmatrix} 2 & 1 \\ 7 & 4 \end{bmatrix}\)

3. Find the vector and the cartesian equations of the lines that pass through the origin and(5,-2,3).

4. If (i) A=\(\begin{bmatrix} \cos\alpha & \sin\alpha\\ -\sin\alpha & \cos\alpha \end{bmatrix}\),then verify that A'A=I(ii) A= \(\begin{bmatrix} \sin\alpha & \cos\alpha\\ -\cos \alpha & \sin\alpha \end{bmatrix}\),then verify that A'A=I

5. Let A=\(\begin{bmatrix} 0 & 1 \\ 0 & 0 \end{bmatrix}\),show that(aI+bA)n=anI+nan-1bA,where I is the identity matrix of order 2 and n∈N

6. If A'= \(\begin{bmatrix} 3 & 4 \\ -1 & 2 \\ 0 &1 \end{bmatrix}\)\(\begin{bmatrix} -1 & 2 & 1 \\ 1 &2 & 3\end{bmatrix}\) , then verify that (i) \((A+B)'=A'+B' \)(ii) \((A-B)'=A'-B'\)

Comments

SUBSCRIBE TO OUR NEWS LETTER

1.
Find the following integral: \(\int (ax^2+bx+c)dx\)

2.
Find the inverse of each of the matrices,if it exists \(\begin{bmatrix} 2 & 1 \\ 7 & 4 \end{bmatrix}\)

3.
Find the vector and the cartesian equations of the lines that pass through the origin and(5,-2,3).

4.
If (i) A=\(\begin{bmatrix} \cos\alpha & \sin\alpha\\ -\sin\alpha & \cos\alpha \end{bmatrix}\),then verify that A'A=I
(ii) A= \(\begin{bmatrix} \sin\alpha & \cos\alpha\\ -\cos \alpha & \sin\alpha \end{bmatrix}\),then verify that A'A=I

5.
Let A=\(\begin{bmatrix} 0 & 1 \\ 0 & 0 \end{bmatrix}\),show that(aI+bA)ⁿ=aⁿI+na^n-1bA,where I is the identity matrix of order 2 and n∈N

6.
If A'= \(\begin{bmatrix} 3 & 4 \\ -1 & 2 \\ 0 &1 \end{bmatrix}\)\(\begin{bmatrix} -1 & 2 & 1 \\ 1 &2 & 3\end{bmatrix}\) , then verify that
(i) \((A+B)'=A'+B' \)
(ii) \((A-B)'=A'-B'\)