
Jasmine Grover Content Strategy Manager
Content Strategy Manager
Differentiation rules allow us to evaluate the derivatives of specific functions. The attribute of linearity is crucial for the procedure of differentiation or finding the derivative of a function. For functions produced from the fundamental elementary functions using the methods of addition and multiplication by a constant integer, this characteristic makes the derivative more natural.
| Table of Content |
Key Takeaways: Differentiation rules, Trigonometric, Inverse Trigonometric, Functions, Addition, Division, Multiplication, Division
What are Differentiation Rules?
[Click Here for Sample Questions]
The derivatives should be evaluated correctly. In fact, differentiating a function helps to provide a universal outcome. As a result, you must comprehend and observe some differentiation concepts or rules. Some Important rules of differentiation include power rule, product rule, chain rule, quotient rule and difference rule. These rules are also sometimes known as derivative rules.
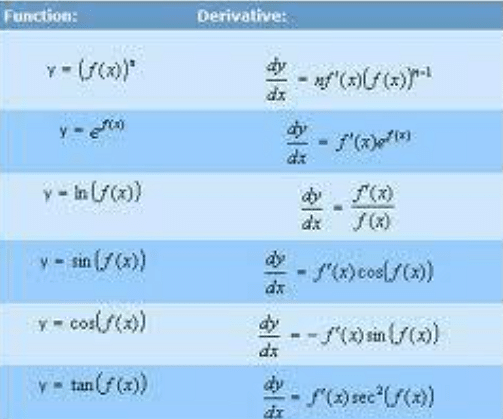
Differentiation Rules
Discover about the Chapter video:
Continuity and Differentiability Detailed Video Explanation:
Also Read:
| Related Articles | ||
|---|---|---|
| Coefficient of Static Friction | Linear Equation in Two Variables | Pair of Linear Equations in Two Variables Formula |
Rules of Differentiation
[Click Here for Sample Questions]
Some of the important rules of differentiation are:
Power Rule
This is a primary differentiating rule and it is very easy to understand . Let’s see how a function changes when a power method is implemented.
If f(x) = xn,
Then, f’(x) = d/dx (xn) = nxn-1
Let’s look at an example, then it will make you understand the application properly.
If f(x) = x6
Then, d/dx (x6) = 6x6-1 = 6x5
Sum Rule
The sum rule of differentiation suggests the following changes when a function is expressed by the difference or methods of integration.
If f(x) = m(x) ± n(x)
Then, f’(x) = m’(x) ± n’(x)
This formula suggests that the smaller functions’ signs will be preserved, but these functions will adhere to the derivative rules. Consider the following example.
If f(x) = x2 + x3
Then, f’(x) = 2x + 3x2
This is how the sum rule of derivatives is implemented.
Product Rule
This rule states that if a variable's function is the product of two other functions, then the result will be as follows.
If f(x) = m(x) × n(x),
Then, f’(x) = m′(x) × n(x) + m(x) × n′(x)
Look at this example to get a better understanding of this rule,
If f(x) = x2 × x3
Then, f’(x) = d/dx (x2 × x3)
= x3 × d/dx (x2) + x2 × d/dx (x3)
= x3 × 2x + x2 × 3x2
= 2x4 + 3x4
= 5x4
Quotient Rule
The quotient rule derivatives indicate how to differentiate a function with two terms in division mode. The rule suggests the following.
If f(x) = m(x) / n(x),
Then, f′(x) = m’(x) × n(x) − m(x) × n’(x) / (n(x))2
If you follow the rule and enter the values of the functions after the differential equation, you will get the required answer.
Chain Rule
When a function is expressed by a function with another variable, and this function is expressed by the first function's variable, the chain rule suggests the differential operation below.
If f(x) = m(u) and u = n(x),
Then, f’(x) = d/dx f(x) = d/du m(u) × d/dx n(x)
This formula or rule is relatively simple to apply if you pay attention to the radio waves in each stage and understand how to prove the chain rule.
Differentiation Rules for Trigonometric Functions
[Click Here for Sample Questions]
There are about six trigonometric functions such as, sin, cos, tan, csc, sec, and cot. Here are the given rules or formulas to help you determine the derivatives of trigonometric functions.
- The derivative of sin x is, d/dx (sin x) = cos x
- The derivative of cos x is, d/dx (cos x) = - sin x
- The derivative of tan x is, d/dx (tan x) = sec2x
- The derivative of csc x is, d/dx (csc x) = - csc x cot x
- The derivative of sec x is, d/dx (sec x) = sec x tan x
- The derivative of cot x is, d/dx (cot x) = - csc2x
Also Read: Quadratic Equations Formula
Differentiation Rules for Inverse Trigonometric Functions
[Click Here for Sample Questions]
To the aforementioned trigonometric functions, there exist 6 inverse trigonometric functions. Remember that there are no trigonometric/inverse trigonometric functions involved in the derivatives of inverse trigonometric functions. The rules are as follows:
- The derivative of inverse sine is, d/dx (sin-1x) = 1/√(1-x2)
- The derivative of inverse cosine is, d/dx (cos-1x) = -1/√(1-x2)
- The derivative of inverse tan is, d/dx (tan-1x) = 1/(1 + x2)
- The derivative of inverse cosec is, d/dx (csc-1x) = -1/ [x √(x2 - 1) ], x ≠ 1, -1, 0
- The derivative of inverse sec is, d/dx (sec-1x) = 1/ [x √(x2 - 1) ], x ≠ 1, -1, 0
- The derivative of inverse cot is, d/dx (cot-1x) = -1/(1 + x2)
Things to Remember
Following are some important points:
- The chain rule has been known since the end of the 17th century, when Isaac Newton and Leibniz first unearthed calculus.
- Calculations involving computing the derivatives of complicated expressions, such as those encountered in many physics applications, are made easier with this rule.
- We can find rates of change using differentiation. It enables us to determine the rate of change of velocity with respect to time, for example.
- Differentiation rules help us to evaluate the derivatives of specific functions.
- Some of the Important rules of differentiation are power rule, product rule, chain rule, quotient rule and difference rule.
Also Read:
| Related Articles | ||
|---|---|---|
| Calculus Formula | Application of derivatives | Factorial Formula |
| Rolle's Theorem | Conditional Probability Formula | Integers As Exponents |
Sample Questions
Ques: f(x) = x + x3. (3 Marks)
Ans: With the help of sum rule of derivative here, we have:
f’(x) = u’(x) + v’(x)
Now, differentiating the given function, we get;
f’(x) = d/dx(x + x3)
f’(x) = d/dx(x) + d/dx(x3)
f’(x) = 1 + 3x2
Ques: Determine the derivative of the function f(x) = 6x2 – 4x. (3 Marks)
Ans: Given function is: f(x) = 6x2 – 4x
This is in the form of: f(x) = u(x) – v(x)
So after applying the difference rule of derivatives, we get,
f’(x) = d/dx (6x2) – d/dx(4x)
= 6(2x) – 4(1)
= 12x – 4
Hence, f’(x) = 12x – 4
Ques: State the derivative of x2(x+3). (4 Marks)
Ans: By applying product rule of derivative, we know;
f′(x) = u′(x) × v(x) + u(x) × v′(x)
Here,
u(x) = x2 and v(x) = x+3
So, on differentiating the given function, we get;
f’(x) = d/dx[x2(x+3)]
f’(x) = d/dx(x2)(x+3)+x2d/dx(x+3)
f’(x) = 2x(x+3)+x2(1)
f’(x) = 2x2+6x+x2
f’(x) = 3x2+6x
f’(x) = 3x(x+2)
Ques: Differentiate f(x)=(x+2)3/√x. (3 Marks)
Ans: Given,
f(x)=(x+2)3/√x
= (x+2)(x2+4x+4)/√x
= [x3+6x2+12x+8]/x1/2
= x-1/2(x3+6x2+12x+8)
= x5/2+6x3/2+12x1/2+8x-½
Now, differentiating the given equation, we get;
f’(x) = 5/2x3/2 + 6(3/2x1/2)+12(1/2x-1/2)+8(−1/2x-3/2)
= 5/2x3/2 + 9x1/2 + 6x-½ − 4x-3/2
Ques: Differentiate f(x) = (x4 – 1)50. (4 Marks)
Ans: Given,
f(x) = (x4 – 1)50
Let g(x) = x4 – 1 and n = 50
u(t) = t50
Thus, t = g(x) = x4 – 1
f(x) = u(g(x))
According to chain rule,
df/dx = (du/dt) × (dt/dx)
Here,
du/dt = d/dt (t50) = 50t49
dt/dx = d/dx g(x)
= d/dx (x4 – 1)
= 4x3
Therefore, df/dx = 50t49 × (4x3)
= 50(x4 – 1)49 × (4x3)
= 200 x3(x4 – 1)49
Ques: Determine the derivative of f(x) = esin(2x). (3 Marks)
Ans: Given,
f(x) = esin(2x)
Let t = g(x) = sin 2x and u(t) = et
According to chain rule,
df/dx = (du/dt) × (dt/dx)
Here,
du/dt = d/dt (et) = et
dt/dx = d/dx g(x)
= d/dx (sin 2x)
= 2 cos 2x
Therefore, df/dx = et × 2 cos 2x
= esin(2x) × 2 cos 2x
= 2 cos(2x) esin(2x)
Ques: Determine the derivative of 2x? (1 Mark)
Ans: We know that according to the formula for the derivative of cx, c is a constant. So, the derivative of 2x is 2.
Ques: Determine the derivative of the function: f(x) = (x + a) / (x + b). (2 Marks)
Ans: Let f(x) = (x + a) / (x + b) = u/v
By quotient rule of derivatives,
(u/v)' = (vu' - uv')/v2
By using this,
f'(x) = [ (x + b) d/dx (x + a) - (x + a) d/dx (x + b)] / (x + b)2
= [ (x + b) (1) - (x + a) (1)] / (x + b)2
= (x + b - x - a) / (x + b)2
= (b - a) / (x + b)2
Ques: With the help of derivative rules, State the derivative of f(x) = (ax2 + b)2. (2 Marks)
Ans: The given function is, f(x) = (ax2 + b)2. Here, one function is inside the other. So we will apply the chain rule.
f'(x) = 2(ax2 + b) d/dx (ax2 + b)
= 2(ax2 + b) (2ax)
= 4ax(ax2 + b)
Ques: Determine the derivative of f(x) = x sin-1x with the help of differentiation rules. (2 Marks)
Ans: The given function is f(x) = x sin-1x = u v.
Because this is a product of two different functions, we can implement the product rule here.
(uv)' = uv' + vu'
f'(x) = x d/dx (sin-1x) + sin-1x d/dx (x)
= x (1/√(1-x2) ) + sin-1x (1)
= x/√(1-x2) + sin-1x.
For Latest Updates on Upcoming Board Exams, Click Here: https://t.me/class_10_12_board_updates
Check-Out:






Comments