
Content Writer
Mode is the value that appears most frequently in a set of data in statistics. The value or number in a data collection with a high frequency or appears more frequently is referred to as the mode or modal value. It is one of the three measures of central tendency, together with the mean and median. The word mode comes from the French phrase La Mode, which means "fashionable."
Read More: Arithematic Formula
| Table of Content |
Key Terms: Mode, Unimodal Mode, Trimodal Mode, Modal Class, Mean, Median, Statistics
What is Mode?
[Click Here for Sample Questions]
A mode is defined as the value with the highest frequency. It's the value that shows up the most frequently.
- For example, the mode of the data set in the given set of data: 2, 4, 5, 5, 6, 7 is 5 because it appears twice in the collection.
- Mode is a method of summarising important information about random variables or populations in a single number, similar to the statistical mean and median.
- In a normal distribution, the modal value is the same as the mean and median, however in a severely skewed distribution, the modal value might be considerably different.
- As an example, the Mode is 6 in {6, 3, 9, 6, 6, 5, 9, 3} as the number 6 has occurred often. As a result, we may easily find the mode with a finite number of observations. A set of values can have only one mode, multiple modes, or none at all.
Mean, Median and Mode Video Explanation
Read More: Geometric Mean
Explanation of Mode
[Click Here for Previous Year Questions]
- In statistics, we represent a set of data by a representative value that roughly defines the full collection of data for this purpose. The measure of central tendency is the name given to this representative value.
- It is implied by the term that it is a value that the data is centred on. We may generate a statistical overview of the huge ordered data using these metrics of central tendency.
- The mode of data is one such measure of central tendency.
- When examining categorical data, such as the most popular soda flavours or bike models, where average median values based on order cannot be derived, mode is the most helpful indicator of central tendency.
Read More:
| Relevant Concepts | ||
|---|---|---|
| Standard Deviation | Median | Dispersion |
| Frequency Distribution Table Statistics | Variance | Weighted Mean |
Types of Mode
[Click Here for Sample Questions]
Unimodal Mode
A unimodal mode is a set of data with only one mode.
The mode of data set A = {14, 15, 16, 17, 15, 18, 15, 19}, for example, is 15 because just one value repeats itself. As a result, it's a unimodal data set.

Unimodal Mode
Bimodal Mode
A bimodal mode is a set of data that has two modes. This indicates that the data values with the highest frequencies are two.
Set A = {2,2,2,3,4,4,5,5,5} has a mode of 2 and 5, because both 2 and 5 are repeated three times in the provided set.

Bimodal Mode
Trimodal Mode
A trimodal mode is a set of data that has three modes. This indicates that the top three data values have the most frequency.
Set A = {2,2,2,3,4,4,5,5,5,7,8,8,8} has a mode of 2, 5, and 8 since all three numbers are repeated thrice in the provided set. As a result, it's a trimodal data collection.

Trimodal Mode
Multimodal Mode
A multimodal mode is a set of data that contains four or more modalities.
Because all four values in the given set recur twice, the mode of data set A = 100, 80, 80, 95, 95, 100, 90, 90,100,95 is 80, 90, 95 and 100. As a result, it's a multimodal dataset.

Multimodal Mode
Read More: Statistics Formula
Application of Mode in Mathematics
[Click Here for Previous Year Questions]
The mode of a set of observations is the value that appears the most frequently. In other terms, the mode of data is the observation in a set of data with the highest frequency. There's a chance that a data set will have more than one mode if there are many observations with the same frequency. The data set is said to be multimodal in this scenario.
Suppose, for example: 3, 7, 5, 13, 20, 23, 39, 23, 40, 23, 14, 12, 56, 23, 29
Putting these numbers in order- 3, 5, 7, 12, 13, 14, 20, 23, 23, 23, 23, 29, 39, 40, 56
Here we find that number 23 appears the most. Hence, the mode will be 23.
Read More: Measures of Central Tendency
Formula of Mode
[Click Here for Sample Questions]
It is not possible to calculate the mode of a clustered frequency distribution simply by looking at the frequency. The modal class is used to determine the data mode in such cases. The modal class contains the mode property. The following formula is used to determine the data mode:
Mode = l + \(\frac {(f_1 - f_0)} {(2f_1- f_0- f_2)}\) h
- Here, l is the modal class's lowest limit
- h denotes the length of the class interval.
- f1 denotes the modal class's frequency.
- f0 denotes the frequency of the class before the modal class.
- f2 denotes the frequency of the class that follows the modal class.
Read More: Set Operations
How to Find Mode?
[Click Here for Previous Year Questions]
Example 1: Determine the mode of the data set of 4, 4, 4, 9, 15, 15, 15, 27, 37, 48.
Solution: The data set is as follows: 4, 4, 4, 9, 15, 15, 15, 27, 37, 48.
A data set or set of values can have more than one mode, as we know, if more than one value happens with similar frequency and number of times as the other values in the set.
As a result, the numbers 4 and 15 are both modes of the set in this case.
Example 2: Determine the data set's mode: 3, 3, 6, 9, 15, 15, 15, 27, 27, 37, 48.
Solution: In the following number sequence- 3, 3, 6, 9, 15, 15, 15, 27, 27, 37, 48,
The number 15 is the mode since it appears more frequently in the set than the other numbers.
Read More: Set Theory Symbols
How to Find Mode for Different Data Groups?
[Click Here for Sample Questions]
Ungrouped Data:
Ungrouped data is information that does not appear in groupings. Let's look at an example to see how to determine the mode of ungrouped data. Assume a clothing firm produced winter coats in the sizes listed in the frequency distribution table:
| Winter Coat Size | 38 | 39 | 40 | 42 | 43 | 44 | 45 |
| Total no. of coats | 33 | 11 | 22 | 55 | 44 | 11 | 22 |
The size 42 has the highest frequency, as can be shown. As a result, the standard size for winter coats is 42. However, this is not the case with aggregated data.
Grouped Data:
Follow the steps below to find the mode for aggregated data.
Step 1: Determine which class interval has the highest frequency. This is also referred to as a modal class.
Step 2: Determine the class size. The higher limit is subtracted from the lower limit to arrive at this figure.
Step 3: Using the mode formula, determine the mode:
Mode = L + h x \(\frac {(f_m - f_1)} {(f_m - f_1) + (f_m - f_2)}\)
Consider the example given below. The data below represents the results of a certain exam taken by students.
Let's see if we can find a mode for this:
10 - 15 is the modal class (This is the most often occurring class.) The modal class's lower limit = (L) = 10, and the modal class's frequency (fm) = 7, Frequency of the modal class before it (f1)= 3, the next modal class's frequency (f2) = 2 and size of the class interval (h) = 5,
By putting the above values in the formula mentioned earlier, the answer will be:
Mode= 10 + 5 \(\frac {(7 - 3)} {(7 - 3) + (7 - 2)}\)= 12.22
So, the mode will be 12.22.
Read More: Matrix Addition
Comparison between Mean, Median and Mode
[Click Here for Previous Year Questions]
A brief comparison between Mean, Median and Mode is tabulated below.
| Points | Mean | Median | Mode |
|---|---|---|---|
| Definition | The average value is the ratio of the sum of the values in a data collection to the total number of values. | When a set of values is organised in order, the median is the value in the middle. | A given set of values' mode is the value that appears the most frequently. |
| Examples | For example, if the values are 2,2,3,4,5, then, mean will be = (2+2+3+4+5)/5 = 3.2 | For example, if the values are 2,2,3,4,5, then, the median will be 3. | For example, if the values are 2,2,3,4,5, then, mode will be 2. |
Read More:
| Related Topics | ||
|---|---|---|
| Sample Size Formula | Sampling Error Formula | Population Mean Formula |
| Absolute Values | Interquartile Range | Chi-Square Test |
Things to Remember
- The mode value is not necessarily the same as the mean and/or median.
- The mode comes in handy while looking for categorical data.
- There can't be a mode for data without any repeated digits.
- Mode can also be determined for data sets without any numbers.
- When the numbers are sorted in ascending order, finding the mode is simple.
- The mode for ungrouped data can be discovered through observation, whereas the mode for grouped data can be discovered through the use of a formula.
- Formula of mode- Mode = l +\(\frac {(f_1 - f_0)} {(2f_1- f_0- f_2)}\) h
Previous Year Questions
- Mean of n observations…..[WBJEE 2017]
- Standard Deviation of n observations…..[WBJEE 2016]
- Let [ ?] denote the greatest integer function and…...[COMEDK UGET 2011]
- The mean of the data set comprising of 16 observations is 16. If one of the observation d…..[JEE Main 2015]
- A student scores the following marks in five tests …...[JEE Main 2019]
- All the students of a class performed poorly in Mathematics. The teacher decided to give grace marks of….[JEE Main 2013]
- The mean and variance of 20 observations are found to be 10 and 4….[JEE Main 2020]
- If the sum of the deviations of 5050 observations from 30 is 50…..[JEE Main 2019]
- Consider the following statements : (1) Measures of dispersion Range,Quartile deviation…..
- Which of the following statements is/are true?…..
Get Live Updates on Board Exams 2023: Telegram Channel
Sample Questions
Ques: Calculate the modal value for the data in the above table. (2 Marks)
![]()
Ans: On arranging the given data in ascending form, 85, 86, 86, 88, 88, 90, 91, 92, 95, we found that it is bi-modal data since there are two repeating values.
As a result, for a particular collection of data, the modal values are 86 and 88.
Ques: Determine the mode of the following marks achieved by 25 students in a mathematics test out of a total of 50. (2 Marks)
45, 39, 43, 22, 27, 37, 46, 35, 34, 39, 40, 30, 30, 41, 37, 46, 39, 29, 34, 39, 35, 43, 30, 34, 46
Ans: By arranging the numbers in the ascending form- 22, 27, 29, 30, 30, 30, 34, 34, 34, 35, 35, 37, 37, 39, 39, 39, 39, 40, 41, 43, 43, 45, 46, 46, 46.
We found that the number 39 appears the most frequently. As a result, the mode is 39.
Ques: For the following data, calculate mode: (2 Marks)

Ans: Class 30-40 is the modal class since its frequency is the highest. Pre-modal and post-modal classes are 20-30 and 40-50, respectively. The mode is as follows:
Mode= 30 + 10×[(15-10)/(2×15-10-10)]
= 30+ 5= 35 (Ans.)
Ques: The table below displays the outcome of tossing three coins 40 times in a row. The total number of heads that appeared was counted. Find out mode. (2 Marks)
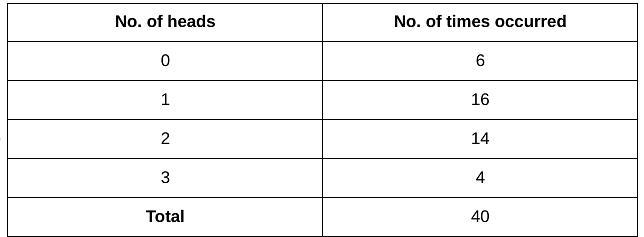
Ans: When looking at the data set, the number 1 appears more frequently. That is a total of 16 times.
As a result, the mode = 1 (Ans.)
Ques: The frequency table below shows how many text messages 50 fifteen-year-olds send in a day. (2 Marks)

Ans: The number 8 appears a greater number of times in the data collection. That is a total of 13 times.
As a result, the mode = 8
Ques: Determine the mode of the frequency distribution below. (3 Marks)

Ans: Based on the information in the table,
20 is the highest frequency.
This number falls between 50-60. As a result, it's the model class.
Model class ranges from 50-60.
l = Modal class's lower limit = 50
h = Class interval size (assuming all classes are the same size) = 10
20 = Frequency of the modal class f1
f0 = Class frequency preceding the modal class=12
f2 = Frequency of the subsequent class after the modal class=11
So,
mode = l x (f1 - f0 / 2f1-f0-f2) x h
= 50 x ( 20-12 / 2x20-12-11)x 10
= 54.706 (Ans.)
Ques: Sam is a duck breeder. The number of ducklings that survived after one month for each pair is recorded in the table. (3 Marks)

Ans: The number 5 appears a greater number of times in the data collection. That's a 30 times increase.
As a result, the mode is set to 5.
FAQs Based on Mode
Ques: What is mode in statistics? (1 Mark)
Ans: In statistics, a mode is defined as the value with the highest frequency among a set of values. It's the value that shows up the most frequently.
Ques: Is it possible for a set of data to have two modes? (1 Mark)
Ans: Yes, a given collection of data can have two modes. Bimodal values are those that have two extremes.
Ques: What does it mean to have a "no mode condition"? (1 Mark)
Ans: It is considered to be no mode if the supplied collection of observations has no value that is repeated in the set more than once.
Ques. Is it possible for the number 0 to be a mode? (1 Mark)
Ans: Yes, if the number 0 appears more than once in a set of data, it can be considered a mode.
Ques: If there are no repeating numbers, what is the mode? (1 Mark)
Ans: If the list contains no repeated numbers, it signifies that no number can be classified as a mode. In such circumstances, zero modes for the supplied data set are discovered. As a result, no modes are discovered.
Ques: Is the Mode Formula the same for both grouped and ungrouped data? (1 Mark)
Ans: No, the mode formula for grouped and ungrouped data is not the same.
Ques: What is the difference between a trimodal and a multimodal mode? (1 Mark)
Ans: When there are three modes in a data collection, it is referred to as trimodal, and when there are four or more, it is referred to as multimodal.
Ques: What are the Benefits of Using Mode in Math? (2 Marks)
Ans: The following are some of the benefits of mode in mathematics:
- It's straightforward to comprehend and calculate.
- For the open end frequency distribution, it is simple to calculate.
- It is a widely used method for determining average variables such as average student grades, average labour earnings, and so on.
- It's also available in graphic form.
- Extreme values will never have an impact on it. As a result, it is a good data representation.
Ques: What are the negative outcomes of Mode?(2 Marks)
Ans. The following are some of the downsides of mode:
- In the case of the multimodal series, it is difficult to characterise.
- In comparison to the mean and median, the value of mode is most affected by sample variation.
- For statistical analysis and algebraic calculations, mode is not appropriate.
- As with mean, modal value cannot be used to find the sum of the entire series.
- Ill-defined, ill-definite, and indeterminate are all terms used to describe mode.
Ques: Students' ages are 14,15,16,15,17,15,18. Using the mode formula in statistics, determine the mode of the given data set. (2 Marks)
Ans: To determine the Mode of a data set, i.e. {14,15,16,15,17,15,18}
Using the mode formula, we can see that just one value is repeated. As a result, it's a unimodal list.
15 is the most common mode.
Check-Out:





Comments