
Content Writer
Factorial is an integral function in Mathematics that is used to find out the number of ways in which things can be arranged. Factorial of a natural number 'n' is the product of that number with every natural number less than or equal to 'n' till 1.
- Factorial is defined as a function that multiplies a number by every number below it till 1.
- The concept of Factorial was proposed by Daniel Bernoulli.
- It is used in numerous mathematical fields such as Probability, Sequences and Series, Permutations and Combinations, etc.
Example: Factorial of 5 represents the multiplication of numbers 5, 4, 3, 2, 1, i.e. 5! = 5 x 4 x 3 × 2 × 1 and is equal to 120.
Read More: NCERT Solutions for Class 11 Mathematics Permutations and Combinations
Key Terms: Factorial, Factorial Formula, Probability, Permutations and Combinations, Arrangement, Factorials Table, Natural Numbers
What is Factorial?
[Click Here for Sample Questions]
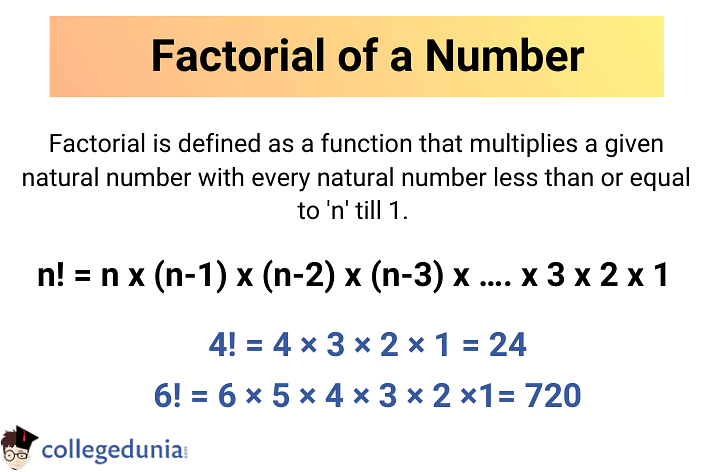
Factorial is defined as a function that multiplies a given natural number with every natural number less than or equal to 'n' till 1.
- It is a multiplication operation of a number with all the natural numbers that are less than it.
- A factorial is represented by an exclamation mark.
- It is symbolically represented as a "!".
- Thus, the product of the first n natural numbers is referred to as factorial and is denoted as ‘n!’.
What is Factorial?
Hence, it can be said “n!” or "n factorial" means:
| n! = 1 x 2 x 3 ……. n = Product of First n Positive Integers = n (n-1) (n-2) ……………… (3) (2) (1) |
Factorial Notation
- Factorial is the multiplication of all positive integers, “n”, that will be smaller than or equivalent to n.
- Factorial of a positive integer is denoted by the symbol “n!”.
Permutations and Combinations Detailed Video Explanation
Factorial Example
Example: Consider the example of Factorial of 4.
Factorial of 4 or 4! will be
4! = 4 × 3 × 2 × 1 = 24
Some numbers and their factorial values are given in the table below.
| n | Factorial of a Number n! | Expansion | Value |
|---|---|---|---|
| 1 | 1! | 1 | 1 |
| 2 | 2! | 2 × 1 | 2 |
| 3 | 3! | 3 × 2 × 1 | 6 |
| 4 | 4! | 4 × 3 × 2 × 1 | 24 |
| 5 | 5! | 5 × 4 × 3 × 2 × 1 | 120 |
Factorial Formula
[Click Here for Previous Years’ Questions]
Factorial Formula is used to find the factorial of a given natural number. Factorial Formula is given as:
| n! = n x (n-1) x (n-2) x (n-3) x …. x 3 x 2 x 1 |
It can be simplified and written as:
| n! = n x (n−1)! |
Thus, the recurrence relation for the factorial of a number is the product of the factorial number and the factorial of that number minus 1.
Solved ExampleExample: Find the factorial of 6. Solution: Factorial Formula is given as: n! = n × (n – 1) × (n – 2) × (n – 3) × ….× 3 × 2 × 1 Substitute the value of 6, 6! = 6 × (6 -1) × (6 – 2) × (6 – 3) × (6 – 4) × 1 6! = 6 × 5 × 4 × 3 × 2 ×1 6! = 720 Thus, the factorial of 6 is 720. |
Factorial Solved Examples
[Click Here for Sample Questions]
Here are some solved examples on Factorials for better understanding:
Example 1: A group of 10 will be awarded $ 200, $ 100, and $ 50 in prize money. How can prizes be awarded?
Solution: It is a case of Permutation because the order is important here. It can be calculated as 10P3 Forms.
Using the Permutation Formula:
10P3 = 10!/(10-3)! = 10!/7! = 10987!/7! = 720 Ways
Example 2: A group of 10 will be awarded three $ 50 prizes. How can prizes be awarded?
Solution: It is a case of Combination, as the order is not important here. It can be calculated in 10C3 ways.
Using the Combination Formula:
10C3 = 10!/3!(10-3)! = 10!/3!7! = 10987!/3217! = 120 Forms.
Read More:
Factorial of Numbers 1 to 10 Table
[Click Here for Previous Years’ Questions]
Factorial of n is indicated by ‘n!’. It is calculated by multiplying the given integer from 1 to n. Factorial Formula is n! = n(n-1)!. Factorial Table for the factorials of the first 15 natural numbers is given below:
| n | Factorial of Number n! | n! |
|---|---|---|
| 1 | 1! | 1 |
| 2 | 2! | 2 |
| 3 | 3! | 6 |
| 4 | 4! | 24 |
| 5 | 5! | 120 |
| 6 | 6! | 720 |
| 7 | 7! | 5040 |
| 8 | 8! | 40,320 |
| 9 | 9! | 362,880 |
| 10 | 10! | 3,628,800 |
| 11 | 11! | 39,916,800 |
| 12 | 12! | 479,001,600 |
| 13 | 13! | 6,227,020,800 |
| 14 | 14! | 8,717,8291,200 |
| 15 | 15! | 1,307,674,368,000 |
Given below is a detailed explanation of the Factorial of a few numbers:
Factorial of 5
Factorial of 5 can be found easily with the help of the Factorial Formula.
Using the Factorial Formula,
n! = 1 × 2 × 3 …… × n
Factorial of 5 will be calculated as:
5! = 1 × 2 × 3 × 4 × 5
5! = 120
Thus, the value of the factorial of 5 is 120.
Factorial of 10
Factorial of 10 can also be calculated using Factorial Formula.
Using the Factorial Formula,
n! = 1 × 2 × 3 …… × n
10! = 10 × 9 × 8 × 7 × 6 × 5 ×4 × 3 × 2 × 1
10! = 3,628,800
Thus, the value of the factorial of 10 factorial is 3,628,800.
Read More: Important Questions For Class 11 Maths Permutations and Combinations
Factorial of 0
The value of the Factorial of 0 is equal to 1, i.e. 0! = 1. Thus, the value of the factorial of zero is not zero but 1. The explanation of the same is as follows:
- 1! = 1
- 2! = 2 × 1 = 2
- 3! = 3 × 2 × 1 = 3 × 2! = 6
- 4! = 4 × 3 × 2 × 1 = 4 × 3! = 24
Factorial Formula is given as n! = n × (n - 1)!
- In order to find 3!, we do 4! / 4.
- Similarly, 2! is given as 3! / 3, and so on.
- 1! is given as 2! / 2.
- Thus, 0! is given as 1! / 1, which is equal to 1.
Factorial of 100
Factorial of 100 is given as
100! = 100 × 99 × 98 × .... × 3 × 2 × 1 = 9.332621544 E+157
The value of the factorial of 100 is too big to calculate manually and thus, a calculator is used.
- Factorial of 100 has 24 trailing zeros in it.
- There are a total of 158 digits in 100!.
- The value of factorial of 100 is 93, 326, 215, 443, 944, 152, 681, 699, 238, 856, 266, 700, 490, 715, 968, 264, 381, 621, 468, 592, 963, 895, 217, 599, 993, 229, 915, 608, 941, 463, 976, 156, 518, 286, 253, 697, 920, 827, 223, 758, 251, 185, 210, 916, 864, 000, 000, 000, 000, 000, 000, 000, 000.
Factorial of Negative Numbers
[Click Here for Sample Questions]
Factorial for negative numbers is not defined. In order to prove the same, we will begin with the factorial of 3.
- 3! = 3 x 2 x 1 = 6
- 2! = 3!/3 = 6/3 = 2
- 1! = 2!/2 = 2/2 = 1
- 0! = 1!/1 = 1/1 = 1
- (-1)! = 0!/0 = 1/0
Dividing by zero is undefined, thus, the factorials of negative numbers are not defined.
What is Sub Factorial of a Number?
Sub-Factorial of a Number, defined by the term “!n”, is the number of rearrangements of n objects. It denotes that the number of permutations of n objects so that no object stands in its original position. Sub-factorial of a number is calculated by the formula:
| \(!n = n!\sum_{k=0}^{n}\frac{(-1)^{k}}{k!}\) |
Uses of Factorials
[Click Here for Previous Years’ Questions]
Factorials are commonly used in Permutations and Combinations.
Permutation is defined as an ordered arrangement of elements and is calculated using the formula:
| \({^nP_r} = \frac{n!}{(n-r)!} \) |
Combination is defined as the grouping of elements in which order does not matter. Combination Formula is given as:
| \({^nC_r} = \frac{n!}{r!(n-r)!} \) |
In both Permutations and Combinations Formulas, 'n' is the total number of things available, and 'r' is the number of things that have to be chosen.
Check More:
Things to Remember
- Factorial is the product of a given number with every natural number less than or equal to it till 1.
- It is denoted by the symbol ‘n!’, where n is the given number.
- Factorial Formula is given as n! = n × (n - 1)!.
- The value of factorial of zero is 1, that is 0! = 1.
- Factorials of Negative Numbers are not defined.
- Factorials are majorly used in Permutations and Combinations Formulas.
For Latest Updates on Upcoming Board Exams, Click Here: https://t.me/class_10_12_board_updates
Previous Years’ Questions
- Consider three boxes, each containing 10 balls labeled 1,2,....,10. Suppose one ball is…? [JEE 2019]
- If in a regular polygon the number of diagonals is 54, then the number of sides of this polygon is…? [JEE 2015]
- If the four letter words (need not be meaningful ) are to be formed using the letters from the word…? [JEE 2016]
- In a collage of 300 students, every student reads 5 newspapers and every newspaper…? [JEE 1998]
- Number of divisors of the form (4n+2),n≥0 of the integer 240 is…? [JEE 1998]
- The number of 4 digit numbers without repetition that can be formed using the digits…? [KCET 2020]
- If nC12 =nC8 then n is equal to…? [KCET 2017]
- How many 5 digit telephone numbers can be constructed using the digits 0 to 9, if each number…? [KCET 2014]
- There are 10 persons including 3 ladies. A committee of 4 persons including at least one…? [KEAM]
- If nCr−1=28, nCr=56 and nCr+1=70, then the value of r is equal to…? [KEAM]
- The number of words that can be formed by using all the letters of the word PROBLEM only one is…? [KEAM]
- How many four digit numbers abcd exist such that a is odd, b is divisible by 3, c is even…? [KEAM]
- Let Tn be the number of all possible triangles formed by joining vertices of an n-sided regular…? [JEE 2013]
- Let A and B be two sets containing four and two elements respectively. Then the number of subsets…? [JEE 2015]
- If all the words (with or without meaning) having five letters, formed using the letters…? [JEE 2016]
Sample Questions
Ques. What is Factorial Notation? (2 Marks)
Ans. Factorial Notation is an extended form of factorial for numbers.
Example: Factorial Notation of 3 and 5 is as follows:
- 3! = 3 x 2 x 1
- 5! = 5 x 4 x 3 x 2 x 1
Ques. What is the value of the given expression involving factorials: 10!/(4! × 6!)? (3 Marks)
Ans. On solving the given expression,
10!/(4! × 6!) = (10 × 9 × 8 × 7 × 6!) / (4! × 6!)
= (10 × 9 × 8 × 7) / (4 × 3 × 2 × 1)
= 210
Thus, the value of 10!/(4! × 6!) is 210.
Answer: Therefore, the value of the expression 10!/(4! × 6!) is 210.
Ques. What is (1 + n) Factorial? (1 Mark)
Ans. (n+1) factorial can be calculated as (n+1)! = (n+1) n!.
Ques. What is the Factorial of 10? (2 Marks)
Ans. Factorial of 10 is calculated as:
10! = 10 × 9 × 8 × 7 × 6 × 5 ×4 × 3 × 2 × 1
10! = 3,628,800
Thus, the value of the factorial of 10 factorial is calculated as 3,628,800.
Ques. What is Factorial? (3 Marks)
Ans. Factorial is defined as a multiplication operation of natural numbers with all the natural numbers that are less than it. It is the product of a natural number with every whole number less than or equal to 'n' till 1.
n! = n × (n-1) × (n-2) × (n-3) × ..... × 3 × 2 × 1
Example: Factorial of 4 is given as
4! = 4 × 3 × 2 ×1 = 24
Ques. What is the relationship between Factorial, Permutation, and Combination? (3 Marks)
Ans. Factorial is a function used to find the number of ways in which a selected number of objects can be placed on each other. Factorial is used to find permutations and combinations of numbers and events. It is used in Permutations and Combinations Formulas.
- Factorial in Permutation Formula is used as: \({^nP_r} = \frac{n!}{(n-r)!} \)
- Factorial in Combination Formula is used as: \({^nC_r} = \frac{n!}{r!(n-r)!} \)
Ques. What is Factorial Sign? (1 Mark)
Ans. The symbol used to represent the Factorial of a number is ‘!’. For example, the factorial of 5 is expressed as 5!.
Ques. What is the value of 5! (6 - 3)!? (3 Marks)
Ans. On simplification, 5! (6−3)! = 5! × 3!
= (5 × 4 × 3 × 2 × 1)(3 × 2 × 1)
= 120 × 6
= 720
Thus, the value of 5! (6 - 3)! is 720.
Ques. What are Factorials used for? (5 Marks)
Ans. Factorials are used to find the number of patterns, solve permutation and combination problems, find the probability of an event, and more. Some applications of factorials in mathematics are:
Recursion
In the recursive definition of a number, factorials are used. A number can be expressed in an expression containing the number only.
p! = p×(p–1) × (p–2) × (p–3)….(p−(p–2)) × (p–(p–1))
Permutations
It is the arrangement of given r things out of total n things when order is strictly important.
\({^nP_r} = \frac{n!}{(n-r)!} \)
Combinations
It is the arrangement of given r things out of total n things when order is not important.
\({^nC_r} = \frac{n!}{r!(n-r)!} \)
Probability Distributions
There are various probability distributions like binomial distribution which include the use of factorial. To find the probability of an event, the concept of permutations and combinations is used a lot.
Ques. What is the Value of 7!? (2 Marks)
Ans. 7! is the factorial of 7. Its value is calculated as:
7! = 7 × 6 × 5 × 4 × 3 × 2 × 1 = 5040
Thus, the value of 7! is 5040.
Check Out:





Comments