
Content Curator
You must have learned several other concepts and skills before you can calculate the angle between two vectors. Before proceeding to know how to find the angle between the two vectors, please ensure that you are familiar with the following material. As a side note, this article employs two vector notations. A vector is a letter that is bold or has an arrow above it. These two symbols, for example, both denote the vector w.
Also Read: NCERT Solutions For Class 12 Mathematics Chapter 10 Vector Algebra
| Table of Content |
Key Terms: Angles between two Vectors, Vectors, Magnitude, trigonometric function, Unit vector, angle equation, Triangle, Angle
Importance of Angles between two Vectors
[Click Here for Sample Questions]
For a variety of reasons, we need to know how to calculate the angle between two vectors. We are surrounded by vectors. The forces acting on beams and other structural supports are called vectors. For predicting weather patterns and climates, vectors are used to represent wind, pressure, humidity, and a variety of other conditions.
Read More: Addition of Vectors
Vectors are used to model the air that flows around an aircraft's wing, the fluid that flows through a pipe, and a variety of other situations. These vectors aid researchers in the development of fuel-efficient aircraft and high-pressure pipes.
What is Vector?
[Click Here for Sample Questions]
Vector is a physical quantity that possesses both magnitude and direction i.e a number and the way of direction. Vectors are oriented in various directions and form various angles. This angle exists between two vectors and is responsible for defining vector erection.
Also Read: magnitude of the vector
For example, if we consider the motion of a tennis ball, its position is described by a position vector and its movement by a velocity vector, the length of which indicates the ball's speed. The vector's direction explains the motion's direction. Similarly, the momentum of the ball is an example of a vector quantity that is mass times velocity.

Read More: Types of Vector
Angle Equation
[Click Here for Sample Questions]
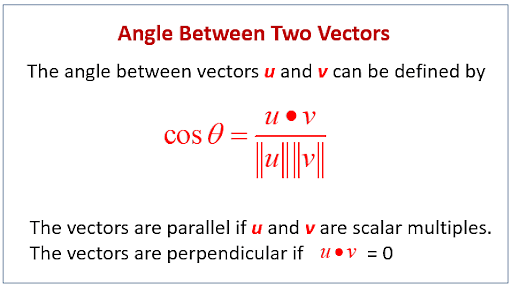
This is the equation for determining the angle between two vectors, u and v.
\(cos \theta = \frac{\overrightarrow{u}.\overrightarrow{v}}{|\overrightarrow{u}||\overrightarrow{v}|}\)
This isn't a simple equation. A trigonometric function - the dot product of two vectors, and the magnitude of two vectors are all involved in this equation.
The following examples will show you how to use the equation to find theta (θ) or the angle between two vectors.
Also Read: Real-Valued Function
- Let us assume two vectors, u and v, in order to determine the angle (in degrees) between them.Example:
\(\vec {u}\) = <_3,4>
\(\vec {v}\)= <5,12>
The dot product of the two vectors is required by the equation,
\(\vec{u} \bullet \vec{v}\)= -3(5) + 4(12)
= -15 + 48
= 33
The magnitudes of the vectors can be calculated as part of the equation, so here they are.
|\(\overrightarrow{u} \)| = \(\sqrt{(-3)^2 + (4)^2} = \sqrt{9 + 16} = \sqrt{25} = 5\)
|\(\overrightarrow{v} \)| = \(\sqrt{(5)^2 + (12)^2} = \sqrt{25 + 144} = \sqrt{169} = 13\)
Now, put this information into the equation as follows:
\(cos \theta = \frac{\overrightarrow{u}.\overrightarrow{v}}{|\overrightarrow{u}||\overrightarrow{v}|}\)
cos θ = \(\frac{33}{5(13)}\)
cos θ = \(\frac{33}{65}\)
Now, use the inverse cosine or arccosine to solve for the angle, theta.
θ = cos-1(\(\frac{33}{65}\))
θ ≈ 59.490°
Thus, the angle between two vectors is.
Also Read:
Dot Product
[Click Here for Sample Questions]
The dot product of vectors u and v is written as follows :
\(\vec{u} \bullet \vec{v}\)
This may appear to be multiplication, but in the world of vectors, it is known as a 'dot product.'

Find the dot product of two vectors:
If, u = <uh , uv> and v = <vh , vv> then add the products of their respective components, as shown below:
\(\vec{u} \bullet \vec{v}\) = uh . vh + uv . vv
A scalar quantity will be obtained as a result. To put it in other words, the outcome is a number.
Example:
- Find the dot product of vectors a and b. If a = <4, -2> and b = <-3,-1>
\(\vec {a} \bullet \vec {b}\)= 4(-3) + (-2)(-1) = (-12) + 2
\(\vec {a} \bullet \vec {b}\)= -10
Solution : There are two methods for calculating the dot product of two vectors. They are
Method One: \(\vec{u} \bullet \vec{v}\)= uh . vh + uv . vv
Method number two: \(\vec{u} \bullet \vec{v}\)= |\(\vec{u}\)| |\(\vec{v}\)| cosθ
where is the angle formed by the two vectors.
Read More: Negative of a Vector
The Angle between two 2D Vectors
[Click Here for Sample Questions]
- Vectors specified by coordinates: vectors a = [xa, ya] , b = [xb, yb] angle = arccos[(xa * xb + ya * yb) / (√(xa2 + ya2) * √(xb2 + yb2))]
- Vectors included in a starting and terminal point:
For vector p: P= [x1, y1] , B = [x2, y2],
Thus, vector P = [x2 - x1, y2 - y1]
For vector q: Q = [x3, y3] , D = [x4, y4],
so vector R = [x4 - x3, y4 - y3]
Next, put the the derived vector coordinates into the angle between two vectors formula for coordinate from pointer 1: we get,
angle = arccos[((x2 - x1) * (x4 - x3) + (y2 - y1) * (y4 - y3)) / (√((x2 - x1)2 + (y2 - y1)2) * √((x4 - x3)2 + (y4 - y3)2))]
Also Read: Horizontal and Vertical Lines
-
The Angle between two 3D Vectors
Similarly, the angle between 3D vectors is given by,
angle = arccos{[(x2 - x1) * (x4 - x3) + (y2 - y1) * (y4 - y3) + (z2 - z1) * (z4 - z3)] / [√((x2 - x1)2 + (y2 - y1)2+ (z2 - z1)2) * √((x4 - x3)2 + (y4 - y3)2 + (z4 - z3)2)]}
Also Read: Straight Lines
-
Unit Vector
A unit vector is a vector having 1 magnitude. Magnitude is a term that refers to the length of a vector. As a result, any vector with length one is a unit vector.
Symbolically, it is written as follows: |v| represents the magnitude of v. As a result, if |v| = 1, v is a unit vector.
For example, \(\hat {i}\) is a one-length vector that figures to the right on a Cartesian plane.
It is written as:- \(\hat {i}\) = <1,0>
How to calculate a unit vector?
A unit vector calculation involves a non-zero vector and dividing it by its magnitude.
\(\hat {u} = \frac { \vec{u}} {|u|}\)
Also Read: Sin 30 Degrees
Determine the Angle Between Two Vectors Using the Cross Product
[Click Here for Sample Questions]
The cross product is another method for calculating the angle between two vectors.
The right-hand rule gives the vector that is perpendicular to both vectors and directions. As a result, the cross product is mathematically represented as,
a * b = |a| |b| . sin (θ) n
-
Example:
Consider two vectors, a and b, whose tails are joined and thus form some angle. We will use the above-mentioned cross-product formula to calculate the angle between two vectors.
(a * b) / (|a|.|b|) = sin (θ)
If the given vectors a and b are parallel to each other, the cross product will be zero because sin (0) = 0. Thus it is important to be cautious when dealing with the cross-product directions.
Read More: vector product of two vectors
Things to Remember
- Vectors find their application in science to describe anything that possesses both a direction and a magnitude.
- A unit vector is the product of a vector and its magnitude.
- The coordinates of the zero vector are (0,0,0), and it is typically represented by 0 with an arrow (→)at the top or simply 0.
- Commutative Law states that the order of addition is irrelevant, i.e., a + b = b + a.
- Associative law states that the sum of three vectors has no bearing on which pair of vectors is added first. That is, (p + q) + r = p + (q + r).
Also Read: multiplication of a vector by a scalar
Sample Questions
Ques. Find the Angle between the Given Two Vectors 4i + 5j – k and 2i – j + k. (3 marks)
Ans: Solution:
Let, a = 4i + 5j – k and b = 2i – j + k
So, the dot product is,
a.b = (4i + 5j – k).(2i – j + k)
= (4)(2) + (5)(-1) + (-1)(1)
= 8 - 5 - 1
= 2
Ques. When are two vectors pointing in the same direction? (2 marks)
Ans: When two vectors are perpendicular to one another, the cross product formula is:
- If both vectors are parallel or opposite to each other, the cross product is zero vectors.
- In the opposite case, if two vectors are parallel or opposite to each other, their product is a zero vector. The sense of direction of the two vectors is the same.
Ques. Find the Angle between the Given Two Vectors 5i + 5j – k and 3i – 2j + k. (3 marks)
Ans: Let, a = 5i + 5j – k and b = 3i – 2j + k
So, the dot product is,
a.b = (5i + 5j – k).(3i – 2j + k)
= (5)(3) + (5)(2) + (-1)(1)
= 15 + 10 - 1
= 24
Ques. What is the Triangle Law of Vector Addition, and how does it work? (2 marks)
Ans: When two vectors are signified as two sides of a triangle with the same order of magnitude and direction, the magnitude and direction of the resultant vector are represented by the third side of the triangle.
In order to obtain, R, which is the sum of vectors \(\vec{A}\) and \(\vec{B}\)having the same order of magnitude and direction
Thus, the Resultant vector is given by,
\(\vec{R}\) = \(\vec{A}\)+\(\vec{B}\)
Ques. Compute the angle between two vectors given that they are unit vectors p and q where p x q = 1 / 3i + 1 / 4j. (3 marks)
Ans: |a| = |b| = 1
Where,
| a x b | = √ ( (1 / 3)^2 + ( 1 / 4)^2) = 1 / 5
Put the given values in the above formula, we get
| a x b | = |a| |b| sin θ
1 / 5 = (1) (1) sin θ
θ = sin-1 (1/ 5)
θ = 30º
For Latest Updates on Upcoming Board Exams, Click Here: https://t.me/class_10_12_board_updates
Also Read:





Comments