Choose the option showing the correct relation between Poisson’s ratio (σ), Bulk modulus (B) and modulus of rigidity (G).
\(\sigma=\frac{3B-2G}{2G+6B}\)
\(\sigma=\frac{6B+2G}{3B-2G}\)
\(\sigma=\frac{9BG}{3B+G}\)
\(B=\frac{3\sigma-3G}{6\sigma+2G}\)
The Correct Option is A
Solution and Explanation
E = 2G(1 + σ) ….(1)
E = 3B(1 – 2σ) ….(2)
\(1=\frac{2G}{3G}(\frac{1+\sigma}{1-2\sigma})\)
⇒ 3B – 6Bσ = 2G + 2Gσ
⇒ 3B – 2G = σ (2G + 6B)
\(\sigma=(\frac{3B-2G}{2G+6B})\)
So, the correct answer is (A) : \(\sigma=\frac{3B-2G}{2G+6B}\)
Top Questions on Elastic and inelastic collisions
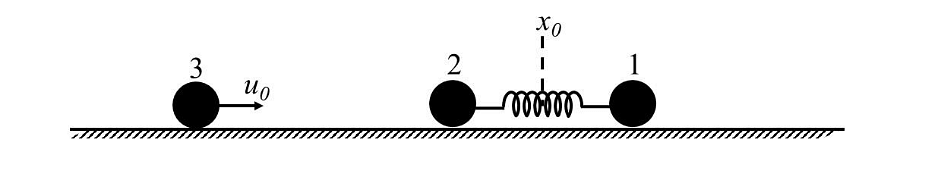
- Two particles, 1 and 2, each of mass 𝑚, are connected by a massless spring, and are on a horizontal frictionless plane, as shown in the figure. Initially, the two particles, with their center of mass at \(𝑥_0\), are oscillating with amplitude 𝑎 and angular frequency 𝜔. Thus, their positions at time 𝑡 are given by \(x_1 (t) = (x_0 + d) + \alpha \ sin \omega t\) and \(x_2t = (x_0 -d) − \alpha sin \ wt,\) respectively, where \(𝑑 > 2𝑎.\) Particle 3 of mass 𝑚 moves towards this system with speed 𝑢0 = 𝑎𝜔/2, and undergoes instantaneous elastic collision with particle 2, at time \(𝑡_0\). Finally, particles 1 and 2 acquire a center of mass speed \(𝑣_{cm}\) and oscillate with amplitude 𝑏 and the same angular frequency 𝜔.
- JEE Advanced - 2024
- Physics
- Elastic and inelastic collisions
- A uniform heavy rod of mass \(20\) kg, cross sectional area \(0.4\) \(m^2\) and length \(20\) \(m\) is hanging from a fixed support. Neglecting the lateral contraction, the elongation in the rod due to its own weight is \(x × 10^{–9}\) \(m\). The value of \(x\) is _____(Given Young’s modulus \(Y = 2 × 10^{11} Nm^{–2}\)and g = \(10\; ms^{–2})\)
- JEE Main - 2022
- Physics
- Elastic and inelastic collisions
- Which one of the following is the correct statement?
- KEAM - 2022
- Physics
- Elastic and inelastic collisions
- A ball hits the floor and rebounds after an inelastic collision. In this case
- KCET - 2021
- Physics
- Elastic and inelastic collisions
- A 1 kg ball moving at 12 ms-1 collides with a 2 kg ball moving in opposite direction at 24 ms-1. If the coefficient of restitution is \(\frac{2}{3}\), then the velocities after the collision are
- KCET - 2021
- Physics
- Elastic and inelastic collisions
Questions Asked in JEE Main exam
- Find the acceleration of \(2\) \(kg\) block shown in the diagram (neglect friction)

- JEE Main - 2024
- Acceleration
- If \(|2A|^3 =21\) and \(\begin{bmatrix} 1 & 0 & 0 \\[0.3em] 0 & α & β \\[0.3em] 0 & β & α \end{bmatrix}\), then a is (if \(α,β∈I\))
- JEE Main - 2024
- Matrices
- Let \(α\) and \(β\) the roots of equation \(px^2 + qx - r = 0\), where \(P≠ 0\). If \(p,q,r\) be the consecutive term of non constant G.P and \(\frac{1}{α} + \frac{1}{β} = \frac{3}{4}\) then the value of \((α - β)^2\) is:
- JEE Main - 2024
- Geometric Progression
- Two lines \(L_1 \;\& \;L_2\) passing through origin trisecting the line segment intercepted by the line \(4x + 5y = 20\) between the coordinate axes. Then the tangent of angle between the lines \(L_1\) and \(L_2\) is
- JEE Main - 2024
- Tangents and Normals
- Rank of the word 'GTWENTY' in dictionary is _____ .
- JEE Main - 2024
- permutations and combinations
Concepts Used:
Elastic and Inelastic Collisions
Elastic Collision:
According to elastic collision, the kinetic energy of the system will remain constant which means there will be no change in the kinetic energy of the system before and after the collision. It also goes along with the conservation of momentum.
Examples of Elastic Collision
- While playing billiards, balls hit each other.
- A ball is thrown and is bounced to the same height it was thrown from, is an example of elastic collision as there is no net change in the kinetic energy.
- The collision of atoms is also an elastic collision.
Inelastic Collision:
According to inelastic collision, the kinetic energy of the system is not conserved, unlike inelastic collision. The kinetic energy is lost as it gets debauched in other forms of energy like heat, sound, etc, or is absorbed by the body. But they go after the conservation of momentum, like an elastic collision.
Examples of Inelastic Collision
- When the ball is dropped on the ground, it fails to reach the height it was dropped from.
- The accident of two vehicles is an inelastic collision.
- If the clay is thrown at a wall, it sticks to it.
Read More: Elastic and Inelastic Collision



