At low pressure, the van der Waal's equation is reduced to
- $Z=\frac{PV_{m}}{RT}=1-\frac{a}{VRT}$
- $Z=\frac{PV_{m}}{RT}=1+\frac{b}{RT}P$
- $PV_{m}=RT$
- $Z=\frac{PV_{m}}{RT}=1-\frac{a}{RT}$
The Correct Option is A
Approach Solution - 1
$\left[P+\frac{a}{V^{2}}\right]\left(V-b\right)=RT$
or $PV=RT+Pb-\frac{a}{V}+\frac{ab}{V^{2}}$
or $\frac{PV}{RT}=1-\frac{a}{VRT}$
$Z=-\frac{a}{VRT}$
$\left(\because\frac{PV}{RT}-Z\right)$
Approach Solution -2
For ideal gases, Z=1 as PV = nRT.
Although, for real gasses, we may apply the Van der Waals equation i.e.
\([\frac{P+an^2}{V^2}]\) [V-nb] = nRT
Where,
P stands for pressure,
V means volume,
n is the number of moles
a is pressure correction constant
b is volume correction constant
T stands for temperature
R is for the universal gas constant
At the lower pressure, the volume correction constant or b becomes almost 0 or negligible and the equation is, therefore,
(P+\(\frac{a}{v^2}\))V = R.T
⇒\(\frac{PV}{RT}\)=(1-\(\frac{a}{RTV}\))=Z
Here,
Z is the compressibility factor.
Therefore, from the above calculation, we understand that Z = \(\frac{PvM}{RT}\) = 1-\(\frac{a}{RTV}\) and the correct answer is option (A).
Top Questions on Van Der Waals equation
- Molar volume ($ V_m $) of a van der Waals gas can be calculated by expressing the van der Waals equation as a cubic equation with $ V_m $ as the variable. The ratio (in mol dm$^{-3}$) of the coefficient of $ V_m^2 $ to the coefficient of $ V_m $ for a gas having van der Waals constants $ a = 6.0 \, \text{dm}^6 \, \text{atm mol}^{-2} $ and $ b = 0.060 \, \text{dm}^3 \, \text{mol}^{-1} $ at 300 K and 300 atm is ____. Use: Universal gas constant $ R = 0.082 \, \text{dm}^3 \, \text{atm mol}^{-1} \, \text{K}^{-1} $
- JEE Advanced - 2025
- Chemistry
- Van Der Waals equation
- Arrange the following gases in increasing order of van der Waals constant 'a'
A. Ar
B. CH4
C. H₂O
D. C6H6
Choose the correct option from the following.- JEE Main - 2023
- Chemistry
- Van Der Waals equation
- Which of the following statemnt is incorect for physisorption?
- GUJCET - 2023
- Chemistry
- Van Der Waals equation
- If $V$ is the volume of one molecule of gas under given conditions, the van der Waal?s constant $b$ is
- BITSAT - 2018
- Chemistry
- Van Der Waals equation
- Given van der Waals constant for NH3, H2, O2 and CO2 are respectively 4.17, 0.244, 1.36 and 3.59, which one of the following gases is most easily liquefied ?
- NEET (UG) - 2018
- Chemistry
- Van Der Waals equation
Questions Asked in VITEEE exam
- Find the value of \( x \) in the equation \( 4(x - 2) = 3(x + 5) \).
- VITEEE - 2025
- Linear Equations
- What is the pH of a solution if the concentration of \( \text{H}^+ \) ions is \( 1 \times 10^{-5} \) mol/L?
- VITEEE - 2025
- Solubility Equilibria Of Sparingly Soluble Salts
- A ball is dropped from a height of 20 m. What is its velocity just before hitting the ground? (Take \( g = 9.8 \, \text{m/s}^2 \))
- VITEEE - 2025
- Motion in a straight line
- A body is thrown vertically upwards with an initial velocity of \( 10 \, \text{m/s} \). How high will the body rise? (Take \( g = 10 \, \text{m/s}^2 \))
- VITEEE - 2025
- Motion in a straight line
- A capacitor is charged with a voltage of 100 V. If the capacitance of the capacitor is \( 10 \, \mu\text{F} \), what is the charge on the capacitor?
- VITEEE - 2025
- Electrostatics
Concepts Used:
Van Der Waals Equation
Van der Waals equation is an equation relating the relationship between the pressure, volume, temperature, and amount of real gases.
Read More: Derivation of Van Der Waals Equation
Derivation of Van der Waals equation:
For a real gas containing ‘n’ moles, the equation is written as
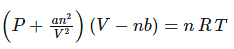
Where, P, V, T, n are the pressure, volume, temperature and moles of the gas. ‘a’ and ‘b’ constants specific to each gas.
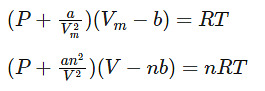
Where,
Vm: molar volume of the gas
R: universal gas constant
T: temperature
P: pressure
V: volume
Thus, Van der Waals equation can be reduced to ideal gas law as PVm = RT.
The equation can further be written as;
- Cube power of volume:
- Reduced equation (Law of corresponding states) in terms of critical constants:
Units of Van der Waals equation Constants
a: atm lit² mol-²
b: litre mol-¹