Select Goal &
City
Select Goal
Explore
Question:
If x3 - 2x2 - 9x + 18 = 0 and A = \(\begin{vmatrix} 1 & 2 & 3 \\ 4 & x & 6 \\ 7 & 8 & 9 \\ \end{vmatrix}\) then the maximum value of A is
If x3 - 2x2 - 9x + 18 = 0 and A = \(\begin{vmatrix} 1 & 2 & 3 \\ 4 & x & 6 \\ 7 & 8 & 9 \\ \end{vmatrix}\) then the maximum value of A is
Updated On: Aug 21, 2024
- 96
- 36
- 24
- 120
Hide Solution
Verified By Collegedunia
The Correct Option is A
Solution and Explanation
The correct answer is () :
Was this answer helpful?
2
1
Top Questions on Determinants
- If \[ f(x) = \begin{vmatrix} x^3 & 2x^2 + 1 & 1 + 3x \\ 3x^2 + 2 & 2x & x^3 + 6 \\ x^3 - x & 4 & x^2 - 2 \end{vmatrix} \] for all \( x \in \mathbb{R} \), then \( 2f(0) + f'(0) \) is equal to
- JEE Main - 2024
- Mathematics
- Determinants
- Let \( A \) be a \( 3 \times 3 \) matrix and \( \det(A) = 2 \). If \(n = \det(\text{adj}(\text{adj}(\ldots(\text{adj}(A))\ldots)))\) with adjoint applied 2024 times, then the remainder when \( n \) is divided by 9 is equal to \(\_\_\_\_\_.\)
- JEE Main - 2024
- Mathematics
- Determinants
- If \( \sin\left(\frac{y}{x}\right) = \log_e |x| + \frac{\alpha}{2} \) is the solution of the differential equation \[x \cos\left(\frac{y}{x}\right) \frac{dy}{dx} = y \cos\left(\frac{y}{x}\right) + x\]and \( y(1) = \frac{\pi}{3} \), then \( \alpha^2 \) is equal to
- JEE Main - 2024
- Mathematics
- Determinants
- If \[ f(x) = \begin{vmatrix} 2 \cos^4 x & 2 \sin^4 x & 3 + \sin^2 2x \\ 3 + 2 \cos^4 x & 2 \sin^4 x & \sin^2 2x \\ 2 \cos^4 x & 3 + 2 \sin^4 x & \sin^2 2x \end{vmatrix} \] then \( \frac{1}{5} f'(0) \) is equal to
- JEE Main - 2024
- Mathematics
- Determinants
- Let $A =\left[ a _{i j}\right], a _{i j} \in Z \cap[0,4], 1 \leq i, j \leq 2$ .
The number of matrices $A$ such that the sum of all entries is a prime number $p \in(2,13)$ is _____.- JEE Main - 2023
- Mathematics
- Determinants
View More Questions
Questions Asked in KCET exam
- The modulus of the complex number \(\frac{(1+i)^2(1+3i)}{(2-6i)(2-2i)}\) is
- KCET - 2023
- complex numbers
- The P-V diagram of a Carnot's engine is shown in the graph below. The engine uses 1 mole of an ideal gas as working substance. From the graph, the area enclosed by the P-V diagram is
[ The heat supplied to the gas is 8000 J ]
- KCET - 2023
- carnot cycle
- A point object is moving at a constant speed of 1 ms-1 along the principal axis of a convex lens of focal length 10cm. The speed of the image is also 1 ms-1 , when the object is at _______ cm from the optic centre of the lens.
- KCET - 2023
- spherical lenses
- A cubical Gaussian surface has side of length a = 10 cm. Electric field lines are parallel to x-axis as shown. The magnitudes of electric fields through surfaces ABCD and EFGH are 6kNC-1 and 9kNC-1 respectively. Then the total charge enclosed by the cube is
[Take ε0 = 9 × 10-12 Fm-1]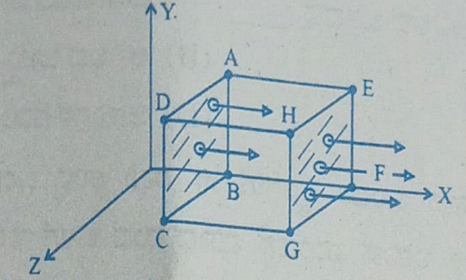
- KCET - 2023
- Gauss Law
- The value of \(\begin{vmatrix} \sin^2 14 \degree & \sin^2 66\degree & \tan 135\degree \\ \sin^2 66\degree & \tan 135\degree & \sin^2 14 \degree \\ \tan 135\degree & \sin^2 14 \degree & \sin^2 66\degree \end{vmatrix}\)
- KCET - 2023
- Trigonometric Identities
View More Questions
SUBSCRIBE TO OUR NEWS LETTER
COLLEGE NOTIFICATIONSEXAM NOTIFICATIONSNEWS UPDATES
Download the Collegedunia app on 
© 2024 Collegedunia Web Pvt. Ltd. All Rights Reserved