Question:
If the refractive index from air to glass is $\frac{3}{2}$ and
that from air to water is $\frac{4}{3}$, then the ratio of focal lengths of a glass lens in water and in air is
If the refractive index from air to glass is $\frac{3}{2}$ and
that from air to water is $\frac{4}{3}$, then the ratio of focal lengths of a glass lens in water and in air is
Updated On: Feb 23, 2024
- $1: 2$
- $2: 1$
- $1: 4$
- $4: 1$
Hide Solution
Verified By Collegedunia
The Correct Option is D
Solution and Explanation
$n _{ w }=\frac{4}{3} \,\,\, n _{ g }=\frac{3}{2}$
$f _{ a }\left( n _{ g }-1\right)= f _{ w }\left(\frac{ n _{ g }}{ n _{ w }}-1\right)$
$ \frac{ f _{ w }}{ f _{ a }}=\frac{ n _{ g }-1}{\frac{ n _{ g }}{ n _{ w }}-1}=\frac{\frac{3}{2}-1}{\frac{\frac{3}{2}}{\frac{4}{3}}-1} $
$=\frac{\frac{3-2}{2}}{\frac{9}{8}-1}=\frac{\frac{1}{2}}{\frac{9-8}{8}}=\frac{\frac{1}{2}}{\frac{1}{8}}=\frac{8}{2}$
$ \therefore \frac{ f _{ w }}{ f _{ a }}=\frac{4}{1} $
$f _{ a }\left( n _{ g }-1\right)= f _{ w }\left(\frac{ n _{ g }}{ n _{ w }}-1\right)$
$ \frac{ f _{ w }}{ f _{ a }}=\frac{ n _{ g }-1}{\frac{ n _{ g }}{ n _{ w }}-1}=\frac{\frac{3}{2}-1}{\frac{\frac{3}{2}}{\frac{4}{3}}-1} $
$=\frac{\frac{3-2}{2}}{\frac{9}{8}-1}=\frac{\frac{1}{2}}{\frac{9-8}{8}}=\frac{\frac{1}{2}}{\frac{1}{8}}=\frac{8}{2}$
$ \therefore \frac{ f _{ w }}{ f _{ a }}=\frac{4}{1} $
Was this answer helpful?
0
0
Top Questions on Spherical Mirrors
- Distance between twice-magnified virtual image of an object placed in front of mirror is 15 cm. Find focal length of spherical mirror in cm.
- JEE Main - 2024
- Physics
- Spherical Mirrors
- Distance between virtual image, which is twice the size of object placed in front of mirror and object is 45 cm. The magnitude of focal length of the mirror is _____cm.
- JEE Main - 2024
- Physics
- Spherical Mirrors
- A convex lens of focal length $20\, cm$ is placed in front of convex mirror with principal axis coinciding each other The distance between the lens and mirror is $10\, cm$ A point object is placed on principal axis at a distance of $60 \, cm$ from the convex lens The image formed by combination coincides the object itself The focal length of the convex mirror is ________ $cm$
- JEE Main - 2023
- Physics
- Spherical Mirrors
- The radius of curvature of a spherical mirror is 16 cm. What is the focal length ?
- TS POLYCET - 2023
- Physics
- Spherical Mirrors
- When the object lies at infinite distance in front of concave mirror then the nature of the image is
- WBJEE JENPAS UG - 2023
- Physical Sciences
- Spherical Mirrors
View More Questions
Questions Asked in KCET exam
- If \(\lim\limits_{x \rightarrow 0} \frac{\sin(2+x)-\sin(2-x)}{x}\)= A cos B, then the values of A and B respectively are
- KCET - 2023
- limits of trigonometric functions
- The Curie temperatures of Cobalt and iron are 1400K and 1000K respectively. At T = 1600K , the ratio of magnetic susceptibility of Cobalt to that of iron is
- KCET - 2023
- Magnetism and matter
- A particle is in uniform circular motion. Related to one complete revolution of the particle, which among the stataments is incorrect ?
- KCET - 2023
- Uniform Circular Motion
- The modulus of the complex number \(\frac{(1+i)^2(1+3i)}{(2-6i)(2-2i)}\) is
- KCET - 2023
- complex numbers
- The energy gap of an LED is 2.4 eV. When the LED is switched ‘ON’, the momentum of the emitted photons is
- KCET - 2023
- Semiconductor electronics: materials, devices and simple circuits
View More Questions
Concepts Used:
Spherical Mirrors
A spherical mirror is a mirror which has been cut out of a spherical surface.
There are two kinds of spherical mirrors:
- Convex Mirror
- Concave Mirror
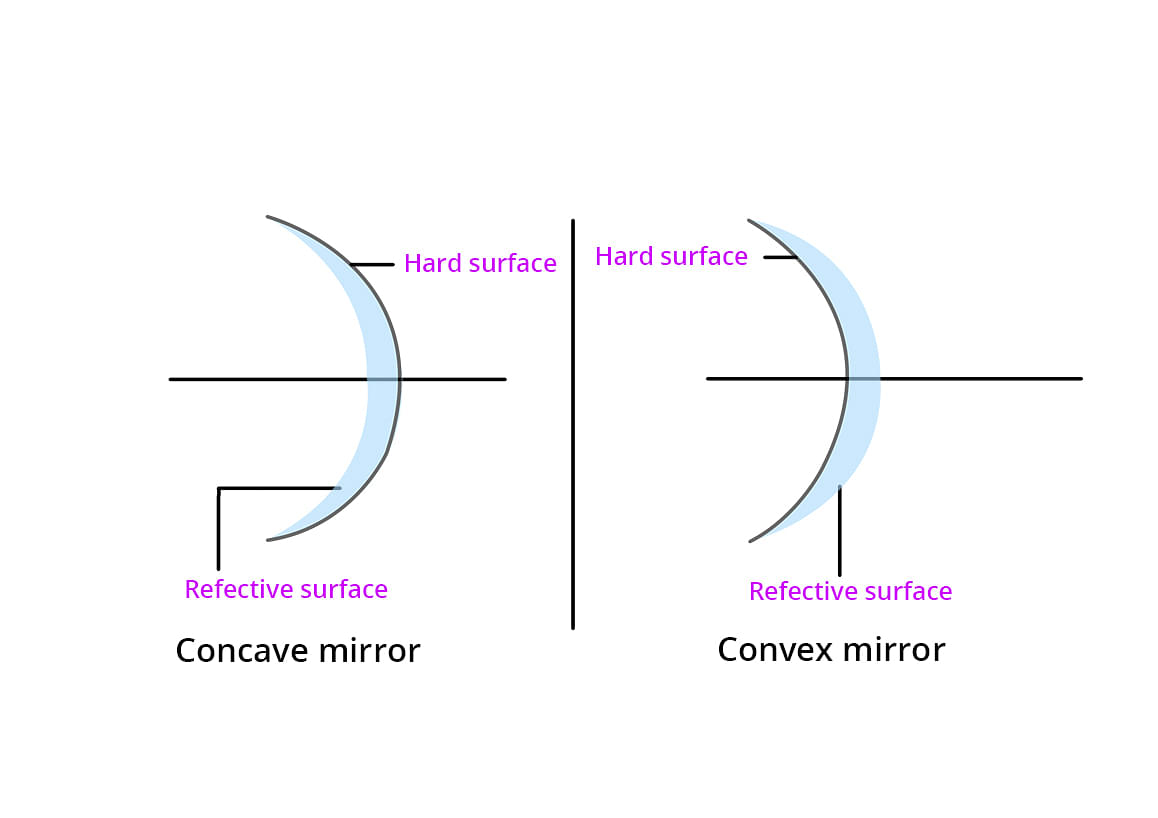
Concave Mirror
Concave mirrors are also called converging mirrors, because in these types of mirrors, light rays converge at a point after impact and reflect back from the reflective surface of the mirror.
Convex Mirror
The convex mirror has a reflective surface that is curved outward. Regardless of the distance between the subject and the mirrors, these mirrors are "always" virtual, upright and reduced.



