Find linear charge density if infinite line charges produce a field of 9 x 104 and the distance is 2 cm.
Solution and Explanation
Electric field due to infinite line charge is given by:
\(E=\frac{\lambda}{2\pi\epsilon_0d}\)
⇒ \(\lambda=2\pi\epsilon_0Ed\) …….....(1)
Where λ is the linear charge density and d is the perpendicular distance of a point from the infinite line charge.
Step 2: Substituting the values
∵\(\frac{1}{4\pi\epsilon_0}=9\times10^9Nm^2C^{-2}\)
⇒ \(2\pi\epsilon_0=\frac{1}{18\times10^9Nm^2C^{-2}}\)
From Eq (1): \(\lambda=\frac{1\times9\times10^4NC^{-1}\times0.02}{18\times10^9Nm^2C^{-2}}=10^{-7}Cm^{-1}\)
Top Questions on Electrostatic potential
- As shown in figure, a cuboid lies in a region with electric field \(E=2 x^2 \hat{i}-4 y \hat{j}+6 \hat{k} N / C\). The magnitude of charge within the cuboid is \(n \epsilon_0 C\). The value of \(n\) is _____. (if dimension of cuboid is \(1 \times 2 \times 3 m ^3\) )
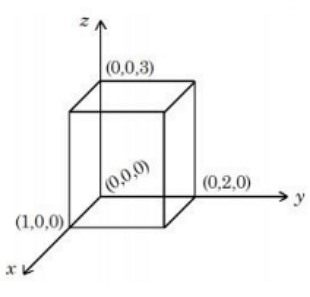
- JEE Main - 2023
- Physics
- Electrostatic potential
- Three identical particle $A, B$ and$ C$ of mass $100 \,kg$ each are placed in a straight line with $AB = BC =13 \,m$ The gravitational force on a fourth particle $P$ of the same mass is $F$, when placed at a distance $13\, m$ from the particle B on the perpendicular bisector of the line $AC$ The value of $F$ will be approximately :
- JEE Main - 2023
- Physics
- Electrostatic potential
- Eight copper wire of length $l$ and diameter $d$ are joined in parallel to form a single composite conductor of resistance $R$ If a single copper wire of length $2 l$ have the same resistance $(R)$ then its diameter will be ______$d$
- JEE Main - 2023
- Physics
- Electrostatic potential
- Two parallel plate capacitors of capacity $C$ and $3 C$ are connected in parallel combination and charged to a potential difference $18\, V$ The battery is then disconnected and the space between the plates of the capacitor of capacity $C$ is completely filled with a material of dielectric constant $9$ The final potential difference across the combination of capacitors will be______ $V$
- JEE Main - 2023
- Physics
- Electrostatic potential
A point source of light is placed at the focus of a concave mirror. Consider only paraxial rays. The shapes of the wavefronts of incident and reflected lights respectively are:
- TS EAMCET - 2023
- Physics
- Electrostatic potential
Questions Asked in AP EAMCET exam
Six coins tossed simultaneously then find the probability of getting at least 4 heads.
- AP EAMCET - 2023
- Probability
- In the chessboard if the rectangle form is 1296, find the total number of squares formed.
- AP EAMCET - 2023
- Coordinate Geometry
Find the products formed if chlorine reacts with the cold and dilute sodium hydroxide solution.
- AP EAMCET - 2023
- Chemical Reactions
- Find the coefficient of \(\frac{y^3}{x^8}\) in \((x+y)^{-5}\) ?
- AP EAMCET - 2023
- Binomial theorem
- Which compound is gem-dihalide?
- AP EAMCET - 2023
- Organic Chemistry- Some Basic Principles and Techniques
Concepts Used:
Electrostatic Potential
The electrostatic potential is also known as the electric field potential, electric potential, or potential drop is defined as “The amount of work that is done in order to move a unit charge from a reference point to a specific point inside the field without producing an acceleration.”
SI Unit of Electrostatic Potential:
SI unit of electrostatic potential - volt
Other units - statvolt
Symbol of electrostatic potential - V or φ
Dimensional formula - ML2T3I-1
Electric Potential Formula:
The electric potential energy of the system is given by the following formula:
U = 1/(4πεº) × [q1q2/d]
Where q1 and q2 are the two charges that are separated by the distance d.



