List of top Physics Questions on Magnetism and matter

An electron moves through a uniform magnetic field \( \vec{B} = B_0 \hat{i} + 2B_0 \hat{j} \, \text{T} \). At a particular instant of time, the velocity of the electron is \( \vec{u} = 3\hat{i} + 5\hat{j} \, \text{m/s} \). If the magnetic force acting on the electron is \( \vec{F} = 5e k \, \text{N} \), where \( e \) is the charge of the electron, then the value of \( B_0 \) is _____ T

A straight magnetic strip has a magnetic moment of \( 44 \, \text{A m}^2 \). If the strip is bent in a semicircular shape, its magnetic moment will be \( \dots \) A m\(^2\). \[ \text{(Given } \pi = \frac{22}{7} \text{)} \]

A proton and a deuteron (\( q = +e, \, m = 2.0u \)) having the same kinetic energies enter a region of uniform magnetic field \( \vec{B} \), moving perpendicular to \( \vec{B} \). The ratio of the radius \( r_d \) of the deuteron path to the radius \( r_p \) of the proton path is:

A regular polygon of 6 sides is formed by bending a wire of length 4 \(\pi\) meter. If an electric current of \(4 \pi \sqrt3 \) A is flowing through the sides of the polygon, the magnetic field at the centre of the polygon would be \(x × 10^{–7}\) T. The value of \(x\) is ____.

Two long, straight wires carry equal currents in opposite directions as shown in figure. The separation between the wires is 5.0 cm. The magnitude of the magnetic field at a point P midway between the wires is ____ μT
(Given: \(\mu_0 = 4\pi \times 10^{-7} \, \text{TmA}^{-1}\))

The magnetic potential due to a magnetic dipole at a point on its axis situated at a distance of 20 cm from its center is \( 1.5 \times 10^{-5} \, \text{Tm} \). The magnetic moment of the dipole is _______ \( \text{Am}^2 \).
(Given: \( \frac{\mu_0}{4\pi} = 10^{-7} \, \text{TmA}^{-1} \))

A particle of mass m is moving in the potential
\[ V(x) = \begin{cases} V_0 + \frac{1}{2} m \omega_{0P}^2 x^2 & \quad \text{if } x > 0 \\\infty & \quad \text{if } x \leq 0 \end{cases}\]
Figures P, Q, R, and S show different combinations of the values of \( \omega_0 \) and \( V_0 \).

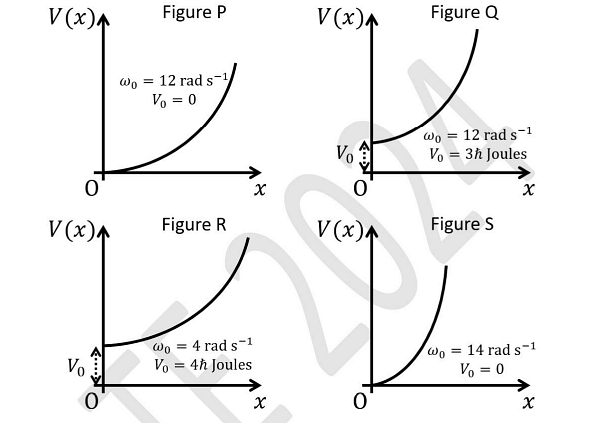
Let \( E^{(P)}_j \), \( E^{(Q)}_j \), \( E^{(R)}_j \), and \( E^{(S)}_j \) with \( j = 0, 1, 2, \ldots \), be the eigen-energies of the j-th level for the potentials shown in Figures P, Q, R, and S, respectively. Which of the following statements is/are true?

A material behaves as a superconductor below a critical temperature \( T_c \) and as a normal conductor above \( T_c \). A magnetic field \(\overrightarrow{B} = B\hat{z}\) is applied when \( T > T_c \). The material is then cooled below \( T_c \) in the presence of \( \vec{B} \). Which of the following figures represents the correct configuration of magnetic field lines?

The temperature \( T \) dependence of magnetic susceptibility \( \chi \) (Column I) of certain magnetic materials (Column II) are given below. Which of the following options is/are correct?

temperature T dependence of magnetic susceptibility x

An infinite one-dimensional lattice extends along the x-axis. At each lattice site, there exists an ion with spin 1/2. The spin can point either in the +z or -z direction only. Let \( S_P \), \( S_F \), and \( S_A \) denote the entropies of paramagnetic, ferromagnetic, and antiferromagnetic configurations, respectively. Which of the following relations is/are true?

Match List I with List II:

List I		List II
A	AC generator	i	Presence of both L and C
B	Transformer	ii	Electromagnetic Induction
C	Resonance phenomenon to occur	iii	Quality factor
D	Sharpness of resonance	iv	Mutual Induction

Choose the correct answer from the options given below:

A proton is projected with speed v in magnetic field B of magnitude 1 T. The angle between velocity and magnetic field is 600 as shown below. The kinetic energy of a proton is 2 eV (mass of proton = \(1.67\times10^{-27} kg\), e = \(1.6\times10^{-19}\)C). The pitch of the path of the proton is approximately?

The Curie temperatures of Cobalt and iron are 1400K and 1000K respectively. At T = 1600K , the ratio of magnetic susceptibility of Cobalt to that of iron is

Statement —I: Electromagnets are made of soft iron.
Statement —II: Soft iron has lower permeability and high retentivity.
Choose the correct option related to statements.

A big circular coil of 1000 turns and average radius 10 m is rotating about its horizontal diameter at 2 rad s^-1. If the vertical component of earth’s magnetic field at that place is 2 × 10^-5 T and electrical resistance of the coil is 12.56 Ω, then the maximum induced current in the coil will be:

When you walk through a metal detectorcarrying a metal object in your pocket, itraises an alarm This phenomenon works on

The magnetic moment of an electron (e) revolving in an orbit around nucleus with an orbital angular momentum is given by :

A toroid with 500 turns of wire carries a current of (2\(\pi\)) Ampere. A metal ring inside the toroid provides the core and has susceptibility of 2x10^-5. If the magnetization 5x10^-2 A/m, then radius of the ring is:

A long solenoid is formed by the winding 20 turns/cm. What will be the current necessary to produce a magnetic field of 20 millitesla inside the solenoid?
(\(\frac{μ_0}{4π}=10^{−7}\) tesla−meter/ampere)

In the magnetic meridian of a certain plane,the horizontal component of earth's magnetic field is 0.36 Gauss and the dip angle is 60°. The magnetic field of the earth at location is:

A proton and an alpha-particle moving with the same velocity enter a uniform magnetic field with their velocities perpendicular to the magnetic field. The ratio of radii of their circular paths is

The horizontal component of the earth’s magnetic field can be represented as

Three wires of equal lengths are bent in the form of loops. One of the loops is a circle, another is a semi-circle and the third one is a square. They are placed in a uniform magnetic field and the same electric current is passed through them. Which of the following loop configuration will experience greater torque?

Two bar magnets oscillate in a horizontal plane in earth’s magnetic field with time periods of 3 s and 4 s respectively. If their moments of inertia are in the ratio of 3 : 2, then the ratio of their magnetic moments will be :

A magnetizing field of 1000 A/m produces a magnetic flux of 2.4 x 10^-5 Wb in an iron bar of cross-sectional area 0.3 cm².The magnetic permeability of the iron bar in SI unit is

Column I	Column II
(1)	(P) Diamagnetic
(2)	(Q) Paramagnetic
(3)	(R) Ferromagnetic
(4)	(S) Antiferromagnetic

List of top Physics Questions on Magnetism and matter

SUBSCRIBE TO OUR NEWS LETTER