Question:
The equation $\frac{x^2}{1-r}-\frac{y^2}{1+r}=1,|r|<1$ represents
The equation $\frac{x^2}{1-r}-\frac{y^2}{1+r}=1,|r|<1$ represents
Updated On: Jun 14, 2022
- an ellipse
- a hyperbola
- a circle
- None of the above
Hide Solution
Verified By Collegedunia
The Correct Option is B
Solution and Explanation
Given equation is $\frac{x^2}{1-r}-\frac{y^2}{1+r}=1,Where |r|<1$
$\Rightarrow$ $1-r is (+ve) and 1+r is (+ve)$
$\therefore$ Given equation is of the form$\frac{x^2}{a^2}-\frac{y^2}{b^2}=1.$
Hence, it represents a hyperbola when $|r|<1.$
$\Rightarrow$ $1-r is (+ve) and 1+r is (+ve)$
$\therefore$ Given equation is of the form$\frac{x^2}{a^2}-\frac{y^2}{b^2}=1.$
Hence, it represents a hyperbola when $|r|<1.$
Was this answer helpful?
0
0
Top Questions on Conic sections
- Consider the ellipse \(\frac{x^2}{9}+\frac{y^2}{4}=1\). Let S (p, q) be a point in the first quadrant such that \(\frac{p^2}{9}+\frac{q^2}{4}>1\). Two tangents are drawn from S to the ellipse, of which one meets the ellipse at one end point of the minor axis and the other meets the ellipse at a point T in the fourth quadrant. Let R be the vertex of the ellipse with positive x-coordinate and Obe the center of the ellipse. If the area of the triangle △ORT is \(\frac{3}{2}\) , then which of the following options is correct ?
- JEE Advanced - 2024
- Mathematics
- Conic sections
- A normal with slope \(\frac{1}{\sqrt6}\) is drawn from the point (0, -α) to the parabola x2 = -4αy, where a > 0. Let L be the line passing through (0, -α) and parallel to the directrix of the parabola. Suppose that L intersects the parabola at two points A and B. Let r denote the length of the latus rectum and s denote the square of the length of the line segment AB. If r : s = 1 : 16 then the value of 24a is __________.
- JEE Advanced - 2024
- Mathematics
- Conic sections
- A particle moves along the curve \(\frac{x^2}{16}+\frac{y^2}{4}=1\). When the rate of change of abscissa is 4 times that of its ordinate, then the quadrant in which the particle lies is
- KCET - 2023
- Mathematics
- Conic sections
- The equations of two sides of a variable triangle are $x-0$ and $y=3$, and its third side is a tangent to the parabola $y^2=6 x$. The locus of its circumcentre is:
- JEE Main - 2023
- Mathematics
- Conic sections
Let P be a point on the parabola y2 = 4ax, where a > 0. The normal to the parabola at P meets the x -axis at a point Q. The area of the triangle PFQ where F is the focus of the parabola, is 120. If the slope m of the normal and a are both positive integers, then the pair (a, m) is
- JEE Advanced - 2023
- Mathematics
- Conic sections
View More Questions
Questions Asked in JEE Advanced exam
- A region in the form of an equilateral triangle (in x-y plane) of height L has a uniform magnetic field 𝐵⃗ pointing in the +z-direction. A conducting loop PQR, in the form of an equilateral triangle of the same height 𝐿, is placed in the x-y plane with its vertex P at x = 0 in the orientation shown in the figure. At 𝑡 = 0, the loop starts entering the region of the magnetic field with a uniform velocity 𝑣 along the +x-direction. The plane of the loop and its orientation remain unchanged throughout its motion.
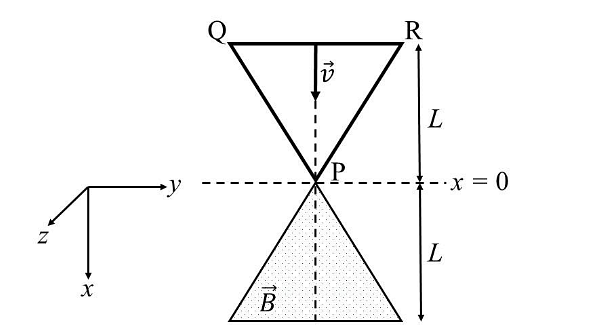
Which of the following graph best depicts the variation of the induced emf (E) in the loop as a function of the distance (𝑥) starting from 𝑥 = 0? - Two beads, each with charge q and mass m, are on a horizontal, frictionless, non-conducting, circular hoop of radius R. One of the beads is glued to the hoop at some point, while the other one performs small oscillations about its equilibrium position along the hoop. The square of the angular frequency of the small oscillations is given by [ \(\epsilon_0 \)is the permittivity of free space.]
- JEE Advanced - 2024
- Moving charges and magnetism
- A group of 9 students, s1, s2,…., s9, is to be divided to form three teams X, Y and Z of sizes 2, 3, and 4, respectively. Suppose that s1 cannot be selected for the team X and s2 cannot be selected for the team Y. Then the number of ways to form such teams, is _______.
- JEE Advanced - 2024
- Combinations
- Let \(\vec{p}=2\hat{i}+\hat{j}+3\hat{k}\) and \(\vec{q}=\hat{i}-\hat{j}+\hat{k}\). If for some real numbers α, β and γ we have
\(15\hat{i}+10\hat{j}+6\hat{k}=α(2\vec{p}+\vec{q})+β(\vec{p}-2\vec{q})+γ(\vec{p}\times\vec{q})\),
then the value of γ is ________.- JEE Advanced - 2024
- Vector Algebra
- Let X be a random variable, and let P(X = x) denote the probability that X takes the value x. Suppose that the points (x, P(X = x)), x = 0,1,2,3,4, lie on a fixed straight line in the xy -plane, and P(X = x) = 0 for all x ∈ R - {0,1,2,3,4}. If the mean of X is \(\frac{5}{2}\) , and the variance of X is α, then the value of 24α is ______.
- JEE Advanced - 2024
- Probability
View More Questions



