lim(x→0)\((\frac {1+tanx}{1+sinx})^{cosec x}\) = ?
lim(x→0)\((\frac {1+tanx}{1+sinx})^{cosec x}\) = ?
- 0
- e
- 1
\(\frac {1}{e}\)
The Correct Option is C
Approach Solution - 1
Let's consider the limit as x approaches 0:
lim(x→0) \((\frac {1+tanx}{1+sinx})^{cosec x}\) as x approaches 0
Substituting x = 0 into the expression gives us:
\((\frac {1+tan(0)}{1+sin(0)})^{cosec (0)}\)
Since tan(0) = 0 and sin(0) = 0, we have:
\((\frac {1+0}{1+0})\)(cosec 0)
Simplifying further:
1(cosec 0)
1
Therefore, the correct option is (C) 1.
Approach Solution -2
\(\lim_{x \to 0} \left( \frac{1 + \tan x}{1 + \sin x} \right)^{\cosec x}\)
we can use approximations and simplifications. Here's a streamlined approach:
Approximate tan x and sin x for small x:
\(\tan x \approx x\)
\(\sin x \approx x\)
Rewrite the expression using these approximations:
\(\frac{1 + \tan x}{1 + \sin x} \approx \frac{1 + x}{1 + x} = 1\)
Consider the entire expression:
\(\left( \frac{1 + \tan x}{1 + \sin x} \right)^{\cosec x} \approx 1^{\cosec x}\)
Note that \(\cosec x = \frac{1}{\sin x}\), and for small x, \(\sin x \approx x\):
\(\cosec x \approx \frac{1}{x}\)
Substitute \(\cosec x \approx \frac{1}{x}\) into the expression:
\(1^{\cosec x} \approx 1^{\frac{1}{x}}\)
Recognize that 1 raised to any power is still 1:
\(1^{\frac{1}{x}} = 1\)
So, the correct option is (C): 1
Top Questions on limits and derivatives
- If α > β > 0 are the roots of the equation ax2 + bx + 1 = 0, and \(\lim\limits_{x \to \frac{1}{\alpha}}\left(\frac{1-cos(x^2+bx+a)}{2(1-ax)^2}\right)^{1/2}=\frac{1}{k}\left(\frac{1}{\beta}-\frac{1}{\alpha}\right),\) then k is equal to
- JEE Main - 2023
- Mathematics
- limits and derivatives
- For any positive integer 7m-3m is divisible by
- CUET (PG) - 2023
- Mathematics
- limits and derivatives
- The derivative \(\frac{dy}{dx} \) of the function y = sin(cos(log(x))) is,
- CUET (PG) - 2023
- Mathematics
- limits and derivatives
- Let f(x)=xm, m being a non-negative integer. The value of m so that the equality f'(a+b)=f'(a)+f'(b) is valid for all a,b>0 is
- WBJEE - 2023
- Mathematics
- limits and derivatives
Lim x -> 0 x.cot4x / (sin2x cot2(2x))
- MHT CET - 2023
- Mathematics
- limits and derivatives
Questions Asked in MHT CET exam
- Two monkeys off mass 10 kg and 8 kg are moving along a vertical light rope the former climbing up with an acceleration of 2 m/second square while the latter coming down with a uniform velocity of 2 m/sec square find the tension in the rope at the fixed support
- MHT CET - 2024
- tension
- Total genetic content of an organism is called
- MHT CET - 2024
- Non-Mendelian Genetics
- How many ATP molecules are needed as an initial investment in the glycolytic cycle (normal glycolysis)?
- MHT CET - 2024
- Glycolysis
- Which disease is primarily spread by female Anopheles mosquitoes?
- MHT CET - 2024
- HIV and AIDS
- If \(A=\begin{bmatrix} 2a & -3b\\ 3 & 2\end{bmatrix}\) and \(\text{adj}A = AA^T\), then 2a+3b is?
- MHT CET - 2023
- Matrices
MHT CET Notification
 MHT CET 2024 CAP Round 2 Seat Allotment Out, Direct Link here.Aug 26, 2024
MHT CET 2024 CAP Round 2 Seat Allotment Out, Direct Link here.Aug 26, 2024Concepts Used:
Limits of Trigonometric Functions
Assume a is any number in the general domain of the corresponding trigonometric function, then we can explain the following limits.
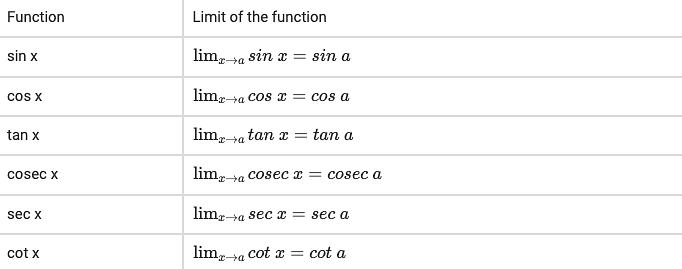
We know that the graphs of the functions y = sin x and y = cos x detain distinct values between -1 and 1 as represented in the above figure. Thus, the function is swinging between the values, so it will be impossible for us to obtain the limit of y = sin x and y = cos x as x tends to ±∞. Hence, the limits of all six trigonometric functions when x tends to ±∞ are tabulated below:




