Select Goal &
City
Select Goal
Explore
Question:
Let $M$ be a $3 \times 3$ invertible matrix with real entries and let $I$ denote the $3 \times 3$ identity matrix. If $M^{-1}=\text{adj}(\text{adj} M)$, then which of the following statements is/are ALWAYS TRUE?
Let $M$ be a $3 \times 3$ invertible matrix with real entries and let $I$ denote the $3 \times 3$ identity matrix. If $M^{-1}=\text{adj}(\text{adj} M)$, then which of the following statements is/are ALWAYS TRUE?
Updated On: May 23, 2024
- $M=I$
- $\text{det} M=1$
- $M^{2}=I$
- $(\text{adj} M)^{2}=I$
Hide Solution
Verified By Collegedunia
The Correct Option is B, C, D
Solution and Explanation
(B) $\text{det} M=1$
(C) $M^{2}=I$
(D) $(\text{adj} M)^{2}=I$
(C) $M^{2}=I$
(D) $(\text{adj} M)^{2}=I$
Was this answer helpful?
0
0
Top Questions on Applications of Determinants and Matrices
- The system of the equations
\(x + y + z = 6\)
\(x + 2y + \alpha z = 5 \)
\(x + 2y + 6z = \)\( \beta\) has- JEE Main - 2023
- Mathematics
- Applications of Determinants and Matrices
- For the system of linear equations $\alpha x+y+z=1, x+\alpha y+z=1, x+y+\alpha z=\beta$, which one of the following statements is NOT correct ?
- JEE Main - 2023
- Mathematics
- Applications of Determinants and Matrices
- Let Ξ±, Ξ² and Ξ³ be real numbers such that the system of linear equations
\(x + 2y + 3z = Ξ±\)
\(4x + 5y + 6z = Ξ²\)
\(7x + 8y + 9z = Ξ³ β 1 \)
is consistent. Let\( |M|\) represent the determinant of the matrix.
\(M = \begin{bmatrix} \alpha & 2 & \gamma &\\ \beta & 1 & 0 & \\-1& 0&1 \end{bmatrix}\)
Let P be the plane containing all those \((Ξ±, Ξ², Ξ³)\) for which the above system of linear equations is consistent, and D be the square of the distance of the point (0, 1, 0) from the plane P.- JEE Advanced - 2021
- Mathematics
- Applications of Determinants and Matrices
\(\text{If} \begin{vmatrix} 1 & x & x^2 \\ x & x^2 & 1 \\ x^2 & 1 & x \end{vmatrix} = 7\ \text{and}\ \triangle = \begin{vmatrix} x^3 - 1 & 0 & x - x^4 \\ 0 & x - x^3 & x^3 - 1 \\ x - x^4 & x^3 - 1 & 0 \end{vmatrix}, \text{then}\)
- SRMJEEE - 2018
- Mathematics
- Applications of Determinants and Matrices
- \[\text{If}\ \triangle = \begin{vmatrix} 3 & 4 & 5 & x \\ 4 & 5 & 6 & y \\ 5 & 6 & 7 & z \\ x & y & z & 0 \end{vmatrix} = 0\]
- SRMJEEE - 2018
- Mathematics
- Applications of Determinants and Matrices
View More Questions
Questions Asked in JEE Advanced exam
- Let the function \(f:[1,\infin)β\R\) be defined by
\(f(t) = \begin{cases} (-1)^{n+1}2, & \text{if } t=2n-1,n\in\N, \\ \frac{(2n+1-t)}{2}f(2n-1)+\frac{(t-(2n-1))}{2}f(2n+1) & \text{if } 2n-1<t<2n+1,n\in\N. \end{cases}\)
Define \(g(x)=\int\limits_{1}^{x}f(t)dt,x\in(1,\infin).\) Let Ξ± denote the number of solutions of the equation g(x) = 0 in the interval (1, 8] and \(Ξ²=\lim\limits_{xβ1+}\frac{g(x)}{x-1}\). Then the value of Ξ± + Ξ² is equal to _____.- JEE Advanced - 2024
- Integral Calculus
- A dimensionless quantity is constructed in terms of electronic charge \(e\), permittivity of free space \(\epsilon_0\) , Planckβs constant β, and speed of light c. If the dimensionless quantity is written as \(e^\alpha\epsilon_0^\beta h^\gamma c^\delta\)and n is a non-zero integer, then\((\alpha, \beta,\gamma,\delta)\) is given by
- JEE Advanced - 2024
- Semiconductor electronics: materials, devices and simple circuits
- A block of mass \(5 kg\) moves along the \(x-\)direction subject to the force \(F = (β20x + 10) N,\) with the value of \(x \) in metre. At time \(t = 0 s,\) it is at rest at position \(x = 1 m\). The position and momentum of the block at \(t = (\pi/4)\) s are
- JEE Advanced - 2024
- Work-energy theorem
- A region in the form of an equilateral triangle (in x-y plane) of height L has a uniform magnetic field π΅β pointing in the +z-direction. A conducting loop PQR, in the form of an equilateral triangle of the same height πΏ, is placed in the x-y plane with its vertex P at x = 0 in the orientation shown in the figure. At π‘ = 0, the loop starts entering the region of the magnetic field with a uniform velocity π£ along the +x-direction. The plane of the loop and its orientation remain unchanged throughout its motion.
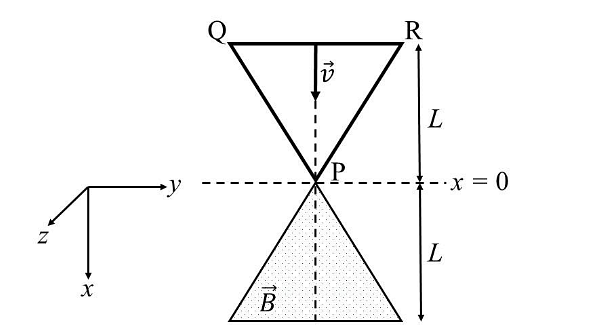
Which of the following graph best depicts the variation of the induced emf (E) in the loop as a function of the distance (π₯) starting from π₯ = 0? - Two beads, each with charge q and mass m, are on a horizontal, frictionless, non-conducting, circular hoop of radius R. One of the beads is glued to the hoop at some point, while the other one performs small oscillations about its equilibrium position along the hoop. The square of the angular frequency of the small oscillations is given by [ \(\epsilon_0 \)is the permittivity of free space.]
- JEE Advanced - 2024
- Moving charges and magnetism
View More Questions
SUBSCRIBE TO OUR NEWS LETTER
COLLEGE NOTIFICATIONSEXAM NOTIFICATIONSNEWS UPDATES
Download the Collegedunia app on 
Β© 2024 Collegedunia Web Pvt. Ltd. All Rights Reserved