If \(\frac{1}{6}\)sinθ.cosθ.tanθ are in G.P, then the solution set θ is
- \(2n\pi\pm\frac{\pi}{6}\)
- \(2n\pi\pm\frac{\pi}{3}\)
- \(n\pi+(-1)^n\frac{\pi}{3}\)
- \(n\pi+\frac{\pi}{3}\)
The Correct Option is B
Approach Solution - 1
The correct answer is option (B): \(2n\pi\pm\frac{\pi}{3}\)
According to the given condition, if a,b and c are in GP,we have b2=ac
Similarly,
\(tan\theta sin\theta\times\frac{1}{6}=cos^2\theta\)
\(\Rightarrow sin^2\theta=6cos^3\theta\)
\(\Rightarrow 6cos^3\theta+cos^2\theta-1=0\)
Since \(cos\theta=\frac{1}{2}\), the general solution becomes \(2n\pi\pm\frac{\pi}{3}\)
Approach Solution -2
\( \cos^2 \theta = \frac{1}{6} \sin \theta \tan \theta \)
\( \cos^2 \theta = \frac{1}{6} \sin^2 \theta \cos \theta \)
\( \cos^3 \theta = \frac{\sin^2 \theta}{6} \)
\( 6 \cos^3 \theta = 1 - \cos^2 \theta \)
\( 6 \cos^3 \theta + \cos^2 \theta - 1 = 0 \)
This simplifies to \( (\cos \theta - \frac{1}{2})(6 \cos^2 \theta + 4 \cos \theta + 2) = 0 \)
Further simplification gives \( 2 (\cos \theta - \frac{1}{2})(3 \cos^2 \theta + 2 \cos \theta + 1) = 0 \)
Solving \( \cos \theta - \frac{1}{2} = 0 \) leads to \( \cos \theta = \frac{1}{2} \).
Therefore, \( \cos \theta = \cos \frac{\pi}{3} \).
Hence, \( \theta = 2n\pi \pm \frac{\pi}{3} \).
Top Questions on Trigonometric Functions
- If, \(x∈(0,π)\) satisfies the equation \(6^{1+sinx+sin^2x....}=36\) ,then the value of \(x\) is_____.
- KEAM - 2023
- Mathematics
- Trigonometric Functions
- The value of a(≠0) for which the equation \(\frac{1}{2}(x-2)^2+1=\sin(\frac{a}{x})\) holds is/are
- KEAM - 2023
- Mathematics
- Trigonometric Functions
- Let P(x)=cos2x+sin4x,for any x∈R.Then which of the following options is correct for all x?
- KEAM - 2023
- Mathematics
- Trigonometric Functions
- Minimum value of 5cos(2x) + 5sin(2x)
- BITSAT - 2023
- Mathematics
- Trigonometric Functions
- If $\tan 15^{\circ}+\frac{1}{\tan 75^{\circ}}+\frac{1}{\tan 105^{\circ}}+\tan 195^{\circ}=2 a$, then the value of $\left(a+\frac{1}{a}\right)$ is :
- JEE Main - 2023
- Mathematics
- Trigonometric Functions
Questions Asked in WBJEE exam
- Three identical convex lenses each of focal length f are placed in a straight line separated by a distance f from each other. An object is located in \(\frac{f}{2}\) in front of the leftmost lens. Then,

- WBJEE - 2023
- Ray optics and optical instruments
- \(\lim_{x\rightarrow \infty}\){\(x-\sqrt[n]{(x-a_1)(x-a_2)......(x-a_n)}\)} where a1,a2,.....an are positive rational numbers.The limit
- A particle of mass m is projected at velocity at a velocity u, making an angle θ with the horizontal(x-axis). If the angle of projection θ is varied keeping all other parameters same, then magnitude of angular momentum(L) at its maximum height about the point of projection varies with θ as,
- WBJEE - 2023
- System of Particles & Rotational Motion
- If \(\int \frac{dx}{(x+1)(x-2)(x-3)}=\frac{1}{k}log_e\left \{ \frac{|x-3|^3|x+1|}{(x-2)^4}\right \}+c\), then the value of k is
- WBJEE - 2023
- Integrals of Some Particular Functions
- A rectangle ABCD has its side parallel to the line y=2x and vertices A,B,D are on y=1,x=1 and x=-1 respectively. The coordinate of C can be
- WBJEE - 2023
- Straight lines
Concepts Used:
Limits of Trigonometric Functions
Assume a is any number in the general domain of the corresponding trigonometric function, then we can explain the following limits.
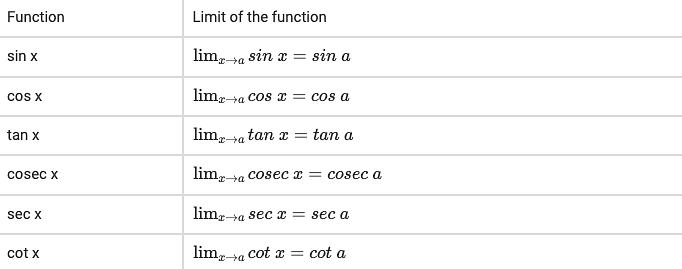
We know that the graphs of the functions y = sin x and y = cos x detain distinct values between -1 and 1 as represented in the above figure. Thus, the function is swinging between the values, so it will be impossible for us to obtain the limit of y = sin x and y = cos x as x tends to ±∞. Hence, the limits of all six trigonometric functions when x tends to ±∞ are tabulated below:




