Question:
A table tennis ball has radius \((3/2) × 10^{−2} \)m and mass \((22/7) × 10^{−3} \)kg. It is slowly pushed down into a swimming pool to a depth of 𝑑 = 0.7 m below the water surface and then released from rest. It emerges from the water surface at speed 𝑣, without getting wet, and rises up to a height 𝐻. Which of the following option(s) is(are) correct?[Given: \(\pi = 22/7, g = 10 m s ^{−2} \), density of water = \(1 × 10^3 kg\ m^{−3}\) , viscosity of water = \(1 × 10^{−3}\) Pa-s.]
A table tennis ball has radius \((3/2) × 10^{−2} \)m and mass \((22/7) × 10^{−3} \)kg. It is slowly pushed down into a swimming pool to a depth of 𝑑 = 0.7 m below the water surface and then released from rest. It emerges from the water surface at speed 𝑣, without getting wet, and rises up to a height 𝐻. Which of the following option(s) is(are) correct?[Given: \(\pi = 22/7, g = 10 m s ^{−2} \), density of water = \(1 × 10^3 kg\ m^{−3}\) , viscosity of water = \(1 × 10^{−3}\) Pa-s.]
Updated On: Jun 17, 2024
- The work done in pushing the ball to the depth 𝑑 is 0.077 J
- If we neglect the viscous force in water, then the speed 𝑣 = 7 m/s.
- If we neglect the viscous force in water, then the height 𝐻 = 1.4 m.
- The ratio of the magnitudes of the net force excluding the viscous force to the maximum viscous force in water is 500/9.
Hide Solution
Verified By Collegedunia
The Correct Option is A, B, D
Solution and Explanation
The correct option is (A): The work done in pushing the ball to the depth 𝑑 is 0.077 J, (B): If we neglect the viscous force in water, then the speed 𝑣 = 7 m/s. and (D): The ratio of the magnitudes of the net force excluding the viscous force to the maximum viscous force in water is 500/9.
Was this answer helpful?
0
1
Top Questions on Fluid Mechanics
- In a sand mold, a sprue of height \(h_2=200\ mm\) is to be provided for maintaining the molten metal flow rate of \(10^6\ mm^3/s\). The height of liquid column above the point 2 is kept constant at \(h_c=25\ mm\). The cross-sectional areas of the sprue at points 2 and 3 are \(A_2\) and \(A_3\), respectively. The points 1 and 3 are at the atmospheric pressure. Assuming the gauge pressure at point 2 to be zero as the limiting case to prevent aspiration effect, the ratio \(\frac {A_3}{A_2}\) _____ . (Rounded off to 2 decimal places)

- GATE PI - 2024
- General Engineering
- Fluid Mechanics
- A 5m × 5m closed tank of 10 m height contains water and oil, and is connected to an overhead water reservoir as shown in the figure. Use \(γ_w=10\ kN/m^3\) and Specific gravity of oil = 0.8

- GATE CE - 2024
- Water Resources Engineering
- Fluid Mechanics
- A spillway has unit discharge of 7.5 m3/s/m. The flow depth at the downstream horizontal apron is 0.5 m. The tail water depth (in meters) required to form a hydraulic jump is_____ (rounded off to 2 decimal places).
- GATE CE - 2024
- Water Resources Engineering
- Fluid Mechanics
A cylinder of mass m and material density ρ hanging from a string is lowered into a vessel of cross - sectional area A containing a liquid of density σ (< ρ) until it is fully immersed. The increase in pressure at the bottom of the vessel is:
- TS EAMCET - 2023
- Physics
- Fluid Mechanics
A cylindrical vessel, open at the top, contains 15 liters of water. Water drains out through a small opening at the bottom. 5 liters of water comes out in time t1, the next 5 litre in further time t2, and the last 5 litre in further time t3. then:
- TS EAMCET - 2023
- Physics
- Fluid Mechanics
View More Questions
Questions Asked in JEE Advanced exam
- A region in the form of an equilateral triangle (in x-y plane) of height L has a uniform magnetic field 𝐵⃗ pointing in the +z-direction. A conducting loop PQR, in the form of an equilateral triangle of the same height 𝐿, is placed in the x-y plane with its vertex P at x = 0 in the orientation shown in the figure. At 𝑡 = 0, the loop starts entering the region of the magnetic field with a uniform velocity 𝑣 along the +x-direction. The plane of the loop and its orientation remain unchanged throughout its motion.
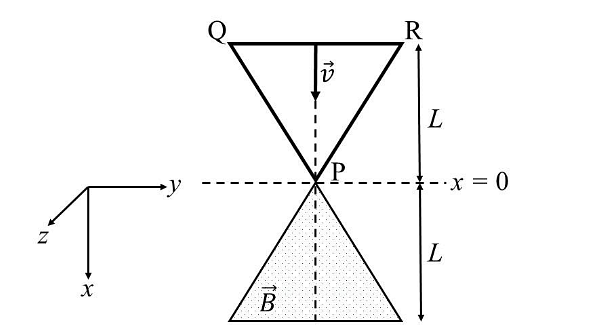
Which of the following graph best depicts the variation of the induced emf (E) in the loop as a function of the distance (𝑥) starting from 𝑥 = 0? - Two beads, each with charge q and mass m, are on a horizontal, frictionless, non-conducting, circular hoop of radius R. One of the beads is glued to the hoop at some point, while the other one performs small oscillations about its equilibrium position along the hoop. The square of the angular frequency of the small oscillations is given by [ \(\epsilon_0 \)is the permittivity of free space.]
- JEE Advanced - 2024
- Moving charges and magnetism
- A group of 9 students, s1, s2,…., s9, is to be divided to form three teams X, Y and Z of sizes 2, 3, and 4, respectively. Suppose that s1 cannot be selected for the team X and s2 cannot be selected for the team Y. Then the number of ways to form such teams, is _______.
- JEE Advanced - 2024
- Combinations
- Let \(\vec{p}=2\hat{i}+\hat{j}+3\hat{k}\) and \(\vec{q}=\hat{i}-\hat{j}+\hat{k}\). If for some real numbers α, β and γ we have
\(15\hat{i}+10\hat{j}+6\hat{k}=α(2\vec{p}+\vec{q})+β(\vec{p}-2\vec{q})+γ(\vec{p}\times\vec{q})\),
then the value of γ is ________.- JEE Advanced - 2024
- Vector Algebra
- Let X be a random variable, and let P(X = x) denote the probability that X takes the value x. Suppose that the points (x, P(X = x)), x = 0,1,2,3,4, lie on a fixed straight line in the xy -plane, and P(X = x) = 0 for all x ∈ R - {0,1,2,3,4}. If the mean of X is \(\frac{5}{2}\) , and the variance of X is α, then the value of 24α is ______.
- JEE Advanced - 2024
- Probability
View More Questions



