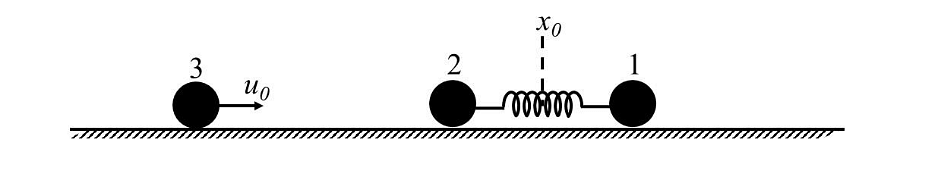
Two particles, 1 and 2, each of mass π, are connected by a massless spring, and are on a horizontal frictionless plane, as shown in the figure. Initially, the two particles, with their center of mass at
\(π₯_0\), are oscillating with amplitude π and angular frequency π. Thus, their positions at time π‘ are given by
\(x_1 (t) = (x_0 + d) + \alpha \ sin \omega t\) and
\(x_2t = (x_0 -d) β \alpha sin \ wt,\) respectively, where
\(π > 2π.\) Particle 3 of mass π moves towards this system with speed π’0 = ππ/2, and undergoes instantaneous elastic collision with particle 2, at time
\(π‘_0\). Finally, particles 1 and 2 acquire a center of mass speed
\(π£_{cm}\) and oscillate with amplitude π and the same angular frequency π.
