
Content Curator
A vertical line, in geometry, is a straight line that is perpendicular to a horizontal plane.
- In coordinate geometry, vertical lines always parallel to the y-axis and intersect horizontal lines at right angles.
- These lines extend either from top to bottom or vice versa and are commonly referred to as standing lines.
- Examples of vertical lines in everyday life are steel rails of a fence, towering trees, table legs, and electric poles.
Through this article, we will analyze the concept of vertical lines and explore their defining characteristics and real-life examples.
| Table of Content |
Key Terms: Cartesian coordinate system, Vertical lines, Horizontal lines, Slope of vertical line, Equation of vertical line, Perpendicular lines
Vertical Line Definition
[Click Here for Sample Questions]
In coordinate geometry, a vertical line is defined by all its points having the same x-coordinate.
- Therefore, a vertical line is perpendicular to the x-axis and parallel to the y-axis.
- Real-life examples of vertical lines include tall structures like towers, table and chair legs, and towering trees.
- Due to its perpendicular orientation to the x-axis, the slope of a vertical line is undefined.
- In the Cartesian plane, a vertical line extends vertically from top to bottom.
Vertical Lines
Also Read:
| Concept-Related Articles | ||
|---|---|---|
| Linear equation in Two Variables | Slope formula | Area of parallelogram |
| Introduction to Three-Dimensional Geometry | Motion in a Straight Line | Angle between Two Planes |
Vertical Line on a Coordinate Plane
[Click Here for Sample Questions]
A vertical line on a coordinate plane is a straight line that extends infinitely in the vertical direction.
- This line is perpendicular to the horizontal x-axis and parallel to the vertical y-axis.
- All points on this line share the same x-coordinate.
- Since the line is vertical, it does not have a defined slope, as it makes a 90-degree angle with the x-axis.
Vertical Line Equation
[Click Here for Sample Questions]
The equation of a vertical line crossing the x-axis at any point a is given by
x = a
Where "a" is a constant representing the x-coordinate of all points on the line.
This equation indicates that all points on the line have the same x-coordinate, while the y-coordinate can vary.
- For example, the equation x = 3 represents a vertical line passing through the point (3, y) for all values of y.
- Similarly, x = -2 represents a vertical line passing through the point (-2, y) for all values of y.
The slope of a Vertical Line
[Click Here for Sample Questions]
The slope of a vertical line is undefined. This is because a vertical line is perpendicular to the horizontal x-axis and parallel to the vertical y-axis.
- In other words, a vertical line rises or falls infinitely, but it does not run horizontally.
- The slope is calculated as the ratio of the vertical change (rise) to the horizontal change (run) between any two points on a line.
- This ratio cannot be determined for a vertical line because there is no horizontal change (run) between any two points on the line.
- Therefore, the slope of a vertical line is said to be undefined.
Vertical Line Test
[Click Here for Sample Questions]
The Vertical Line Test is a method used to determine if a curve or graph represents a function.
- The test is performed by drawing vertical lines across the graph.
- If any vertical line intersects the graph at more than one point, then the graph fails the Vertical Line Test and does not represent a function.
- In other words, if every vertical line intersects the graph at most once, then the graph represents a function.
- This test is particularly useful when visually analyzing graphs to determine if they represent functions or not.
For example, consider the graph of a circle. If you were to draw vertical lines across the circle, you would find that each vertical line intersects the circle at two points, meaning the circle does not represent a function. However, if you were to graph a straight line, you would find that every vertical line intersects the line at most once, indicating that the line represents a function.
Properties of Vertical Line
[Click Here for Sample Questions]
Vertical lines possess several distinct properties:
- Direction: Vertical lines extend infinitely in the vertical direction, perpendicular to the horizontal x-axis and parallel to the vertical y-axis.
- Equation Form: The equation of a vertical line is of the form x = constant, where the constant value specifies the x-coordinate of all points on the line.
- Slope: The slope of a vertical line is undefined because it does not have a horizontal component.
- Intersection: A vertical line intersects the x-axis at a single point, and it does not intersect the y-axis.
- Function Representation: A vertical line can represent a relation, but it cannot represent a function unless it is restricted to a single value of x (i.e., a vertical line with an equation x = a, where a is a constant).
- Visual Representation: On a Cartesian coordinate plane, vertical lines appear as straight lines extending vertically from top to bottom, with all points sharing the same x-coordinate.
Vertical Line of Symmetry
[Click Here for Sample Questions]
A vertical line of symmetry is a line that divides a shape or object into two mirror-image halves such that each half is a reflection of the other across the line. In other words, if a shape or object has a vertical line of symmetry, folding it along this line would result in both halves perfectly overlapping each other.
- For example, the letter "A" has a vertical line of symmetry.
- If you were to fold it along its central vertical axis, the left and right sides would match perfectly.
In terms of mathematical functions or graphs, a vertical line of symmetry refers to a line such that if a graph is folded along this line, the resulting portions of the graph are mirror images of each other.
- This often occurs in symmetrical functions such as parabolas and other even functions.
- Identifying vertical lines of symmetry is important in geometry, algebra, and other mathematical concepts.
- It helps in understanding the symmetry properties of shapes, graphs, and equations.
Solved Examples of Vertical Lines
[Click Here for Sample Questions]
| Ques. Plot the graph for the following equation of vertical lines:
The equation x = 6 represents a vertical line that intersects the x-axis at 8 units to the right of the origin. This line is parallel to the y-axis.
The equation x = - 6 represents a vertical line that intersects the x-axis at 6 units to the left of the origin. This line is also parallel to the y-axis.
|
Things To Remember
- A vertical line is a straight line that extends infinitely in the vertical direction.
- It is perpendicular to the horizontal x-axis and parallel to the vertical y-axis.
- The equation of a vertical line is of the form x = constant.
- The slope of a vertical line is undefined because it does not have a horizontal component.
- A vertical line intersects the x-axis at a single point and does not intersect the y-axis.
- A vertical line of symmetry divides a shape or object into two mirror-image halves such that each half is a reflection of the other across the line.
- A vertical line can represent a relation, but it cannot represent a function unless it is restricted to a single value of x.
Read Also:
Sample Questions
Ques: What is a vertical and a horizontal line? (2 Marks)
Ans: In a coordinate plane, a vertical line is parallel to the y-axis and extends infinitely in the vertical direction. On the other hand, a horizontal line is parallel to the x-axis and extends infinitely left and right.
Ques: What is the equation of vertical line? (1 Mark)
Ans: The equation of a vertical line crossing the x-axis at any point a is given by
x = a
Where a is a constant.
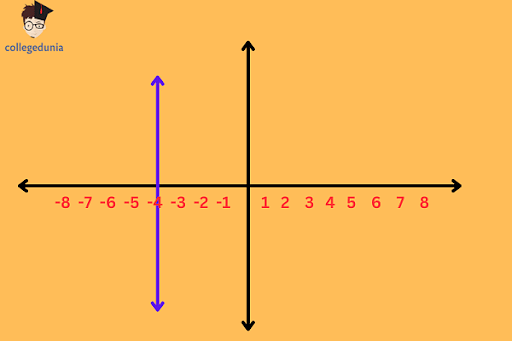
Ques: Sketch the graph of the equation x = - 4 on the Cartesian coordinate plane. (2 Marks)
Ans: The graph of x = -4 is a vertical line passing through the point (-4, 0) on the x-axis.
Ques: Find the equation of the vertical line passing through the point (5, -2). (1 Mark)
Ans: The equation of a vertical line passing through a point on the x-axis is given by the equation x = a
Therefore, the equation of the vertical line passing through the point (5, -2) is x = 5.
Ques: Determine the slope of the line passing through the points (-3, 4) and (-3, -2). (1 Mark)
Ans: Since the x-coordinate in both points is the same (-3), the line will be vertical. Therefore, the slope is undefined.
Ques: Does x = 0 represent a vertical line? (1 Mark)
Ans: Yes, x = 0 is a vertical line. This line passes through the origin and is parallel to the y-axis.
Ques: Sketch the graph of the equation x = - 5 on the Cartesian coordinate plane. (2 Marks)
Ans: The graph of x = -5 is a vertical line passing through the point (-5, 0) on the x-axis.

Ques: Does the graph of the equation y = 2x - 3 pass the Vertical Line Test? (2 Marks)
Ans: Yes, the graph of y = 2x - 3 passes the Vertical Line Test because every vertical line intersects the graph at most once, confirming that it represents a function.
In the equation y = 2x - 3, for any given x-value, there will be only one corresponding y-value due to the linear relationship. This means that a vertical line drawn anywhere on the graph will intersect the line at only one point where that specific x-value produces a y-value according to the equation.
Ques: Identify the vertical line(s) of symmetry, if any, for the shape represented by the equation y = x² - 4. (1 Mark)
Ans: The graph of y = x² - 4 is a parabola opening upwards. Since it is not symmetric about any vertical line, it does not have a vertical line of symmetry.
Ques: Determine whether the equation y = 5 represents a function. If yes, justify your answer. (1 Mark)
Ans: Yes, the equation y = 5 represents a function because for every input x, there is exactly one output y (5). Visually, it represents a horizontal line parallel to the x-axis.
Ques: Identify the vertical line(s) of symmetry, if any, for the shape represented by the equation y = -x² + 3x. (1 Mark)
Ans: The graph of y = -x² + 3x is a downward-opening parabola. Since it is not symmetric about any vertical line, it does not have a vertical line of symmetry.
For Latest Updates on Upcoming Board Exams, Click Here: https://t.me/class_10_12_board_updates
Check-Out:







Comments