The number of values of x in the interval \((\frac \pi 4, \frac {7\pi }{4})\) for which \(14cosec^2x – 2sin^2x = 21 – 4cos^2x\) holds, is ______.
Correct Answer: 4
Solution and Explanation
\(14cosec^2x – 2sin^2x = 21 – 4cos^2x\)
\(\frac {14}{sin^2x}−2sin^2 x = 21−4(1−sin^2x)\)
Let \(sin^2x = t\)
\(⇒ 14 – 2t^2 = 21t – 4t + 4t^2\)
\(⇒ 6t^2 + 17t – 14 = 0\)
\(⇒ 6t^2 + 21t – 4t – 14 = 0\)
\(⇒ 3t(2t + 7) – 2(2t + 7) = 0\)
\(⇒ (2t + 7) (3t– 2) = 0\)
\(⇒t = \frac 23\ or\ -\frac {7}{2}\)
\(⇒ sin^2x=\frac 23\ or −\frac 72\) (not cosider)
\(⇒ sin\ x=±\sqrt {\frac 23}\)

Therefore, \(sin\ x=±\sqrt {\frac 23}\) has \(4\) solutions in the interval \((\frac \pi 4, \frac {7\pi }{4})\).
Top Questions on Trigonometric Functions
- If, \(x∈(0,π)\) satisfies the equation \(6^{1+sinx+sin^2x....}=36\) ,then the value of \(x\) is_____.
- KEAM - 2023
- Mathematics
- Trigonometric Functions
- The value of a(≠0) for which the equation \(\frac{1}{2}(x-2)^2+1=\sin(\frac{a}{x})\) holds is/are
- KEAM - 2023
- Mathematics
- Trigonometric Functions
- Let P(x)=cos2x+sin4x,for any x∈R.Then which of the following options is correct for all x?
- KEAM - 2023
- Mathematics
- Trigonometric Functions
- Minimum value of 5cos(2x) + 5sin(2x)
- BITSAT - 2023
- Mathematics
- Trigonometric Functions
- If $\tan 15^{\circ}+\frac{1}{\tan 75^{\circ}}+\frac{1}{\tan 105^{\circ}}+\tan 195^{\circ}=2 a$, then the value of $\left(a+\frac{1}{a}\right)$ is :
- JEE Main - 2023
- Mathematics
- Trigonometric Functions
Questions Asked in JEE Main exam
- Let \[\vec{a} = \hat{i} + \hat{j} + \hat{k}, \quad \vec{b} = -\hat{i} - 8\hat{j} + 2\hat{k}, \quad \text{and} \quad \vec{c} = 4\hat{i} + c_2\hat{j} + c_3\hat{k} \]be three vectors such that \[\vec{b} \times \vec{a} = \vec{c} \times \vec{a}.\]If the angle between the vector $\vec{c}$ and the vector $3\hat{i} + 4\hat{j} + \hat{k}$ is $\theta$, then the greatest integer less than or equal to $\tan^2 \theta$ is:
- JEE Main - 2024
- Vector Algebra
- 10 mL of gaseous hydrocarbon on combustion gives 40 mL of CO\(_2\)(g) and 50 mL of water vapour. The total number of carbon and hydrogen atoms in the hydrocarbon is ______ .
- JEE Main - 2024
- Hydrocarbons
- If each term of a geometric progression \( a_1, a_2, a_3, \dots \) with \( a_1 = \frac{1}{8} \) and \( a_2 \neq a_1 \), is the arithmetic mean of the next two terms and \( S_n = a_1 + a_2 + \dots + a_n \), then \( S_{20} - S_{18} \) is equal to
- JEE Main - 2024
- Arithmetic Mean
A body of mass 1000 kg is moving horizontally with a velocity of 6 m/s. If 200 kg extra mass is added, the final velocity (in m/s) is:
- JEE Main - 2024
- speed and velocity
- $\textbf{Choose the correct statements about the hydrides of group 15 elements.}$
A. The stability of the hydrides decreases in the order \(\text{NH}_3 > \text{PH}_3 > \text{AsH}_3 > \text{SbH}_3 > \text{BiH}_3\)
B. The reducing ability of the hydrides increases in the order \(\text{NH}_3 < \text{PH}_3 < \text{AsH}_3 < \text{SbH}_3 < \text{BiH}_3\)
C. Among the hydrides, \(\text{NH}_3\) is a strong reducing agent while \(\text{BiH}_3\) is a mild reducing agent.
D. The basicity of the hydrides increases in the order \(\text{NH}_3 < \text{PH}_3 < \text{AsH}_3 < \text{SbH}_3 < \text{BiH}_3\)
Choose the most appropriate from the option given below:- JEE Main - 2024
- p -Block Elements
Concepts Used:
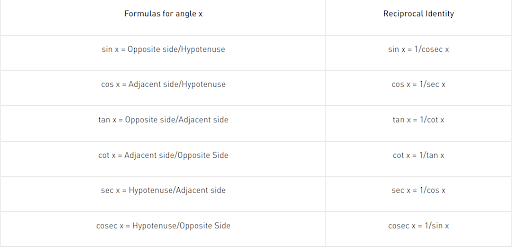
Trigonometric Functions
The relationship between the sides and angles of a right-angle triangle is described by trigonometry functions, sometimes known as circular functions. These trigonometric functions derive the relationship between the angles and sides of a triangle. In trigonometry, there are three primary functions of sine (sin), cosine (cos), tangent (tan). The other three main functions can be derived from the primary functions as cotangent (cot), secant (sec), and cosecant (cosec).
Six Basic Trigonometric Functions:
- Sine Function: The ratio between the length of the opposite side of the triangle to the length of the hypotenuse of the triangle.
sin x = a/h
- Cosine Function: The ratio between the length of the adjacent side of the triangle to the length of the hypotenuse of the triangle.
cos x = b/h
- Tangent Function: The ratio between the length of the opposite side of the triangle to the adjacent side length.
tan x = a/b
Tan x can also be represented as sin x/cos x
- Secant Function: The reciprocal of the cosine function.
sec x = 1/cosx = h/b
- Cosecant Function: The reciprocal of the sine function.
cosec x = 1/sinx = h/a
- Cotangent Function: The reciprocal of the tangent function.
cot x = 1/tan x = b/a
Formulas of Trigonometric Functions: