If \(I_n=\int_{0}^{\frac{\pi}{2}}cos^nxcos\,nxdx\), then \(I_1,I_2,I_3\).....are in
- A.P
- G.P
- H.P
- No such relationship
The Correct Option is D
Solution and Explanation
The given question involves the evaluation of the integral In = ∫[0, π/2] cos^n(x) * cos^n(x) dx, and it introduces a sequence of integrals I1, I2, I3, ... that are not further defined. The claim is that there is no specific relationship between these integrals.
Let's analyze the situation:
Integral Expression: The expression ∫[0, π/2] cos^n(x) * cos^n(x) dx is the integral of the square of the cosine function over the interval [0, π/2].
I1, I2, I3, The question mentions a sequence of integrals, I1, I2, I3, but it doesn't specify the form of these integrals or provide any further information about the sequence.
No Relationship: The claim is that there is no specific relationship between these integrals. This suggests that each integral I1, I2, I3, is independent of the others and doesn't follow any particular pattern or formula based on n.
Given the information provided, we can conclude the following:
I1, I2, I3 are unspecified integrals, and we have no information about their definitions or any pattern connecting them.
The integral In = ∫[0, π/2] cos^n(x) * cos^n(x) dx is a specific integral involving the square of the cosine function over the interval [0, π/2]. This integral depends on the parameter n.
There is no provided relationship or pattern connecting In to the unspecified integrals I1, I2, I3,
Since there is no specified relationship, there is no way to justify or establish any specific relationship between In and the sequence I1, I2, I3,
In summary, the given solution is correct in stating that there is no such relationship between the integrals In and the unspecified sequence I1, I2, I3,The information provided in the question does not allow us to establish any meaningful connection or relationship between these integrals.
The correct answer is option (D): No such relationship
Top Questions on Definite Integral
The value \( 9 \int_{0}^{9} \left\lfloor \frac{10x}{x+1} \right\rfloor \, dx \), where \( \left\lfloor t \right\rfloor \) denotes the greatest integer less than or equal to \( t \), is ________.
- JEE Main - 2024
- Mathematics
- Definite Integral
- The value of the integral \[ \int_{-1}^{2} \log_e \left( x + \sqrt{x^2 + 1} \right) \, dx \] is:
- JEE Main - 2024
- Mathematics
- Definite Integral
- For \( x \in \left( -\frac{\pi}{2}, \frac{\pi}{2} \right) \), if\[y(x) = \int \frac{\csc x + \sin x}{\csc x \sec x + \tan x \sin^2 x} \, dx\]and\[\lim_{x \to -\frac{\pi}{2}} y(x) = 0\]then \( y\left(\frac{\pi}{4}\right) \) is equal to
- JEE Main - 2024
- Mathematics
- Definite Integral
If the value of the integral
\[ \int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \left( \frac{x^2 \cos x}{1 + \pi^x} + \frac{1 + \sin^2 x}{1 + e^{\sin^x 2023}} \right) dx = \frac{\pi}{4} (\pi + a) - 2, \]
then the value of \(a\) is:
- JEE Main - 2024
- Mathematics
- Definite Integral
- Evaluate the limit : \(\lim_{x \to \frac{\pi}{2}} \left( \frac{1}{\left( x - \frac{\pi}{2} \right)^3} \int_{\frac{\pi}{2}}^x \cos \left( \frac{1}{t^3} \right) \, dt \right)\)
- JEE Main - 2024
- Mathematics
- Definite Integral
Questions Asked in WBJEE exam
- Three identical convex lenses each of focal length f are placed in a straight line separated by a distance f from each other. An object is located in \(\frac{f}{2}\) in front of the leftmost lens. Then,

- WBJEE - 2023
- Ray optics and optical instruments
- \(\lim_{x\rightarrow \infty}\){\(x-\sqrt[n]{(x-a_1)(x-a_2)......(x-a_n)}\)} where a1,a2,.....an are positive rational numbers.The limit
- A particle of mass m is projected at velocity at a velocity u, making an angle θ with the horizontal(x-axis). If the angle of projection θ is varied keeping all other parameters same, then magnitude of angular momentum(L) at its maximum height about the point of projection varies with θ as,
- WBJEE - 2023
- System of Particles & Rotational Motion
- If \(\int \frac{dx}{(x+1)(x-2)(x-3)}=\frac{1}{k}log_e\left \{ \frac{|x-3|^3|x+1|}{(x-2)^4}\right \}+c\), then the value of k is
- WBJEE - 2023
- Integrals of Some Particular Functions
- A rectangle ABCD has its side parallel to the line y=2x and vertices A,B,D are on y=1,x=1 and x=-1 respectively. The coordinate of C can be
- WBJEE - 2023
- Straight lines
Concepts Used:
Definite Integral
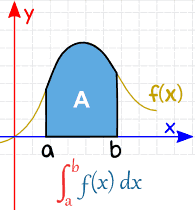
Definite integral is an operation on functions which approximates the sum of the values (of the function) weighted by the length (or measure) of the intervals for which the function takes that value.
Definite integrals - Important Formulae Handbook
A real valued function being evaluated (integrated) over the closed interval [a, b] is written as :
\(\int_{a}^{b}f(x)dx\)
Definite integrals have a lot of applications. Its main application is that it is used to find out the area under the curve of a function, as shown below: